Prüfer theorems

Last updated May 03, 2019

In mathematics, two Prüfer theorems, named after Heinz Prüfer, describe the structure of certain infinite abelian groups. They have been generalized by L. Ya. Kulikov.

Mathematics Field of study concerning quantity, patterns and change

Mathematics includes the study of such topics as quantity, structure, space, and change.

Ernst Paul Heinz Pruefer was a German Jewish mathematician born in Wilhelmshaven. His major contributions were on abelian groups, algebraic numbers, knot theory and Sturm–Liouville theory.

In abstract algebra, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on the order in which they are written. That is, these are the groups that obey the axiom of commutativity. Abelian groups generalize the arithmetic of addition of integers. They are named after early 19th century mathematician Niels Henrik Abel.

Statement

Let A be an abelian group. If A is finitely generated then by the fundamental theorem of finitely generated abelian groups, A is decomposable into a direct sum of cyclic subgroups, which leads to the classification of finitely generated abelian groups up to isomorphism. The structure of general infinite abelian groups can be considerably more complicated and the conclusion needs not to hold, but Prüfer proved that it remains true for periodic groups in two special cases.

In abstract algebra, an abelian group (G, +) is called finitely generated if there exist finitely many elements x₁, ..., x_s in G such that every x in G can be written in the form

The direct sum is an operation from abstract algebra. For example, the direct sum $, where is real coordinate space, is the Cartesian plane, . To see how direct sum is used in abstract algebra, consider a more elementary structure in abstract algebra, the abelian group. The direct sum of two abelian groups and is another abelian group consisting of the ordered pairs where and . To add ordered pairs, we define the sum to be; in other words addition is defined coordinate-wise. A similar process can be used to form the direct sum of any two algebraic structures, such as rings, modules, and vector spaces.$

In group theory, a branch of abstract algebra, a cyclic group or monogenous group is a group that is generated by a single element. That is, it is a set of invertible elements with a single associative binary operation, and it contains an element g such that every other element of the group may be obtained by repeatedly applying the group operation to g or its inverse. Each element can be written as a power of g in multiplicative notation, or as a multiple of g in additive notation. This element g is called a generator of the group.

The first Prüfer theorem states that an abelian group of bounded exponent is isomorphic to a direct sum of cyclic groups. The second Prüfer theorem states that a countable periodic abelian group whose elements have finite height is isomorphic to a direct sum of cyclic groups. Examples show that the assumption that the group be countable cannot be removed.

In mathematics, a countable set is a set with the same cardinality as some subset of the set of natural numbers. A countable set is either a finite set or a countably infinite set. Whether finite or infinite, the elements of a countable set can always be counted one at a time and, although the counting may never finish, every element of the set is associated with a unique natural number.

In mathematics, the height of an element g of an abelian group A is an invariant that captures its divisibility properties: it is the largest natural number N such that the equation Nx = g has a solution x ∈ A, or symbol ∞ if the largest number with this property does not exist. The p-height considers only divisibility properties by the powers of a fixed prime number p. The notion of height admits a refinement so that the p-height becomes an ordinal number. Height plays an important role in Prüfer theorems and also in Ulm's theorem, which describes the classification of certain infinite abelian groups in terms of their Ulm factors or Ulm invariants.

The two Prüfer theorems follow from a general criterion of decomposability of an abelian group into a direct sum of cyclic subgroups due to L. Ya. Kulikov:

An abelian p-group A is isomorphic to a direct sum of cyclic groups if and only if it is a union of a sequence {A_i} of subgroups with the property that the heights of all elements of A_i are bounded by a constant (possibly depending on i).
In mathematical group theory, given a prime number p, a p-group is a group in which each element has a power of p as its order. That is, for each element g of a p-group, there exists a nonnegative integer n such that the product of pⁿ copies of g, and not fewer, is equal to the identity element. The orders of different elements may be different powers of p.
In set theory, the union of a collection of sets is the set of all elements in the collection. It is one of the fundamental operations through which sets can be combined and related to each other.

Related Research Articles

Generating set of a group Subset of a group such that all group elements can be expressed by finitely many group operations on its elements

In abstract algebra, a generating set of a group is a subset such that every element of the group can be expressed as the combination of finitely many elements of the subset and their inverses.

In mathematics, a group G is called the direct sum of two subgroups H₁ and H₂ if

In the theory of abelian groups, the torsion subgroupA_T of an abelian group A is the subgroup of A consisting of all elements that have finite order. An abelian group A is called a torsion group if every element of A has finite order and is called torsion-free if every element of A except the identity is of infinite order.

In abstract algebra, a free abelian group or free Z-module is an abelian group with a basis. Being an abelian group means that it is a set with an addition operation that is associative, commutative, and invertible. A basis is a subset such that every element of the group can be found by adding or subtracting basis elements, and such that every element's expression as a linear combination of basis elements is unique. For instance, the integers under addition form a free abelian group with basis {1}. Addition of integers is commutative, associative, and has subtraction as its inverse operation, each integer is the sum or difference of some number of copies of the number 1, and each integer has a unique representation as an integer multiple of the number 1.

In abstract algebra, a finite group is a group, of which the underlying set contains a finite number of elements.

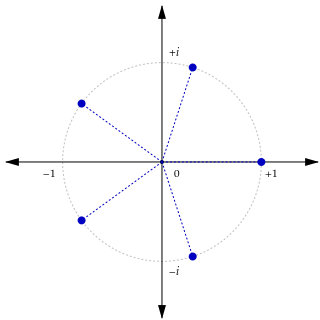
In group theory, a locally cyclic group is a group in which every finitely generated subgroup is cyclic.

In mathematics, the Frattini subgroup Φ( $G$ ) of a group $G$ is the intersection of all maximal subgroups of $G$ . For the case that $G$ has no maximal subgroups, for example the trivial group e or the Prüfer group, it is defined by Φ( $G$ ) = $G$ . It is analogous to the Jacobson radical in the theory of rings, and intuitively can be thought of as the subgroup of "small elements". It is named after Giovanni Frattini, who defined the concept in a paper published in 1885.

In abstract algebra, a module is indecomposable if it is non-zero and cannot be written as a direct sum of two non-zero submodules.

In algebra, a finitely generated group is a group G that has some finite generating set S so that every element of G can be written as the combination of finitely many elements of the finite set S and of inverses of such elements.

In group theory, a branch of mathematics, a torsion group or a periodic group is a group in which each element has finite order. All finite groups are periodic. The concept of a periodic group should not be confused with that of a cyclic group.

In mathematics, specifically in group theory, the Prüfer p-group or the p-quasicyclic group or p^∞-group, Z(p^∞), for a prime number p is the unique p-group in which every element has p different p-th roots.

In mathematics, in the field of group theory, a locally finite group is a type of group that can be studied in ways analogous to a finite group. Sylow subgroups, Carter subgroups, and abelian subgroups of locally finite groups have been studied. The concept is credited to work in the 1930s by Russian mathematician Sergei Chernikov.

In mathematics, especially in the area of algebra studying the theory of abelian groups, a pure subgroup is a generalization of direct summand. It has found many uses in abelian group theory and related areas.

In mathematics, in the field of abstract algebra, the structure theorem for finitely generated modules over a principal ideal domain is a generalization of the fundamental theorem of finitely generated abelian groups and roughly states that finitely generated modules over a principal ideal domain can be uniquely decomposed in much the same way that integers have a prime factorization. The result provides a simple framework to understand various canonical form results for square matrices over fields.

In abstract algebra, a basic subgroup is a subgroup of an abelian group which is a direct sum of cyclic subgroups and satisfies further technical conditions. This notion was introduced by L. Ya. Kulikov and by László Fuchs in an attempt to formulate classification theory of infinite abelian groups that goes beyond the Prüfer theorems. It helps to reduce the classification problem to classification of possible extensions between two well understood classes of abelian groups: direct sums of cyclic groups and divisible groups.

References

László Fuchs (1970), Infinite abelian groups, Vol. I. Pure and Applied Mathematics, Vol. 36. New York–London: Academic Press MR 0255673
Kurosh, A. G. (1960), The theory of groups, New York: Chelsea, MR 0109842

Mathematical Reviews is a journal published by the American Mathematical Society (AMS) that contains brief synopses, and in some cases evaluations, of many articles in mathematics, statistics, and theoretical computer science. The AMS also publishes an associated online bibliographic database called MathSciNet which contains an electronic version of Mathematical Reviews and additionally contains citation information for over 3.5 million items as of 2018.

Alexander Gennadyevich Kurosh was a Soviet mathematician, known for his work in abstract algebra. He is credited with writing the first modern and high-level text on group theory, his The Theory of Groups published in 1944.

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.