In mathematics, more specifically in functional analysis, a Banach space is a complete normed vector space. Thus, a Banach space is a vector space with a metric that allows the computation of vector length and distance between vectors and is complete in the sense that a Cauchy sequence of vectors always converges to a well-defined limit that is within the space.
In quantum mechanics, bra–ket notation, or Dirac notation, is used ubiquitously to denote quantum states. The notation uses angle brackets, and , and a vertical bar , to construct "bras" and "kets".
In mathematics, any vector space has a corresponding dual vector space consisting of all linear forms on together with the vector space structure of pointwise addition and scalar multiplication by constants.
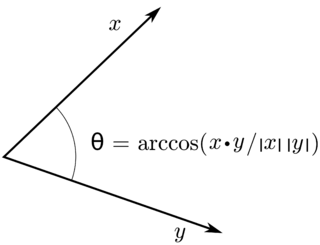
In mathematics, an inner product space is a real vector space or a complex vector space with an operation called an inner product. The inner product of two vectors in the space is a scalar, often denoted with angle brackets such as in . Inner products allow formal definitions of intuitive geometric notions, such as lengths, angles, and orthogonality of vectors. Inner product spaces generalize Euclidean vector spaces, in which the inner product is the dot product or scalar product of Cartesian coordinates. Inner product spaces of infinite dimension are widely used in functional analysis. Inner product spaces over the field of complex numbers are sometimes referred to as unitary spaces. The first usage of the concept of a vector space with an inner product is due to Giuseppe Peano, in 1898.
The Riesz representation theorem, sometimes called the Riesz–Fréchet representation theorem after Frigyes Riesz and Maurice René Fréchet, establishes an important connection between a Hilbert space and its continuous dual space. If the underlying field is the real numbers, the two are isometrically isomorphic; if the underlying field is the complex numbers, the two are isometrically anti-isomorphic. The (anti-) isomorphism is a particular natural isomorphism.
In mathematics, weak topology is an alternative term for certain initial topologies, often on topological vector spaces or spaces of linear operators, for instance on a Hilbert space. The term is most commonly used for the initial topology of a topological vector space with respect to its continuous dual. The remainder of this article will deal with this case, which is one of the concepts of functional analysis.
In mathematics, a self-adjoint operator on an infinite-dimensional complex vector space V with inner product is a linear map A that is its own adjoint. If V is finite-dimensional with a given orthonormal basis, this is equivalent to the condition that the matrix of A is a Hermitian matrix, i.e., equal to its conjugate transpose A∗. By the finite-dimensional spectral theorem, V has an orthonormal basis such that the matrix of A relative to this basis is a diagonal matrix with entries in the real numbers. This article deals with applying generalizations of this concept to operators on Hilbert spaces of arbitrary dimension.
In mathematics, specifically functional analysis, a trace-class operator is a linear operator for which a trace may be defined, such that the trace is a finite number independent of the choice of basis used to compute the trace. This trace of trace-class operators generalizes the trace of matrices studied in linear algebra. All trace-class operators are compact operators.
The Fock space is an algebraic construction used in quantum mechanics to construct the quantum states space of a variable or unknown number of identical particles from a single particle Hilbert space H. It is named after V. A. Fock who first introduced it in his 1932 paper "Konfigurationsraum und zweite Quantelung".
In mathematics as well as physics, a linear operator acting on an inner product space is called positive-semidefinite if, for every , and , where is the domain of . Positive-semidefinite operators are denoted as . The operator is said to be positive-definite, and written , if for all .

In functional analysis, a reproducing kernel Hilbert space (RKHS) is a Hilbert space of functions in which point evaluation is a continuous linear functional. Roughly speaking, this means that if two functions and in the RKHS are close in norm, i.e., is small, then and are also pointwise close, i.e., is small for all . The converse does not need to be true. Informally, this can be shown by looking at the supremum norm: the sequence of functions converges pointwise, but do not converge uniformly i.e. do not converge with respect to the supremum norm.
In mathematics, specifically in operator theory, each linear operator on an inner product space defines a Hermitian adjoint operator on that space according to the rule
In mathematics, a norm is a function from a real or complex vector space to the non-negative real numbers that behaves in certain ways like the distance from the origin: it commutes with scaling, obeys a form of the triangle inequality, and is zero only at the origin. In particular, the Euclidean distance in a Euclidean space is defined by a norm on the associated Euclidean vector space, called the Euclidean norm, the 2-norm, or, sometimes, the magnitude of the vector. This norm can be defined as the square root of the inner product of a vector with itself.
Convex analysis is the branch of mathematics devoted to the study of properties of convex functions and convex sets, often with applications in convex minimization, a subdomain of optimization theory.
Weak formulations are important tools for the analysis of mathematical equations that permit the transfer of concepts of linear algebra to solve problems in other fields such as partial differential equations. In a weak formulation, equations or conditions are no longer required to hold absolutely and has instead weak solutions only with respect to certain "test vectors" or "test functions". In a strong formulation, the solution space is constructed such that these equations or conditions are already fulfilled.
In mathematics, Gårding's inequality is a result that gives a lower bound for the bilinear form induced by a real linear elliptic partial differential operator. The inequality is named after Lars Gårding.

In mathematics, Hilbert spaces allow generalizing the methods of linear algebra and calculus from (finite-dimensional) Euclidean vector spaces to spaces that may be infinite-dimensional. Hilbert spaces arise naturally and frequently in mathematics and physics, typically as function spaces. Formally, a Hilbert space is a vector space equipped with an inner product that defines a distance function for which the space is a complete metric space.
In mathematics, the Dirichlet space on the domain , is the reproducing kernel Hilbert space of holomorphic functions, contained within the Hardy space , for which the Dirichlet integral, defined by
In functional analysis, every C*-algebra is isomorphic to a subalgebra of the C*-algebra of bounded linear operators on some Hilbert space This article describes the spectral theory of closed normal subalgebras of . A subalgebra of is called normal if it is commutative and closed under the operation: for all , we have and that .
This is a glossary for the terminology in a mathematical field of functional analysis.










































