
The Matrix is a 1999 science fiction action film written and directed by the Wachowskis that stars Keanu Reeves, Laurence Fishburne, Carrie-Anne Moss, Hugo Weaving, and Joe Pantoliano and the first installment in the Matrix franchise. It depicts a dystopian future in which humanity is unknowingly trapped inside a simulated reality, the Matrix, created by thought-capable machines to distract humans while using their bodies as an energy source. When computer programmer Thomas Anderson, under the hacker alias "Neo", uncovers the truth, he "is drawn into a rebellion against the machines" along with other people who have been freed from the Matrix.

Dot matrix printing, sometimes called impact matrix printing, is the process of computer printing from a collection of dot matrix data to a device, which can be one of:

In the mathematical theory of knots, the unknot, or trivial knot, is the least knotted of all knots. Intuitively, the unknot is a closed loop of rope without a knot tied into it. To a knot theorist, an unknot is any embedded topological circle in the 3-sphere that is ambient isotopic to a geometrically round circle, the standard unknot.

The Gordian Knot is a legend of Phrygian Gordium associated with Alexander the Great. It is often used as a metaphor for an intractable problem solved easily by finding an approach to the problem that renders the perceived constraints of the problem moot :

In topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life, such as those in shoelaces and rope, a mathematical knot differs in that the ends are joined together so that it cannot be undone, the simplest knot being a ring. In mathematical language, a knot is an embedding of a circle in 3-dimensional Euclidean space, R3. Two mathematical knots are equivalent if one can be transformed into the other via a deformation of R3 upon itself ; these transformations correspond to manipulations of a knotted string that do not involve cutting the string or passing the string through itself.

Eagle Scout is the highest achievement or rank attainable in the Scouts BSA program of the Boy Scouts of America (BSA). Since its inception in 1911, only four percent of Scouts have earned this rank after a lengthy review process. The Eagle Scout rank has been earned by over 2.5 million youth.
In software development, a traceability matrix is a document, usually in the form of a table, used to assist in determining the completeness of a relationship by correlating any two baselined documents using a many-to-many relationship comparison. It is often used with high-level requirements and detailed requirements of the product to the matching parts of high-level design, detailed design, test plan, and test cases.

In the mathematical field of knot theory, a knot polynomial is a knot invariant in the form of a polynomial whose coefficients encode some of the properties of a given knot.
Skein relations are a mathematical tool used to study knots. A central question in the mathematical theory of knots is whether two knot diagrams represent the same knot. One way to answer the question is using knot polynomials, which are invariants of the knot. If two diagrams have different polynomials, they represent different knots. In general, the converse does not hold.

The Piper PA-46 Malibu and Matrix are a family of American light aircraft manufactured by Piper Aircraft of Vero Beach, Florida. The aircraft is powered by a single engine and has the capacity for one pilot and five passengers. Early Malibus were all piston-engined, but a turboprop version, introduced as the Malibu Meridian but now called the M500, is also available. Currently, Piper offers the M350, M500, and M600 in the PA-46 family.
In the mathematical field of knot theory, the Jones polynomial is a knot polynomial discovered by Vaughan Jones in 1984.,. Specifically, it is an invariant of an oriented knot or link which assigns to each oriented knot or link a Laurent polynomial in the variable with integer coefficients.
In mathematics, the Alexander polynomial is a knot invariant which assigns a polynomial with integer coefficients to each knot type. James Waddell Alexander II discovered this, the first knot polynomial, in 1923. In 1969, John Conway showed a version of this polynomial, now called the Alexander–Conway polynomial, could be computed using a skein relation, although its significance was not realized until the discovery of the Jones polynomial in 1984. Soon after Conway's reworking of the Alexander polynomial, it was realized that a similar skein relation was exhibited in Alexander's paper on his polynomial.
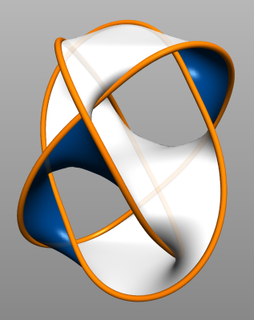
In mathematics, a Seifert surface is a surface whose boundary is a given knot or link.

In knot theory, a branch of mathematics, a knot or link in the 3-dimensional sphere is called fibered or fibred if there is a 1-parameter family of Seifert surfaces for , where the parameter runs through the points of the unit circle , such that if is not equal to then the intersection of and is exactly .
In the mathematical field of knot theory, a chiral knot is a knot that is not equivalent to its mirror image. An oriented knot that is equivalent to its mirror image is an amphichiral knot, also called an achiral knot or amphicheiral knot. The chirality of a knot is a knot invariant. A knot's chirality can be further classified depending on whether or not it is invertible.
In the mathematical field of knot theory, the Arf invariant of a knot, named after Cahit Arf, is a knot invariant obtained from a quadratic form associated to a Seifert surface. If F is a Seifert surface of a knot, then the homology group H1(F, Z/2Z) has a quadratic form whose value is the number of full twists mod 2 in a neighborhood of an imbedded circle representing an element of the homology group. The Arf invariant of this quadratic form is the Arf invariant of the knot.
In mathematics, especially in the area of topology known as knot theory, an invertible knot is a knot that can be continuously deformed to itself, but with its orientation reversed. A non-invertible knot is any knot which does not have this property. The invertibility of a knot is a knot invariant. An invertible link is the link equivalent of an invertible knot.

Ever since Sir William Thomson's vortex theory, mathematicians have tried to classify and tabulate all possible knots. As of May 2008, all prime knots up to 16 crossings have been tabulated.











