Dynamic response index

Last updated

The Dynamic Response Index (DRI) is a measure of the likelihood of spinal damage arising from a vertical shock load such as might be encountered in a military environment (i.e., during a mine blast, or in an ejection seat). The DRI is a dimensionless number which is proportional to the maximum spinal compression suffered during the event.

The DRI is derived as the solution to an equation which models the human spine as a lumped single-degree-of-freedom spring-shock absorber system. The model uses an ordinary linear second-order differential equation with constant coefficients with spinal compression as the variable. The forcing function in the equation is the accelerative shock load delivered to the pelvis by the event. The equation is given below: [1]

Differential equation Mathematical equation that contains derivatives of an unknown function

A differential equation is a mathematical equation that relates some function with its derivatives. In applications, the functions generally represent physical quantities, the derivatives represent their rates of change, and the differential equation defines a relationship between the two. Because such relations are extremely common, differential equations play a prominent role in many disciplines including engineering, physics, economics, and biology.

In this equation, X denotes the spinal compression, and d2z/dt2 denotes the time-dependent shock acceleration (the input). The coefficients ω and ζ are the lumped spinal frequency and damping, ω = 52.90 radians/second, ζ = 0.224.

The DRI is defined in terms of the maximum spinal compression (Xmax) calculated from the differential equation, DRI = (ω2/G)·Xmax, where G is the acceleration of gravity (9.806 m/s2).

The DRI measure was derived from research on cadavers, as well as from “willing volunteers” (essentially, aviators who activated the ejection seat mechanism). [2] [3]

The limiting DRI value according to NATO STANAG 4569 [4] [5] is 17.7 with a 10% chance of serious injury. This corresponds to a maximum spinal compression of about 62 mm.

NATO AEP-55 STANAG 4569 is a NATO Standardization Agreement covering the standards for the "Protection Levels for Occupants of Logistic and Light Armored Vehicles".

A simple rule of thumb for short duration shocks (much less than about a quarter of a cycle, which means much less than about 30 ms) is DRI = 3.96·ΔV, where ΔV is the total impulse delivered by the shock in units of meters per second. ΔV is simply the integral under the shock acceleration curve, equivalent to the “liftoff” velocity of the object undergoing shock loading. For a constant impulse, the DRI decreases as the duration of the pulse increases beyond about 30 ms.

See also

Back injury damage, wear, or trauma to the bones, muscles, or other tissues of the back

Back injuries result from damage, wear, or trauma to the bones, muscles, or other tissues of the back. Common back injuries include sprains and strains, herniated discs, and fractured vertebrae. The lumbar spine is often the site of back pain. The area is susceptible because of its flexibility and the amount of body weight it regularly bears. It is estimated that low-back pain may affect as much as 80 to 90 percent of the general population in the United States.

Crashworthiness is the ability of a structure to protect its occupants during an impact. This is commonly tested when investigating the safety of aircraft and vehicles. Depending on the nature of the impact and the vehicle involved, different criteria are used to determine the crashworthiness of the structure. Crashworthiness may be assessed either prospectively, using computer models or experiments, or retrospectively by analyzing crash outcomes. Several criteria are used to assess crashworthiness prospectively, including the deformation patterns of the vehicle structure, the acceleration experienced by the vehicle during an impact, and the probability of injury predicted by human body models. Injury probability is defined using criteria, which are mechanical parameters that correlate with injury risk. A common injury criterion is the Head impact criterion (HIC). Crashworthiness is assessed retrospectively by analyzing injury risk in real-world crashes, often using regression or other statistical techniques to control for the myriad of confounders that are present in crashes.

Ejection seat system designed to rescue the other crew of an aircraft in an emergency

In aircraft, an ejection seat or ejector seat is a system designed to rescue the pilot or other crew of an aircraft in an emergency. In most designs, the seat is propelled out of the aircraft by an explosive charge or rocket motor, carrying the pilot with it. The concept of an ejectable escape crew capsule has also been tried. Once clear of the aircraft, the ejection seat deploys a parachute. Ejection seats are common on certain types of military aircraft.

Related Research Articles

Acceleration rate at which the magnitude and/or direction of velocity changes with time

In physics, acceleration is the rate of change of velocity of an object with respect to time. An object's acceleration is the net result of all forces acting on the object, as described by Newton's Second Law. The SI unit for acceleration is metre per second squared (m⋅s−2). Accelerations are vector quantities and add according to the parallelogram law. The vector of the net force acting on a body has the same direction as the vector of the body's acceleration, and its magnitude is proportional to the magnitude of the acceleration, with the object's mass as proportionality constant.

In classical mechanics, a harmonic oscillator is a system that, when displaced from its equilibrium position, experiences a restoring force F proportional to the displacement x:

In mechanics and physics, simple harmonic motion is a special type of periodic motion or oscillation where the restoring force is directly proportional to the displacement and acts in the direction opposite to that of displacement.

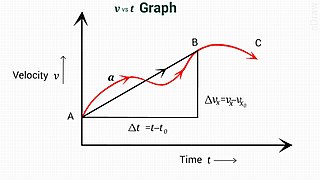
Equations of motion equations of motion related variables

In physics, equations of motion are equations that describe the behavior of a physical system in terms of its motion as a function of time. More specifically, the equations of motion describe the behaviour of a physical system as a set of mathematical functions in terms of dynamic variables: normally spatial coordinates and time are used, but others are also possible, such as momentum components and time. The most general choice are generalized coordinates which can be any convenient variables characteristic of the physical system. The functions are defined in a Euclidean space in classical mechanics, but are replaced by curved spaces in relativity. If the dynamics of a system is known, the equations are the solutions for the differential equations describing the motion of the dynamics.

Kinematics is a branch of classical mechanics that describes the motion of points, bodies (objects), and systems of bodies without considering the forces that cause them to move. Kinematics, as a field of study, is often referred to as the "geometry of motion" and is occasionally seen as a branch of mathematics. A kinematics problem begins by describing the geometry of the system and declaring the initial conditions of any known values of position, velocity and/or acceleration of points within the system. Then, using arguments from geometry, the position, velocity and acceleration of any unknown parts of the system can be determined. The study of how forces act on bodies falls within kinetics, not kinematics. For further details, see analytical dynamics.

Work (physics) process or amount (and direction) of energy transfer to an object via the application of forces on it through a displacement

Work is the product of force and displacement. In physics, a force is said to do work if, when acting, there is a movement of the point of application in the direction of the force.

The vorticity equation of fluid dynamics describes evolution of the vorticity ω of a particle of a fluid as it moves with its flow, that is, the local rotation of the fluid . The equation is:

Fictitious force A force on objects moving within a reference frame that rotates with respect to an inertial frame.

A fictitious force is a force that appears to act on a mass whose motion is described using a non-inertial frame of reference, such as an accelerating or rotating reference frame. An example is seen in a passenger vehicle that is accelerating in the forward direction - passengers perceive that they are acted upon by a force in the rearward direction pushing them back into their seats. An example in a rotating reference frame is the force that appears to push objects outwards towards the rim of a centrifuge. These apparent forces are examples of fictitious forces.

Nondimensionalization is the partial or full removal of units from an equation involving physical quantities by a suitable substitution of variables. This technique can simplify and parameterize problems where measured units are involved. It is closely related to dimensional analysis. In some physical systems, the term scaling is used interchangeably with nondimensionalization, in order to suggest that certain quantities are better measured relative to some appropriate unit. These units refer to quantities intrinsic to the system, rather than units such as SI units. Nondimensionalization is not the same as converting extensive quantities in an equation to intensive quantities, since the latter procedure results in variables that still carry units.

In a compressible sound transmission medium - mainly air - air particles get an accelerated motion: the particle acceleration or sound acceleration with the symbol a in metre/second2. In acoustics or physics, acceleration is defined as the rate of change of velocity. It is thus a vector quantity with dimension length/time2. In SI units, this is m/s2.

Damping is an influence within or upon an oscillatory system that has the effect of reducing, restricting or preventing its oscillations. In physical systems, damping is produced by processes that dissipate the energy stored in the oscillation. Examples include viscous drag in mechanical systems, resistance in electronic oscillators, and absorption and scattering of light in optical oscillators. Damping not based on energy loss can be important in other oscillating systems such as those that occur in biological systems and bikes.

The Mason–Weaver equation describes the sedimentation and diffusion of solutes under a uniform force, usually a gravitational field. Assuming that the gravitational field is aligned in the z direction, the Mason–Weaver equation may be written

In theory of vibrations, Duhamel's integral is a way of calculating the response of linear systems and structures to arbitrary time-varying external perturbation.

The motion of a non-offset piston connected to a crank through a connecting rod, can be expressed through several mathematical equations. This article shows how these motion equations are derived, and shows an example graph.

Potential vorticity (PV) is seen as one of the important theoretical successes of modern meteorology. It is a simplified approach for understanding fluid motions in a rotating system such as the Earth's atmosphere and ocean. Its development traces back to the circulation theorem by Bjerknes in 1898, which is a specialized form of Kelvin's circulation theorem. Starting from Hoskins et al., 1985, PV has been more commonly used in operational weather diagnosis such as tracing dynamics of air parcels and inverting for the full flow field. Even after detailed numerical weather forecasts on finer scales were made possible by increases in computational power, the PV view is still used in academia and routine weather forecasts, shedding light on the synoptic scale features for forecasters and researchers.

In classical mechanics, Appell's equation of motion is an alternative general formulation of classical mechanics described by Paul Émile Appell in 1900 and Josiah Willard Gibbs in 1879

Mild-slope equation The combined effects of diffraction and refraction for water waves propagating over variable depth and with lateral boundaries

In fluid dynamics, the mild-slope equation describes the combined effects of diffraction and refraction for water waves propagating over bathymetry and due to lateral boundaries—like breakwaters and coastlines. It is an approximate model, deriving its name from being originally developed for wave propagation over mild slopes of the sea floor. The mild-slope equation is often used in coastal engineering to compute the wave-field changes near harbours and coasts.

RLC circuit Resistor Inductor Capacitor Circuit

An RLC circuit is an electrical circuit consisting of a resistor (R), an inductor (L), and a capacitor (C), connected in series or in parallel. The name of the circuit is derived from the letters that are used to denote the constituent components of this circuit, where the sequence of the components may vary from RLC.

While geostrophic motion refers to the wind that would result from an exact balance between the Coriolis force and horizontal pressure-gradient forces, quasi-geostrophic (QG) motion refers to flows where the Coriolis force and pressure gradient forces are almost in balance, but with inertia also having an effect.

Accelerations in special relativity (SR) follow, as in Newtonian Mechanics, by differentiation of velocity with respect to time. Because of the Lorentz transformation and time dilation, the concepts of time and distance become more complex, which also leads to more complex definitions of "acceleration". SR as the theory of flat Minkowski spacetime remains valid in the presence of accelerations, because general relativity (GR) is only required when there is curvature of spacetime caused by the energy-momentum tensor. However, since the amount of spacetime curvature is not particularly high on Earth or its vicinity, SR remains valid for most practical purposes, such as experiments in particle accelerators.

References

  1. ↑ MIL-DTL-9479E, DETAIL SPECIFICATION SEAT SYSTEM, UPWARD EJECTION, AIRCRAFT, GENERAL SPECIFICATION FOR, 17 September 1999.
  2. ↑ Aircraft Crash Survival Design Guide, Richard E. Zimmermann, Norman A. Merritt, U.S. Army Aviation Research and Technology Activity (USAAVSCOM TR 89-D-22A), December 1989. E.L. Stech, P.R. Payne, Dynamic Models of the Human Body, Aerospace Medical Research Laboratory (AMRL Technical Report 66-157), November 1969.
  3. ↑ J.W. Brinkley, J.T. Shaffer, Dynamic Simulation Techniques for the Design of Escape Systems: Current Applications and Future Air Force Requirements, Aerospace Medical Research Laboratory (AMRL Technical Report 71-292), December 1971.
  4. ↑ AEP-55, Volume 2, Procedures for Evaluating the Protection Level of Logistics and Light Armored Vehicles, NATO Standardization Organization.
  5. ↑ NATO Stanag 4569, Protection Levels for Occupants of Logistics and Light Armored Vehicles, NATO Standardization Organization.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.