Four square is a ball game.
Contents
Four square may also refer to:
Four square is a ball game.
Four square may also refer to:
In number theory, Waring's problem asks whether each natural number k has an associated positive integer s such that every natural number is the sum of at most s natural numbers raised to the power k. For example, every natural number is the sum of at most 4 squares, 9 cubes, or 19 fourth powers. Waring's problem was proposed in 1770 by Edward Waring, after whom it is named. Its affirmative answer, known as the Hilbert–Waring theorem, was provided by Hilbert in 1909. Waring's problem has its own Mathematics Subject Classification, 11P05, "Waring's problem and variants".
Pinball games are a family of games in which a ball is propelled into a specially designed table where it bounces off various obstacles, scoring points either en route or when it comes to rest. Historically the board was studded with nails called 'pins' and had hollows or pockets which scored points if the ball came to rest in them. Today, pinball is most commonly an arcade game in which the ball is fired into a specially designed cabinet known as a pinball machine, hitting various lights, bumpers, ramps, and other targets depending on its design.
Hilbert's tenth problem is the tenth on the list of mathematical problems that the German mathematician David Hilbert posed in 1900. It is the challenge to provide a general algorithm that, for any given Diophantine equation, can decide whether the equation has a solution with all unknowns taking integer values.
21 (twenty-one) is the natural number following 20 and preceding 22.
The works of the 17th-century mathematician Pierre de Fermat engendered many theorems. Fermat's theorem may refer to one of the following theorems:
33 (thirty-three) is the natural number following 32 and preceding 34.
Lagrange's four-square theorem, also known as Bachet's conjecture, states that every nonnegative integer can be represented as a sum of four non-negative integer squares. That is, the squares form an additive basis of order four. where the four numbers are integers. For illustration, 3, 31, and 310 can be represented as the sum of four squares as follows:
25 (twenty-five) is the natural number following 24 and preceding 26.
100 or one hundred is the natural number following 99 and preceding 101.
In arithmetic and algebra, the fourth power of a number n is the result of multiplying four instances of n together. So:
Klaus Friedrich Roth was a German-born British mathematician who won the Fields Medal for proving Roth's theorem on the Diophantine approximation of algebraic numbers. He was also a winner of the De Morgan Medal and the Sylvester Medal, and a Fellow of the Royal Society.
In additive number theory, the Fermat polygonal number theorem states that every positive integer is a sum of at most nn-gonal numbers. That is, every positive integer can be written as the sum of three or fewer triangular numbers, and as the sum of four or fewer square numbers, and as the sum of five or fewer pentagonal numbers, and so on. That is, the n-gonal numbers form an additive basis of order n.
167 is the natural number following 166 and preceding 168.
In additive number theory, Fermat's theorem on sums of two squares states that an odd prime p can be expressed as:
In mathematics, Lagrange's theorem usually refers to any of the following theorems, attributed to Joseph Louis Lagrange:
In mathematics and statistics, sums of powers occur in a number of contexts:
In number theory, Jacobi's four-square theorem gives a formula for the number of ways that a given positive integer n can be represented as the sum of four squares.
In mathematics, statistics and elsewhere, sums of squares occur in a number of contexts:
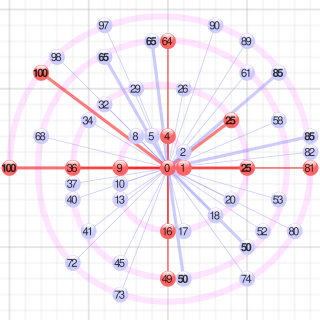
In number theory, the sum of two squares theorem relates the prime decomposition of any integer n > 1 to whether it can be written as a sum of two squares, such that n = a2 + b2 for some integers a, b.
An integer greater than one can be written as a sum of two squares if and only if its prime decomposition contains no factor pk, where prime and k is odd.