| Look up hyperspace in Wiktionary, the free dictionary. |
Hyperspace is a faster-than-light method of traveling used in science fiction.
Contents
Hyperspace or HyperSpace may also refer to:
- the hypertextual or architectural aspect of the Internet
| Look up hyperspace in Wiktionary, the free dictionary. |
Hyperspace is a faster-than-light method of traveling used in science fiction.
Hyperspace or HyperSpace may also refer to:
| This disambiguation page lists articles associated with the title Hyperspace. If an internal link led you here, you may wish to change the link to point directly to the intended article. |

In physics and mathematics, the dimension of a mathematical space is informally defined as the minimum number of coordinates needed to specify any point within it. Thus a line has a dimension of one because only one coordinate is needed to specify a point on it – for example, the point at 5 on a number line. A surface such as a plane or the surface of a cylinder or sphere has a dimension of two because two coordinates are needed to specify a point on it – for example, both a latitude and longitude are required to locate a point on the surface of a sphere. The inside of a cube, a cylinder or a sphere is three-dimensional because three coordinates are needed to locate a point within these spaces.
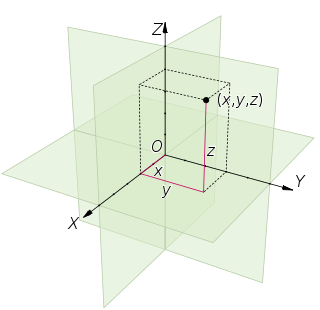
Euclidean space is the fundamental space of classical geometry. Originally it was the three-dimensional space of Euclidean geometry, but in modern mathematics there are Euclidean spaces of any nonnegative integer dimension, including the three-dimensional space and the Euclidean plane. It was introduced by the Ancient Greek mathematician Euclid of Alexandria, and the qualifier Euclidean is used to distinguish it from other spaces that were later discovered in physics and modern mathematics.

In mathematics, topology is concerned with the properties of a geometric object that are preserved under continuous deformations, such as stretching, twisting, crumpling and bending, but not tearing or gluing.

In geometry, the tesseract is the four-dimensional analogue of the cube; the tesseract is to the cube as the cube is to the square. Just as the surface of the cube consists of six square faces, the hypersurface of the tesseract consists of eight cubical cells. The tesseract is one of the six convex regular 4-polytopes.

Algebraic topology is a branch of mathematics that uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classify up to homotopy equivalence.

In geometry, a hyperplane is a subspace whose dimension is one less than that of its ambient space. If a space is 3-dimensional then its hyperplanes are the 2-dimensional planes, while if the space is 2-dimensional, its hyperplanes are the 1-dimensional lines. This notion can be used in any general space in which the concept of the dimension of a subspace is defined.

In mathematics, a discrete subgroup of a topological group G is a subgroup H such that there is an open cover of G in which every open subset contains exactly one element of H; in other words, the subspace topology of H in G is the discrete topology. For example, the integers, Z, form a discrete subgroup of the reals, R, but the rational numbers, Q, do not. A discrete group is a topological group G equipped with the discrete topology.

In mathematics, low-dimensional topology is the branch of topology that studies manifolds, or more generally topological spaces, of four or fewer dimensions. Representative topics are the structure theory of 3-manifolds and 4-manifolds, knot theory, and braid groups. This can be regarded as a part of geometric topology. It may also be used to refer to the study of topological spaces of dimension 1, though this is more typically considered part of continuum theory.

In mathematics, a 3-manifold is a space that locally looks like Euclidean 3-dimensional space. A 3-manifold can be thought of as a possible shape of the universe. Just as a sphere looks like a plane to a small enough observer, all 3-manifolds look like our universe does to a small enough observer. This is made more precise in the definition below.
Hyperspace is a notion from science fiction of a superluminal method of travel. It is typically described as an alternative "sub-region" of space co-existing with our own universe which may be entered using an energy field or other device. In most fiction, hyperspace is described as a physical place that can be entered and exited. Once in hyperspace, the laws of general and special relativity do not behave in the same way when compared to normal outer space, allowing travelers though hyperspace to go great distances without being physically present in normal space and taking less time, measured from normal outer space, to travel said distance. "Through hyper-space, that unimagineable region that was neither space nor time, matter nor energy, something nor nothing, one could traverse the length of the Galaxy in the interval between two neighboring instants of time." Hyperspace is a part of the universe where time can be traveled just like normal space's distance. This allows faster-than-light travel which is necessary to have practical outer space travel.

In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, each point of an n-dimensional manifold has a neighborhood that is homeomorphic to the Euclidean space of dimension n. In this more precise terminology, a manifold is referred to as an n-manifold.

In topology, a branch of mathematics, a topological manifold is a topological space which locally resembles real n-dimensional space in a sense defined below. Topological manifolds form an important class of topological spaces with applications throughout mathematics. All manifolds are topological manifolds by definition, but many manifolds may be equipped with additional structure. Every manifold has an "underlying" topological manifold, gotten by simply "forgetting" any additional structure the manifold has.
The dimension of a space or object is informally defined as the minimum number of coordinates needed to specify any point within it.

In mathematics, a space is a set with some added structure.

In geometry, a honeycomb is a space filling or close packing of polyhedral or higher-dimensional cells, so that there are no gaps. It is an example of the more general mathematical tiling or tessellation in any number of dimensions. Its dimension can be clarified as n-honeycomb for a honeycomb of n-dimensional space.
In topological graph theory, an embedding of a graph on a surface is a representation of on in which points of are associated with vertices and simple arcs are associated with edges in such a way that:

Parallel Worlds: A Journey Through Creation, Higher Dimensions, and the Future of the Cosmos is a popular science book by Michio Kaku first published in 2004.

Geometry is a branch of mathematics concerned with questions of shape, size, relative position of figures, and the properties of space. A mathematician who works in the field of geometry is called a geometer.
In mathematics and physics, a vector is an element of a vector space.
The idea of a fourth dimension has been a factor in the evolution of modern art, but use of concepts relating to higher dimensions has been little discussed by academics in the literary world. From the late 1800s onwards, many writers began to make use of possibilities opened up by the exploration of such concepts as hypercubes and non-Euclidean geometry. While many writers took the fourth dimension to be one of time, others preferred to think of it in spatial terms, and some associated the new mathematics with wider changes in modern culture.