In mathematics, especially in order theory, the cofinality cf(A) of a partially ordered set A is the least of the cardinalities of the cofinal subsets of A.
In the mathematical discipline of set theory, 0# is the set of true formulae about indiscernibles and order-indiscernibles in the Gödel constructible universe. It is often encoded as a subset of the integers, or as a subset of the hereditarily finite sets, or as a real number. Its existence is unprovable in ZFC, the standard form of axiomatic set theory, but follows from a suitable large cardinal axiom. It was first introduced as a set of formulae in Silver's 1966 thesis, later published as Silver (1971), where it was denoted by Σ, and rediscovered by Solovay, who considered it as a subset of the natural numbers and introduced the notation O#.
In mathematics, particularly in set theory, the aleph numbers are a sequence of numbers used to represent the cardinality of infinite sets that can be well-ordered. They were introduced by the mathematician Georg Cantor and are named after the symbol he used to denote them, the Hebrew letter aleph.
In the foundations of mathematics, a covering lemma is used to prove that the non-existence of certain large cardinals leads to the existence of a canonical inner model, called the core model, that is, in a sense, maximal and approximates the structure of the von Neumann universe V. A covering lemma asserts that under some particular anti-large cardinal assumption, the core model exists and is maximal in a sense that depends on the chosen large cardinal. The first such result was proved by Ronald Jensen for the constructible universe assuming 0# does not exist, which is now known as Jensen's covering theorem.
In mathematics, limit cardinals are certain cardinal numbers. A cardinal number λ is a weak limit cardinal if λ is neither a successor cardinal nor zero. This means that one cannot "reach" λ from another cardinal by repeated successor operations. These cardinals are sometimes called simply "limit cardinals" when the context is clear.
In set theory, a regular cardinal is a cardinal number that is equal to its own cofinality. More explicitly, this means that is a regular cardinal if and only if every unbounded subset has cardinality . Infinite well-ordered cardinals that are not regular are called singular cardinals. Finite cardinal numbers are typically not called regular or singular.
PCF theory is the name of a mathematical theory, introduced by Saharon Shelah (1978), that deals with the cofinality of the ultraproducts of ordered sets. It gives strong upper bounds on the cardinalities of power sets of singular cardinals, and has many more applications as well. The abbreviation "PCF" stands for "possible cofinalities".
In mathematics, specifically set theory and model theory, a stationary set is a set that is not too small in the sense that it intersects all club sets and is analogous to a set of non-zero measure in measure theory. There are at least three closely related notions of stationary set, depending on whether one is looking at subsets of an ordinal, or subsets of something of given cardinality, or a powerset.
In model theory, a branch of mathematical logic, the spectrum of a theory is given by the number of isomorphism classes of models in various cardinalities. More precisely, for any complete theory T in a language we write I(T, κ) for the number of models of T (up to isomorphism) of cardinality κ. The spectrum problem is to describe the possible behaviors of I(T, κ) as a function of κ. It has been almost completely solved for the case of a countable theory T.
In set theory, an ordinal number α is an admissible ordinal if Lα is an admissible set ; in other words, α is admissible when α is a limit ordinal and Lα ⊧ Σ0-collection. The term was coined by Richard Platek in 1966.
In the mathematical field of set theory, the proper forcing axiom (PFA) is a significant strengthening of Martin's axiom, where forcings with the countable chain condition (ccc) are replaced by proper forcings.
In set theory, an Aronszajn tree is a tree of uncountable height with no uncountable branches and no uncountable levels. For example, every Suslin tree is an Aronszajn tree. More generally, for a cardinal κ, a κ-Aronszajn tree is a tree of height κ in which all levels have size less than κ and all branches have height less than κ. They are named for Nachman Aronszajn, who constructed an Aronszajn tree in 1934; his construction was described by Kurepa (1935).
In mathematics, infinitary combinatorics, or combinatorial set theory, is an extension of ideas in combinatorics to infinite sets. Some of the things studied include continuous graphs and trees, extensions of Ramsey's theorem, and Martin's axiom. Recent developments concern combinatorics of the continuum and combinatorics on successors of singular cardinals.

Menachem Magidor is an Israeli mathematician who specializes in mathematical logic, in particular set theory. He served as president of the Hebrew University of Jerusalem, was president of the Association for Symbolic Logic from 1996 to 1998 and as president of the Division for Logic, Methodology and Philosophy of Science and Technology of the International Union for History and Philosophy of Science (DLMPST/IUHPS) from 2016 to 2019. In 2016 he was elected an honorary foreign member of the American Academy of Arts and Sciences. In 2018 he received the Solomon Bublick Award.
In the mathematical theory of infinite graphs, the Erdős–Dushnik–Miller theorem is a form of Ramsey's theorem stating that every infinite graph contains either a countably infinite independent set, or a clique with the same cardinality as the whole graph.
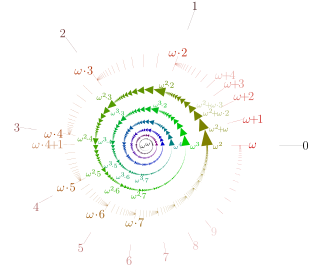
In set theory, an ordinal number, or ordinal, is a generalization of ordinal numerals aimed to extend enumeration to infinite sets.
In model theory, a discipline within mathematical logic, an abstract elementary class, or AEC for short, is a class of models with a partial order similar to the relation of an elementary substructure of an elementary class in first-order model theory. They were introduced by Saharon Shelah.
In the mathematical discipline of set theory, a cardinal characteristic of the continuum is an infinite cardinal number that may consistently lie strictly between , and the cardinality of the continuum, that is, the cardinality of the set of all real numbers. The latter cardinal is denoted or . A variety of such cardinal characteristics arise naturally, and much work has been done in determining what relations between them are provable, and constructing models of set theory for various consistent configurations of them.