Isaac Newton's rotating bucket argument was designed to demonstrate that true rotational motion cannot be defined as the relative rotation of the body with respect to the immediately surrounding bodies. It is one of five arguments from the "properties, causes, and effects" of "true motion and rest" that support his contention that, in general, true motion and rest cannot be defined as special instances of motion or rest relative to other bodies, but instead can be defined only by reference to absolute space. Alternatively, these experiments provide an operational definition of what is meant by "absolute rotation", and do not pretend to address the question of "rotation relative to what?" General relativity dispenses with absolute space and with physics whose cause is external to the system, with the concept of geodesics of spacetime.

A sphere is a perfectly round geometrical object in three-dimensional space that is the surface of a completely round ball.

A rotation is a circular movement of an object around a center of rotation. A three-dimensional object can always be rotated around an infinite number of imaginary lines called rotation axes. If the axis passes through the body's center of mass, the body is said to rotate upon itself, or spin. A rotation about an external point, e.g. the Earth about the Sun, is called a revolution or orbital revolution, typically when it is produced by gravity. The axis is called a pole.

A cam is a rotating or sliding piece in a mechanical linkage used especially in transforming rotary motion into linear motion. It is often a part of a rotating wheel or shaft that strikes a lever at one or more points on its circular path. The cam can be a simple tooth, as is used to deliver pulses of power to a steam hammer, for example, or an eccentric disc or other shape that produces a smooth reciprocating motion in the follower, which is a lever making contact with the cam.
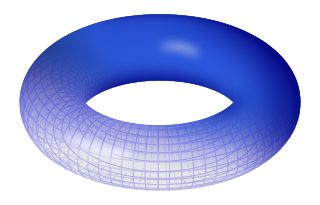
In geometry, a torus is a surface of revolution generated by revolving a circle in three-dimensional space about an axis that is coplanar with the circle.

A lathe is a machine tool that rotates a workpiece about an axis of rotation to perform various operations such as cutting, sanding, knurling, drilling, deformation, facing, and turning, with tools that are applied to the workpiece to create an object with symmetry about that axis.
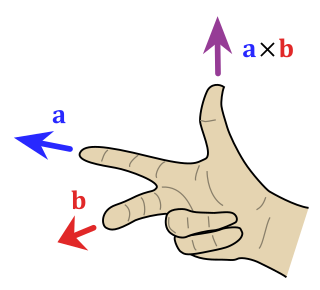
In mathematics and physics, the right-hand rule is a common mnemonic for understanding orientation of axes in three-dimensional space.

The Magnus effect is an observable phenomenon that is commonly associated with a spinning object moving through the air or a fluid. The path of the spinning object is deflected in a manner that is not present when the object is not spinning. The deflection can be explained by the difference in pressure of the fluid on opposite sides of the spinning object.

A surface of revolution is a surface in Euclidean space created by rotating a curve around an axis of rotation.

Gimbal lock is the loss of one degree of freedom in a three-dimensional, three-gimbal mechanism that occurs when the axes of two of the three gimbals are driven into a parallel configuration, "locking" the system into rotation in a degenerate two-dimensional space.
In mathematics, the special orthogonal group in three dimensions, otherwise known as the rotation group SO(3), is a naturally occurring example of a manifold. The various charts on SO(3) set up rival coordinate systems: in this case there cannot be said to be a preferred set of parameters describing a rotation. There are three degrees of freedom, so that the dimension of SO(3) is three. In numerous applications one or other coordinate system is used, and the question arises how to convert from a given system to another.

In physics, a rigid body is a solid body in which deformation is zero or so small it can be neglected. The distance between any two given points on a rigid body remains constant in time regardless of external forces exerted on it. A rigid body is usually considered as a continuous distribution of mass.

In geometry, Villarceau circles are a pair of circles produced by cutting a torus obliquely through the center at a special angle. Given an arbitrary point on a torus, four circles can be drawn through it. One is in the plane parallel to the equatorial plane of the torus. Another is perpendicular to it. The other two are Villarceau circles. They are named after the French astronomer and mathematician Yvon Villarceau (1813–1883). Mannheim (1903) showed that the Villarceau circles meet all of the parallel circular cross-sections of the torus at the same angle, a result that he said a Colonel Schoelcher had presented at a congress in 1891.

Turning is a machining process in which a cutting tool, typically a non-rotary tool bit, describes a helix toolpath by moving more or less linearly while the workpiece rotates.

In geometry, circular symmetry is a type of continuous symmetry for a planar object that can be rotated by any arbitrary angle and map onto itself.

In machining, boring is the process of enlarging a hole that has already been drilled by means of a single-point cutting tool, such as in boring a gun barrel or an engine cylinder. Boring is used to achieve greater accuracy of the diameter of a hole, and can be used to cut a tapered hole. Boring can be viewed as the internal-diameter counterpart to turning, which cuts external diameters.

Rotation around a fixed axis or about a fixed axis of revolution or motion with respect to a fixed axis of rotation is a special case of rotational motion. The fixed axis hypothesis excludes the possibility of an axis changing its orientation, and cannot describe such phenomena as wobbling or precession. According to Euler's rotation theorem, simultaneous rotation along a number of stationary axes at the same time is impossible. If two rotations are forced at the same time, a new axis of rotation will appear.
In Gaussian optics, the cardinal points consist of three pairs of points located on the optical axis of a rotationally symmetric, focal, optical system. These are the focal points, the principal points, and the nodal points. For ideal systems, the basic imaging properties such as image size, location, and orientation are completely determined by the locations of the cardinal points; in fact only four points are necessary: the focal points and either the principal or nodal points. The only ideal system that has been achieved in practice is the plane mirror, however the cardinal points are widely used to approximate the behavior of real optical systems. Cardinal points provide a way to analytically simplify a system with many components, allowing the imaging characteristics of the system to be approximately determined with simple calculations.

In Newtonian mechanics, the centrifugal force is an inertial force that appears to act on all objects when viewed in a rotating frame of reference. It is directed away from an axis passing through the coordinate system's origin and parallel to the axis of rotation. If the axis of rotation passes through the coordinate system's origin, the centrifugal force is directed radially outwards from that axis. The concept of centrifugal force can be applied in rotating devices, such as centrifuges, centrifugal pumps, centrifugal governors, and centrifugal clutches, and in centrifugal railways, planetary orbits and banked curves, when they are analyzed in a rotating coordinate system. The term has sometimes also been used for the reactive centrifugal force that may be viewed as a reaction to a centripetal force in some circumstances.

In mathematics, a minimal surface of revolution or minimum surface of revolution is a surface of revolution defined from two points in a half-plane, whose boundary is the axis of revolution of the surface. It is generated by a curve that lies in the half-plane and connects the two points; among all the surfaces that can be generated in this way, it is the one that minimizes the surface area. A basic problem in the calculus of variations is finding the curve between two points that produces this minimal surface of revolution.















