Look up polarity in Wiktionary, the free dictionary.
Polarity may refer to:
Polarity may refer to:
Dual or Duals may refer to:
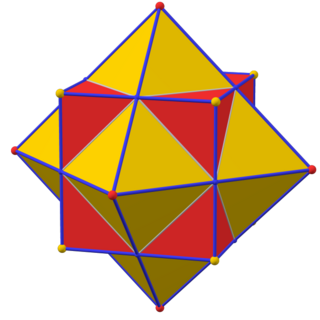
In geometry, every polyhedron is associated with a second dual structure, where the vertices of one correspond to the faces of the other, and the edges between pairs of vertices of one correspond to the edges between pairs of faces of the other. Such dual figures remain combinatorial or abstract polyhedra, but not all can also be constructed as geometric polyhedra. Starting with any given polyhedron, the dual of its dual is the original polyhedron.
PCP may refer to:
Transformation may refer to:
Pole may refer to:
Polar may refer to:
Category, plural categories, may refer to:
Reciprocity may refer to:
Bipolar may refer to:
Duality may refer to:
Negative may refer to:
In chemistry, polarity is a separation of electric charge leading to a molecule or its chemical groups having an electric dipole moment, with a negatively charged end and a positively charged end.
Absolute may refer to:
In geometry, a striking feature of projective planes is the symmetry of the roles played by points and lines in the definitions and theorems, and (plane) duality is the formalization of this concept. There are two approaches to the subject of duality, one through language and the other a more functional approach through special mappings. These are completely equivalent and either treatment has as its starting point the axiomatic version of the geometries under consideration. In the functional approach there is a map between related geometries that is called a duality. Such a map can be constructed in many ways. The concept of plane duality readily extends to space duality and beyond that to duality in any finite-dimensional projective geometry.
Continuity or continuous may refer to:
Polarization or polarisation may refer to:
Double Negative may refer to:
Dualism most commonly refers to:
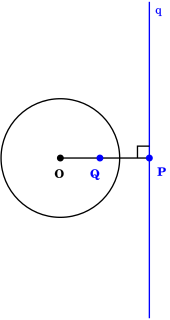
In geometry, a pole and polar are respectively a point and a line that have a unique reciprocal relationship with respect to a given conic section.
In projective geometry, a von Staudt conic is the point set defined by all the absolute points of a polarity that has absolute points. In the real projective plane a von Staudt conic is a conic section in the usual sense. In more general projective planes this is not always the case. Karl Georg Christian von Staudt introduced this definition in Geometrie der Lage (1847) as part of his attempt to remove all metrical concepts from projective geometry.