
In elementary geometry, two geometric objects are perpendicular if their intersection forms right angles at the point of intersection called a foot. The condition of perpendicularity may be represented graphically using the perpendicular symbol, ⟂. Perpendicular intersections can happen between two lines, between a line and a plane, and between two planes.

In geometry, an altitude of a triangle is a line segment through a vertex and perpendicular to a line containing the the side opposite the vertex. This line containing the opposite side is called the extended base of the altitude. The intersection of the extended base and the altitude is called the foot of the altitude. The length of the altitude, often simply called "the altitude", is the distance between the extended base and the vertex. The process of drawing the altitude from the vertex to the foot is known as dropping the altitude at that vertex. It is a special case of orthogonal projection.

In geometry, the incircle or inscribed circle of a triangle is the largest circle that can be contained in the triangle; it touches the three sides. The center of the incircle is a triangle center called the triangle's incenter.

Spherical geometry or spherics is the geometry of the two-dimensional surface of a sphere or the n-dimensional surface of higher dimensional spheres.

In geometry, the Euler line, named after Leonhard Euler, is a line determined from any triangle that is not equilateral. It is a central line of the triangle, and it passes through several important points determined from the triangle, including the orthocenter, the circumcenter, the centroid, the Exeter point and the center of the nine-point circle of the triangle.

In geometry, the incenter of a triangle is a triangle center, a point defined for any triangle in a way that is independent of the triangle's placement or scale. The incenter may be equivalently defined as the point where the internal angle bisectors of the triangle cross, as the point equidistant from the triangle's sides, as the junction point of the medial axis and innermost point of the grassfire transform of the triangle, and as the center point of the inscribed circle of the triangle.
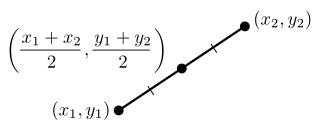
In geometry, the midpoint is the middle point of a line segment. It is equidistant from both endpoints, and it is the centroid both of the segment and of the endpoints. It bisects the segment.
In geometry, a striking feature of projective planes is the symmetry of the roles played by points and lines in the definitions and theorems, and (plane) duality is the formalization of this concept. There are two approaches to the subject of duality, one through language and the other a more functional approach through special mappings. These are completely equivalent and either treatment has as its starting point the axiomatic version of the geometries under consideration. In the functional approach there is a map between related geometries that is called a duality. Such a map can be constructed in many ways. The concept of plane duality readily extends to space duality and beyond that to duality in any finite-dimensional projective geometry.

In geometry, lines in a plane or higher-dimensional space are concurrent if they intersect at a single point. They are in contrast to parallel lines.

In Euclidean plane geometry, Apollonius's problem is to construct circles that are tangent to three given circles in a plane (Figure 1). Apollonius of Perga posed and solved this famous problem in his work Ἐπαφαί ; this work has been lost, but a 4th-century AD report of his results by Pappus of Alexandria has survived. Three given circles generically have eight different circles that are tangent to them (Figure 2), a pair of solutions for each way to divide the three given circles in two subsets.
In geometry, collinearity of a set of points is the property of their lying on a single line. A set of points with this property is said to be collinear. In greater generality, the term has been used for aligned objects, that is, things being "in a line" or "in a row".

In geometry, the GEOS circle is derived from the intersection of four lines that are associated with a generalized triangle: the Euler line, the Soddy line, the orthic axis and the Gergonne line. Note that the Euler line is orthogonal to the orthic axis and that the Soddy line is orthogonal to the Gergonne line.

In geometry, the de Longchamps point of a triangle is a triangle center named after French mathematician Gaston Albert Gohierre de Longchamps. It is the reflection of the orthocenter of the triangle about the circumcenter.
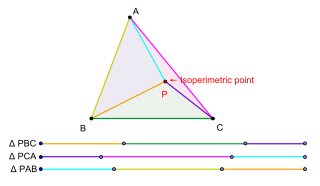
In geometry, the isoperimetric point is a special point associated with a plane triangle. The term was originally introduced by G.R. Veldkamp in a paper published in the American Mathematical Monthly in 1985 to denote a point P in the plane of a triangle ABC having the property that the triangles PBC, PCA and PAB have isoperimeters, that is, having the property that

In geometry, the orthocentroidal circle of a non-equilateral triangle is the circle that has the triangle's orthocenter and centroid at opposite ends of its diameter. This diameter also contains the triangle's nine-point center and is a subset of the Euler line, which also contains the circumcenter outside the orthocentroidal circle.

In geometry, the tangential triangle of a reference triangle is the triangle whose sides are on the tangent lines to the reference triangle's circumcircle at the reference triangle's vertices. Thus the incircle of the tangential triangle coincides with the circumcircle of the reference triangle.
John Frankland Rigby was an English mathematician and academic of the University College of South Wales, Cardiff, when it was part of the University of Wales, and of its successor Cardiff University.
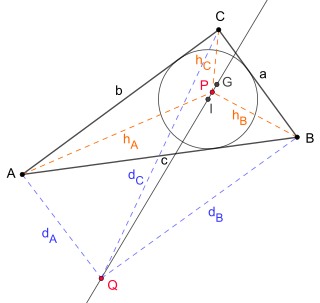
The equal detour point is a triangle center with the Kimberling number X(176). It is characterized by the equal detour property, that is if you travel from any vertex of a triangle to another by taking a detour through some inner point then the additional distance travelled is constant. This means the following equation has to hold:
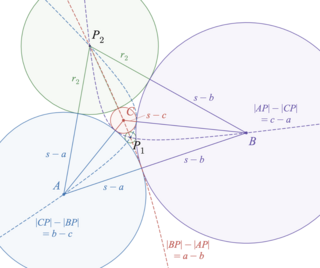
In geometry, the Soddy circles of a triangle are two circles associated with any triangle in the plane. Their centers are the Soddy centers of the triangle. They are all named for Frederick Soddy, who rediscovered Descartes' theorem on the radii of mutually tangent quadruples of circles.



























