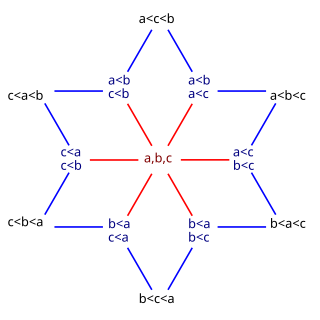
In combinatorics, Sun's curious identity is the following identity involving binomial coefficients, first established by Zhi-Wei Sun in 2002:
In combinatorics, Sun's curious identity is the following identity involving binomial coefficients, first established by Zhi-Wei Sun in 2002:
After Sun's publication of this identity in 2002, five other proofs were obtained by various mathematicians:

In mathematics, the binomial coefficients are the positive integers that occur as coefficients in the binomial theorem. Commonly, a binomial coefficient is indexed by a pair of integers n ≥ k ≥ 0 and is written It is the coefficient of the xk term in the polynomial expansion of the binomial power (1 + x)n; this coefficient can be computed by the multiplicative formula
In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomial. According to the theorem, it is possible to expand the polynomial (x + y)n into a sum involving terms of the form axbyc, where the exponents b and c are nonnegative integers with b + c = n, and the coefficient a of each term is a specific positive integer depending on n and b. For example, for n = 4,
In mathematics, Pascal's triangle is a triangular array of the binomial coefficients arising in probability theory, combinatorics, and algebra. In much of the Western world, it is named after the French mathematician Blaise Pascal, although other mathematicians studied it centuries before him in Persia, India, China, Germany, and Italy.
This article collects together a variety of proofs of Fermat's little theorem, which states that
Hilbert's tenth problem is the tenth on the list of mathematical problems that the German mathematician David Hilbert posed in 1900. It is the challenge to provide a general algorithm that, for any given Diophantine equation, can decide whether the equation has a solution with all unknowns taking integer values.

In combinatorial mathematics, the Catalan numbers are a sequence of natural numbers that occur in various counting problems, often involving recursively defined objects. They are named after the French-Belgian mathematician Eugène Charles Catalan.
Combinatorics is a branch of mathematics concerning the study of finite or countable discrete structures.
In mathematics, the binomial series is a generalization of the polynomial that comes from a binomial formula expression like for a nonnegative integer . Specifically, the binomial series is the MacLaurin series for the function , where and . Explicitly,
In mathematics, the nth Motzkin number is the number of different ways of drawing non-intersecting chords between n points on a circle. The Motzkin numbers are named after Theodore Motzkin and have diverse applications in geometry, combinatorics and number theory.
In combinatorics, Vandermonde's identity is the following identity for binomial coefficients:
In combinatorics, bijective proof is a proof technique for proving that two sets have equally many elements, or that the sets in two combinatorial classes have equal size, by finding a bijective function that maps one set one-to-one onto the other. This technique can be useful as a way of finding a formula for the number of elements of certain sets, by corresponding them with other sets that are easier to count. Additionally, the nature of the bijection itself often provides powerful insights into each or both of the sets.
Sun Zhiwei is a Chinese mathematician, working primarily in number theory, combinatorics, and group theory. He is a professor at Nanjing University.
In number theory and enumerative combinatorics, the ordered Bell numbers or Fubini numbers count the number of weak orderings on a set of elements. Weak orderings arrange their elements into a sequence allowing ties, such as might arise as the outcome of a horse race). Starting from , these numbers are
In number theory, Lucas's theorem expresses the remainder of division of the binomial coefficient by a prime number p in terms of the base p expansions of the integers m and n.
In additive number theory and combinatorics, a restricted sumset has the form
In mathematics, Dixon's identity is any of several different but closely related identities proved by A. C. Dixon, some involving finite sums of products of three binomial coefficients, and some evaluating a hypergeometric sum. These identities famously follow from the MacMahon Master theorem, and can now be routinely proved by computer algorithms.
In mathematics, MacMahon's master theorem (MMT) is a result in enumerative combinatorics and linear algebra. It was discovered by Percy MacMahon and proved in his monograph Combinatory analysis (1916). It is often used to derive binomial identities, most notably Dixon's identity.

In mathematics, the telephone numbers or the involution numbers form a sequence of integers that count the ways n people can be connected by person-to-person telephone calls. These numbers also describe the number of matchings of a complete graph on n vertices, the number of permutations on n elements that are involutions, the sum of absolute values of coefficients of the Hermite polynomials, the number of standard Young tableaux with n cells, and the sum of the degrees of the irreducible representations of the symmetric group. Involution numbers were first studied in 1800 by Heinrich August Rothe, who gave a recurrence equation by which they may be calculated, giving the values
In combinatorial mathematics, cyclic sieving is a phenomenon by which evaluating a generating function for a finite set at roots of unity counts symmetry classes of objects acted on by a cyclic group.