Tannery's theorem

Last updated July 02, 2023

In mathematical analysis, Tannery's theorem gives sufficient conditions for the interchanging of the limit and infinite summation operations. It is named after Jules Tannery.^[1]

Statement

Let $S_{n}=\sum _{k=0}^{\infty }a_{k}(n)$ and suppose that $\lim _{n\to \infty }a_{k}(n)=b_{k}$ . If $|a_{k}(n)|\leq M_{k}$ and $\sum _{k=0}^{\infty }M_{k}<\infty$ , then $\lim _{n\to \infty }S_{n}=\sum _{k=0}^{\infty }b_{k}$ .^[2]^[3]

Proofs

Tannery's theorem follows directly from Lebesgue's dominated convergence theorem applied to the sequence space $\ell ^{1}$ .

An elementary proof can also be given.^[3]

Example

Tannery's theorem can be used to prove that the binomial limit and the infinite series characterizations of the exponential $e^{x}$ are equivalent. Note that

\lim _{n\to \infty }\left(1+{\frac {x}{n}}\right)^{n}=\lim _{n\to \infty }\sum _{k=0}^{n}{n \choose k}{\frac {x^{k}}{n^{k}}}.

Define $a_{k}(n)={n \choose k}{\frac {x^{k}}{n^{k}}}$ . We have that $|a_{k}(n)|\leq {\frac {|x|^{k}}{k!}}$ and that $\sum _{k=0}^{\infty }{\frac {|x|^{k}}{k!}}=e^{|x|}<\infty$ , so Tannery's theorem can be applied and

\lim _{n\to \infty }\sum _{k=0}^{\infty }{n \choose k}{\frac {x^{k}}{n^{k}}}=\sum _{k=0}^{\infty }\lim _{n\to \infty }{n \choose k}{\frac {x^{k}}{n^{k}}}=\sum _{k=0}^{\infty }{\frac {x^{k}}{k!}}=e^{x}.

Related Research Articles

In complex analysis, an entire function, also called an integral function, is a complex-valued function that is holomorphic on the whole complex plane. Typical examples of entire functions are polynomials and the exponential function, and any finite sums, products and compositions of these, such as the trigonometric functions sine and cosine and their hyperbolic counterparts sinh and cosh, as well as derivatives and integrals of entire functions such as the error function. If an entire function $has a root at, then, taking the limit value at, is an entire function. On the other hand, the natural logarithm, the reciprocal function, and the square root are all not entire functions, nor can they be continued analytically to an entire function.$

In mathematics, a series is, roughly speaking, the operation of adding infinitely many quantities, one after the other, to a given starting quantity. The study of series is a major part of calculus and its generalization, mathematical analysis. Series are used in most areas of mathematics, even for studying finite structures through generating functions. In addition to their ubiquity in mathematics, infinite series are also widely used in other quantitative disciplines such as physics, computer science, statistics and finance.

In mathematics, the prime number theorem (PNT) describes the asymptotic distribution of the prime numbers among the positive integers. It formalizes the intuitive idea that primes become less common as they become larger by precisely quantifying the rate at which this occurs. The theorem was proved independently by Jacques Hadamard and Charles Jean de la Vallée Poussin in 1896 using ideas introduced by Bernhard Riemann.

In mathematics, the branch of real analysis studies the behavior of real numbers, sequences and series of real numbers, and real functions. Some particular properties of real-valued sequences and functions that real analysis studies include convergence, limits, continuity, smoothness, differentiability and integrability.

In probability theory, the Borel–Cantelli lemma is a theorem about sequences of events. In general, it is a result in measure theory. It is named after Émile Borel and Francesco Paolo Cantelli, who gave statement to the lemma in the first decades of the 20th century. A related result, sometimes called the second Borel–Cantelli lemma, is a partial converse of the first Borel–Cantelli lemma. The lemma states that, under certain conditions, an event will have probability of either zero or one. Accordingly, it is the best-known of a class of similar theorems, known as zero-one laws. Other examples include Kolmogorov's zero–one law and the Hewitt–Savage zero–one law.

In the mathematical field of analysis, uniform convergence is a mode of convergence of functions stronger than pointwise convergence. A sequence of functions $converges uniformly to a limiting function on a set as the function domain if, given any arbitrarily small positive number, a number can be found such that each of the functions differs from by no more than at every point in . Described in an informal way, if converges to uniformly, then the rate at which approaches is "uniform" throughout its domain in the following sense: in order to show that uniformly falls within a certain distance of, we do not need to know the value of in question — there can be found a single value of independent of, such that choosing will ensure that is within of for all . In contrast, pointwise convergence of to merely guarantees that for any given in advance, we can find such that, for that particular, falls within of whenever .$

In the mathematical field of real analysis, the monotone convergence theorem is any of a number of related theorems proving the convergence of monotonic sequences that are also bounded. Informally, the theorems state that if a sequence is increasing and bounded above by a supremum, then the sequence will converge to the supremum; in the same way, if a sequence is decreasing and is bounded below by an infimum, it will converge to the infimum.

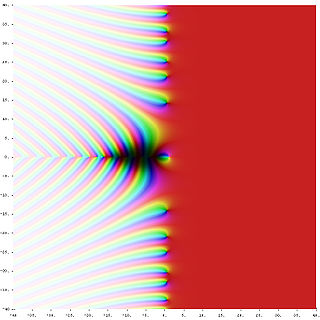
In mathematics, analytic number theory is a branch of number theory that uses methods from mathematical analysis to solve problems about the integers. It is often said to have begun with Peter Gustav Lejeune Dirichlet's 1837 introduction of Dirichlet L-functions to give the first proof of Dirichlet's theorem on arithmetic progressions. It is well known for its results on prime numbers and additive number theory.

In mathematics, the limit of a sequence is the value that the terms of a sequence "tend to", and is often denoted using the $symbol. If such a limit exists, the sequence is called convergent . A sequence that does not converge is said to be divergent . The limit of a sequence is said to be the fundamental notion on which the whole of mathematical analysis ultimately rests.$

In mathematics, the ratio test is a test for the convergence of a series

In mathematics, the integral test for convergence is a method used to test infinite series of monotonous terms for convergence. It was developed by Colin Maclaurin and Augustin-Louis Cauchy and is sometimes known as the Maclaurin–Cauchy test.

In mathematics, nonstandard calculus is the modern application of infinitesimals, in the sense of nonstandard analysis, to infinitesimal calculus. It provides a rigorous justification for some arguments in calculus that were previously considered merely heuristic.

In mathematics, a divergent series is an infinite series that is not convergent, meaning that the infinite sequence of the partial sums of the series does not have a finite limit.

In analytic number theory, Mertens' theorems are three 1874 results related to the density of prime numbers proved by Franz Mertens.

In mathematics, the root test is a criterion for the convergence of an infinite series. It depends on the quantity

In mathematical analysis, the alternating series test is the method used to show that an alternating series is convergent when its terms (1) decrease in absolute value, and (2) approach zero in the limit. The test was used by Gottfried Leibniz and is sometimes known as Leibniz's test, Leibniz's rule, or the Leibniz criterion. The test is only sufficient, not necessary, so some convergent alternating series may fail the first part of the test.

In mathematics, the comparison test, sometimes called the direct comparison test to distinguish it from similar related tests, provides a way of deducing the convergence or divergence of an infinite series or an improper integral. In both cases, the test works by comparing the given series or integral to one whose convergence properties are known.

Euclid's theorem is a fundamental statement in number theory that asserts that there are infinitely many prime numbers. It was first proved by Euclid in his work Elements. There are several proofs of the theorem.

In mathematics, convergence tests are methods of testing for the convergence, conditional convergence, absolute convergence, interval of convergence or divergence of an infinite series $.$

In multivariable calculus, an iterated limit is a limit of a sequence or a limit of a function in the form

References

↑ Loya, Paul (2018). Amazing and Aesthetic Aspects of Analysis. Springer. ISBN 9781493967957.
↑ Ismail, Mourad E. H.; Koelink, Erik, eds. (2005). Theory and Applications of Special Functions: A Volume Dedicated to Mizan Rahman. New York: Springer. p. 448. ISBN 9780387242330.
1 2 Hofbauer, Josef (2002). "A Simple Proof of $1+1/2^{2}+1/3^{2}+\cdots ={\frac {\pi ^{2}}{6}}$ and Related Identities". The American Mathematical Monthly. 109 (2): 196–200. doi:10.2307/2695334. JSTOR 2695334.

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] Loya, Paul (2018). Amazing and Aesthetic Aspects of Analysis. Springer. ISBN 9781493967957.

[2] Ismail, Mourad E. H.; Koelink, Erik, eds. (2005). Theory and Applications of Special Functions: A Volume Dedicated to Mizan Rahman. New York: Springer. p. 448. ISBN 9780387242330.

[:0-3] 1 2 Hofbauer, Josef (2002). "A Simple Proof of $1+1/2^{2}+1/3^{2}+\cdots ={\frac {\pi ^{2}}{6}}$ and Related Identities". The American Mathematical Monthly. 109 (2): 196–200. doi:10.2307/2695334. JSTOR 2695334.

[1]

[2]

[3]