Lute of Pythagoras

Last updated
Lute of Pythagoras Lute of Pythagoras.svg
Lute of Pythagoras

The lute of Pythagoras is a self-similar geometric figure made from a sequence of pentagrams.

Self-similarity

In mathematics, a self-similar object is exactly or approximately similar to a part of itself. Many objects in the real world, such as coastlines, are statistically self-similar: parts of them show the same statistical properties at many scales. Self-similarity is a typical property of artificial fractals. Scale invariance is an exact form of self-similarity where at any magnification there is a smaller piece of the object that is similar to the whole. For instance, a side of the Koch snowflake is both symmetrical and scale-invariant; it can be continually magnified 3x without changing shape. The non-trivial similarity evident in fractals is distinguished by their fine structure, or detail on arbitrarily small scales. As a counterexample, whereas any portion of a straight line may resemble the whole, further detail is not revealed.

Geometry branch of mathematics that measures the shape, size and position of objects

Geometry is a branch of mathematics concerned with questions of shape, size, relative position of figures, and the properties of space. A mathematician who works in the field of geometry is called a geometer.

Pentagram star polygon

A pentagram is the shape of a five-pointed star.

Contents

Constructions

The lute may be drawn from a sequence of pentagrams. The centers of the pentagraphs lie on a line and (except for the first and largest of them) each shares two vertices with the next larger one in the sequence. [1] [2]

In geometry, a vertex is a point where two or more curves, lines, or edges meet. As a consequence of this definition, the point where two lines meet to form an angle and the corners of polygons and polyhedra are vertices.

An alternative construction is based on the golden triangle, an isosceles triangle with base angles of 72° and apex angle 36°. Two smaller copies of the same triangle may be drawn inside the given triangle, having the base of the triangle as one of their sides. The two new edges of these two smaller triangles, together with the base of the original golden triangle, form three of the five edges of the polygon. Adding a segment between the endpoints of these two new edges cuts off a smaller golden triangle, within which the construction can be repeated. [3] [4]

Golden triangle (mathematics) isosceles triangle in which the duplicated side is in the golden ratio to the distinct side

A golden triangle, also known as the sublime triangle,is an isosceles triangle in which the duplicated side is in the golden ratio to the distinct side:

Isosceles triangle triangle with at least two sides congruent

In geometry, an isosceles triangle is a triangle that has two sides of equal length. Sometimes it is specified as having exactly two sides of equal length, and sometimes as having at least two sides of equal length, the latter version thus including the equilateral triangle as a special case. Examples of isosceles triangles include the isosceles right triangle, the golden triangle, and the faces of bipyramids and certain Catalan solids.

Some sources add another pentagram, inscribed within the inner pentagon of the largest pentagram of the figure. The other pentagons of the figure do not have inscribed pentagrams. [3] [4] [5]

Properties

The convex hull of the lute is a kite shape with three 108° angles and one 36° angle. [2] The sizes of any two consecutive pentagrams in the sequence are in the golden ratio to each other, and many other instances of the golden ratio appear within the lute. [1] [2] [3] [4] [5]

Convex hull Notion in topological vector spaces

In mathematics, the convex hull or convex envelope or convex closure of a set X of points in the Euclidean plane or in a Euclidean space is the smallest convex set that contains X. For instance, when X is a bounded subset of the plane, the convex hull may be visualized as the shape enclosed by a rubber band stretched around X.

Kite (geometry) quadrilateral whose four sides can be grouped into two pairs of equal-length sides that are adjacent to each other

In Euclidean geometry, a kite is a quadrilateral whose four sides can be grouped into two pairs of equal-length sides that are adjacent to each other. In contrast, a parallelogram also has two pairs of equal-length sides, but they are opposite to each other rather than adjacent. Kite quadrilaterals are named for the wind-blown, flying kites, which often have this shape and which are in turn named for a bird. Kites are also known as deltoids, but the word "deltoid" may also refer to a deltoid curve, an unrelated geometric object.

Golden ratio ratio between two quantities whose sum is at the same ratio to the larger one

In mathematics, two quantities are in the golden ratio if their ratio is the same as the ratio of their sum to the larger of the two quantities. The figure on the right illustrates the geometric relationship. Expressed algebraically, for quantities a and b with a > b > 0,

History

The lute is named after the ancient Greek mathematician Pythagoras, but its origins are unclear. [3] An early reference to it is in a 1990 book on the golden ratio by Boles and Newman. [6]

Pythagoras ancient Greek philosopher and mystic

Pythagoras of Samos was an ancient Ionian Greek philosopher and the eponymous founder of Pythagoreanism. His political and religious teachings were well known in Magna Graecia and influenced the philosophies of Plato, Aristotle, and, through them, Western philosophy. Knowledge of his life is clouded by legend, but he appears to have been the son of Mnesarchus, a seal engraver on the island of Samos. Modern scholars disagree regarding Pythagoras's education and influences, but they do agree that, around 530 BC, he travelled to Croton, where he founded a school in which initiates were sworn to secrecy and lived a communal, ascetic lifestyle. This lifestyle entailed a number of dietary prohibitions, traditionally said to have included vegetarianism, although modern scholars doubt that he ever advocated for complete vegetarianism.

See also

Related Research Articles

Regular icosahedron Platonic solid

In geometry, a regular icosahedron is a convex polyhedron with 20 faces, 30 edges and 12 vertices. It is one of the five Platonic solids, and the one with the most sides.

In elementary geometry, a polygon is a plane figure that is described by a finite number of straight line segments connected to form a closed polygonal chain or polygonal circuit. The solid plane region, the bounding circuit, or the two together, may be called a polygon.

Star polygon regular non-convex polygon

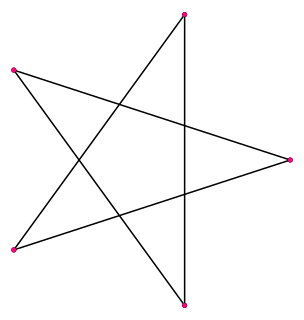
In geometry, a star polygon is a type of non-convex polygon. Only the regular star polygons have been studied in any depth; star polygons in general appear not to have been formally defined.

Decagon shape with ten sides

In geometry, a decagon is a ten-sided polygon or 10-gon.

Schläfli symbol notation that defines regular polytopes and tessellations

In geometry, the Schläfli symbol is a notation of the form {p,q,r,...} that defines regular polytopes and tessellations.

Spidron A geometric figure composed of triangles with shared legs between adjacent triangles creating a spiral, often within a polygon.

In geometry, a spidron is a continuous flat geometric figure composed entirely of triangles, where, for every pair of joining triangles, each has a leg of the other as one of its legs, and neither has any point inside the interior of the other. A deformed spidron is a three-dimensional figure sharing the other properties of a specific spidron, as if that spidron were drawn on paper, cut out in a single piece, and folded along a number of legs.

<i>Donald in Mathmagic Land</i> 1959 animated short film

Donald in Mathmagic Land is a 27-minute Donald Duck educational featurette released on June 26, 1959. The film was nominated for an Academy Award, and became a widely viewed educational film in American schools of the 1960s.

Special right triangle right triangle with a feature making calculations on the triangle easier

A special right triangle is a right triangle with some regular feature that makes calculations on the triangle easier, or for which simple formulas exist. For example, a right triangle may have angles that form simple relationships, such as 45°–45°–90°. This is called an "angle-based" right triangle. A "side-based" right triangle is one in which the lengths of the sides form ratios of whole numbers, such as 3 : 4 : 5, or of other special numbers such as the golden ratio. Knowing the relationships of the angles or ratios of sides of these special right triangles allows one to quickly calculate various lengths in geometric problems without resorting to more advanced methods.

Small stellated dodecahedron Kepler-Poinsot polyhedron

In geometry, the small stellated dodecahedron is a Kepler-Poinsot polyhedron, named by Arthur Cayley, and with Schläfli symbol {5/2,5}. It is one of four nonconvex regular polyhedra. It is composed of 12 pentagrammic faces, with five pentagrams meeting at each vertex.

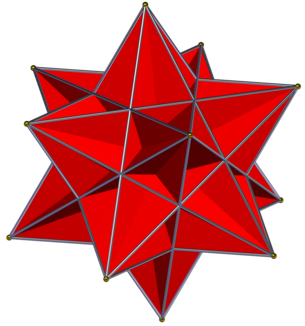
Great icosahedron Kepler–Poinsot polyhedron

In geometry, the great icosahedron is one of four Kepler-Poinsot polyhedra, with Schläfli symbol {3,5/2} and Coxeter-Dynkin diagram of . It is composed of 20 intersecting triangular faces, having five triangles meeting at each vertex in a pentagrammic sequence.

Regular dodecahedron Platonic solid

A regular dodecahedron or pentagonal dodecahedron is a dodecahedron that is regular, which is composed of twelve regular pentagonal faces, three meeting at each vertex. It is one of the five Platonic solids. It has 12 faces, 20 vertices, 30 edges, and 160 diagonals. It is represented by the Schläfli symbol {5,3}.

Kepler triangle A right triangle whose edge lengths form a geometric progression

A Kepler triangle is a right triangle with edge lengths in a geometric progression in which the common ratio is √φ, where φ is the golden ratio, and can be written: , or approximately 1 : 1.272 : 1.618. The squares of the edges of this triangle are in geometric progression according to the golden ratio.

Pentagon shape with five sides

In geometry, a pentagon is any five-sided polygon or 5-gon. The sum of the internal angles in a simple pentagon is 540°.

Pythagorean theorem Equation relating the side lengths of a right triangle

In mathematics, the Pythagorean theorem, also known as Pythagoras' theorem, is a fundamental relation in Euclidean geometry among the three sides of a right triangle. It states that the square of the hypotenuse is equal to the sum of the squares of the other two sides. The theorem can be written as an equation relating the lengths of the sides a, b and c, often called the "Pythagorean equation":

Penrose tiling non-periodic tiling of the plane

A Penrose tiling is an example of non-periodic tiling generated by an aperiodic set of prototiles. Penrose tilings are named after mathematician and physicist Roger Penrose, who investigated these sets in the 1970s. The aperiodicity of prototiles implies that a shifted copy of a tiling will never match the original. A Penrose tiling may be constructed so as to exhibit both reflection symmetry and fivefold rotational symmetry, as in the diagram at the right.

Pythagorean tiling

A Pythagorean tiling or two squares tessellation is a tiling of a Euclidean plane by squares of two different sizes, in which each square touches four squares of the other size on its four sides. Many proofs of the Pythagorean theorem are based on it, explaining its name. It is commonly used as a pattern for floor tiles. When used for this, it is also known as a hopscotch pattern or pinwheel pattern, but it should not be confused with the mathematical pinwheel tiling, an unrelated pattern.

References

  1. 1 2 Gullberg, Jan (1997), Mathematics: From the Birth of Numbers, W. W. Norton & Company, p. 420, ISBN   9780393040029 .
  2. 1 2 3 Darling, David (2004), The Universal Book of Mathematics: From Abracadabra to Zeno's Paradoxes, John Wiley & Sons, p. 260, ISBN   9780471667001 .
  3. 1 2 3 4 Lamb, Evelyn (May 29, 2013), "Strumming the Lute of Pythagoras", Scientific American .
  4. 1 2 3 Ellison, Elaine Krajenke (2008), "Create a Mathematical Banner Using the Lute, the Sacred Cut, and the Spidron", Bridges Leeuwarden: Mathematics, Music, Art, Architecture, Culture, pp. 467–468.
  5. 1 2 Pickover, Clifford A. (2011), A Passion for Mathematics: Numbers, Puzzles, Madness, Religion, and the Quest for Reality, John Wiley & Sons, pp. 331–332, ISBN   9781118046074 .
  6. ↑ Boles, Martha; Newman, Rochelle (1990), The Golden Relationship: Universal patterns, Pythagorean Press, pp. 86–87, ISBN   9780961450434 .
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.