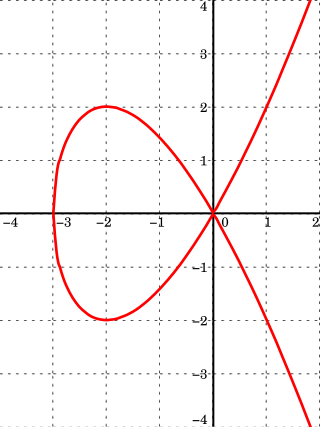Toric variety

Last updated November 25, 2023

In algebraic geometry, a toric variety or torus embedding is an algebraic variety containing an algebraic torus as an open dense subset, such that the action of the torus on itself extends to the whole variety. Some authors also require it to be normal. Toric varieties form an important and rich class of examples in algebraic geometry, which often provide a testing ground for theorems. The geometry of a toric variety is fully determined by the combinatorics of its associated fan, which often makes computations far more tractable. For a certain special, but still quite general class of toric varieties, this information is also encoded in a polytope, which creates a powerful connection of the subject with convex geometry. Familiar examples of toric varieties are affine space, projective spaces, products of projective spaces and bundles over projective space.

Toric varieties from tori

The original motivation to study toric varieties was to study torus embeddings. Given the algebraic torus T, the group of characters Hom(T,C^x) forms a lattice. Given a collection of points A, a subset of this lattice, each point determines a map to C and thus the collection determines a map to C^|A|. By taking the Zariski closure of the image of such a map, one obtains an affine variety. If the collection of lattice points A generates the character lattice, this variety is a torus embedding. In similar fashion one may produce a parametrized projective toric variety, by taking the projective closure of the above map, viewing it as a map into an affine patch of projective space.

Given a projective toric variety, observe that we may probe its geometry by one-parameter subgroups. Each one parameter subgroup, determined by a point in the lattice, dual to the character lattice, is a punctured curve inside the projective toric variety. Since the variety is compact, this punctured curve has a unique limit point. Thus, by partitioning the one-parameter subgroup lattice by the limit points of punctured curves, we obtain a lattice fan, a collection of polyhedral rational cones. The cones of highest dimension correspond precisely to the torus fixed points, the limits of these punctured curves.

The toric variety of a fan

Suppose that N is a finite-rank free abelian group. A strongly convex rational polyhedral cone in N is a convex cone (of the real vector space of N) with apex at the origin, generated by a finite number of vectors of N, that contains no line through the origin. These will be called "cones" for short.

For each cone σ its affine toric variety U_σ is the spectrum of the semigroup algebra of the dual cone.

A fan is a collection of cones closed under taking intersections and faces.

The toric variety of a fan is given by taking the affine toric varieties of its cones and gluing them together by identifying U_σ with an open subvariety of U_τ whenever σ is a face of τ. Conversely, every fan of strongly convex rational cones has an associated toric variety.

The fan associated with a toric variety condenses some important data about the variety. For example, a variety is smooth if every cone in its fan can be generated by a subset of a basis for the free abelian group N.

Morphisms of toric varieties

Suppose that Δ₁ and Δ₂ are fans in lattices N₁ and N₂. If f is a linear map from N₁ to N₂ such that the image of every cone of Δ₁ is contained in a cone of Δ₂, then f induces a morphism f_* between the corresponding toric varieties. This map f_* is proper if and only if the preimage of |Δ₂| under the map f is |Δ₁|, where |Δ| is the underlying space of a fan Δ given by the union of its cones.

Resolution of singularities

A toric variety is nonsingular if its cones of maximal dimension are generated by a basis of the lattice. This implies that every toric variety has a resolution of singularities given by another toric variety, which can be constructed by subdividing the maximal cones into cones of nonsingular toric varieties.

The toric variety of a convex polytope

The fan of a rational convex polytope in N consists of the cones over its proper faces. The toric variety of the polytope is the toric variety of its fan. A variation of this construction is to take a rational polytope in the dual of N and take the toric variety of its polar set in N.

The toric variety has a map to the polytope in the dual of N whose fibers are topological tori. For example, the complex projective plane CP² may be represented by three complex coordinates satisfying

|z_{1}|^{2}+|z_{2}|^{2}+|z_{3}|^{2}=1,\,\!

where the sum has been chosen to account for the real rescaling part of the projective map, and the coordinates must be moreover identified by the following U(1) action:

(z_{1},z_{2},z_{3})\approx e^{i\phi }(z_{1},z_{2},z_{3}).\,\!

The approach of toric geometry is to write

(x,y,z)=(|z_{1}|^{2},|z_{2}|^{2},|z_{3}|^{2}).\,\!

The coordinates $x,y,z$ are non-negative, and they parameterize a triangle because

x+y+z=1;\,\!

that is,

\quad z=1-x-y.\,\!

The triangle is the toric base of the complex projective plane. The generic fiber is a two-torus parameterized by the phases of $z_{1},z_{2}$ ; the phase of $z_{3}$ can be chosen real and positive by the $U(1)$ symmetry.

However, the two-torus degenerates into three different circles on the boundary of the triangle i.e. at $x=0$ or $y=0$ or $z=0$ because the phase of $z_{1},z_{2},z_{3}$ becomes inconsequential, respectively.

The precise orientation of the circles within the torus is usually depicted by the slope of the line intervals (the sides of the triangle, in this case).

Relation to mirror symmetry

The idea of toric varieties is useful for mirror symmetry because an interpretation of certain data of a fan as data of a polytope leads to a geometric construction of mirror manifolds.

Related Research Articles

In mathematics, particularly in complex analysis, a Riemann surface is a one-dimensional complex manifold.

In mathematics, complex geometry is the study of geometric structures and constructions arising out of, or described by, the complex numbers. In particular, complex geometry is concerned with the study of spaces such as complex manifolds and complex algebraic varieties, functions of several complex variables, and holomorphic constructions such as holomorphic vector bundles and coherent sheaves. Application of transcendental methods to algebraic geometry falls in this category, together with more geometric aspects of complex analysis.

In mathematics, particularly in algebraic geometry, complex analysis and algebraic number theory, an abelian variety is a projective algebraic variety that is also an algebraic group, i.e., has a group law that can be defined by regular functions. Abelian varieties are at the same time among the most studied objects in algebraic geometry and indispensable tools for much research on other topics in algebraic geometry and number theory.

Algebraic varieties are the central objects of study in algebraic geometry, a sub-field of mathematics. Classically, an algebraic variety is defined as the set of solutions of a system of polynomial equations over the real or complex numbers. Modern definitions generalize this concept in several different ways, while attempting to preserve the geometric intuition behind the original definition.

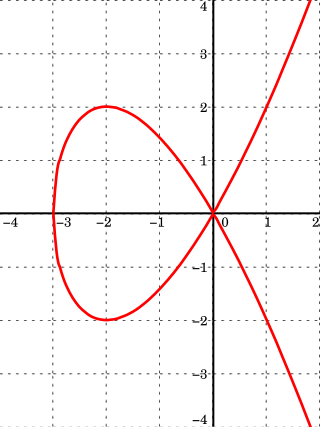
In mathematics, an affine algebraic plane curve is the zero set of a polynomial in two variables. A projective algebraic plane curve is the zero set in a projective plane of a homogeneous polynomial in three variables. An affine algebraic plane curve can be completed in a projective algebraic plane curve by homogenizing its defining polynomial. Conversely, a projective algebraic plane curve of homogeneous equation $h (x, y, t) = 0$ can be restricted to the affine algebraic plane curve of equation $h (x, y, 1) = 0$ . These two operations are each inverse to the other; therefore, the phrase algebraic plane curve is often used without specifying explicitly whether it is the affine or the projective case that is considered.

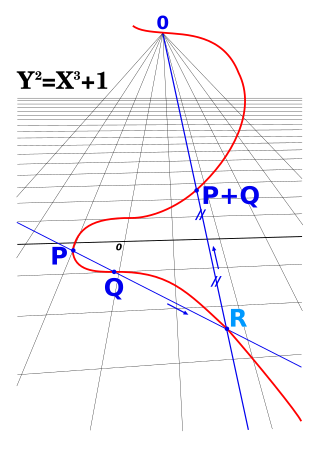
In algebraic geometry, a projective variety over an algebraically closed field k is a subset of some projective n-space $over k that is the zero-locus of some finite family of homogeneous polynomials of n + 1 variables with coefficients in k, that generate a prime ideal, the defining ideal of the variety. Equivalently, an algebraic variety is projective if it can be embedded as a Zariski closed subvariety of .$

<span class="mw-page-title-main">Birational geometry</span> Field of algebraic geometry

In mathematics, birational geometry is a field of algebraic geometry in which the goal is to determine when two algebraic varieties are isomorphic outside lower-dimensional subsets. This amounts to studying mappings that are given by rational functions rather than polynomials; the map may fail to be defined where the rational functions have poles.

In mathematics, an algebraic torus, where a one dimensional torus is typically denoted by $,, or, is a type of commutative affine algebraic group commonly found in projective algebraic geometry and toric geometry. Higher dimensional algebraic tori can be modelled as a product of algebraic groups . These groups were named by analogy with the theory of tori in Lie group theory. For example, over the complex numbers the algebraic torus is isomorphic to the group scheme, which is the scheme theoretic analogue of the Lie group . In fact, any -action on a complex vector space can be pulled back to a -action from the inclusion as real manifolds.$

In mathematics, a duality translates concepts, theorems or mathematical structures into other concepts, theorems or structures in a one-to-one fashion, often by means of an involution operation: if the dual of $A$ is $B$ , then the dual of $B$ is $A$ . Such involutions sometimes have fixed points, so that the dual of $A$ is $A$ itself. For example, Desargues' theorem is self-dual in this sense under the standard duality in projective geometry.

In linear algebra, a cone—sometimes called a linear cone for distinguishing it from other sorts of cones—is a subset of a vector space that is closed under positive scalar multiplication; that is, $C$ is a cone if $implies for every positive scalar s .$

In mathematics, a surface is a mathematical model of the common concept of a surface. It is a generalization of a plane, but, unlike a plane, it may be curved; this is analogous to a curve generalizing a straight line.

In mathematics, specifically in combinatorial commutative algebra, a convex lattice polytope P is called normal if it has the following property: given any positive integer n, every lattice point of the dilation nP, obtained from P by scaling its vertices by the factor n and taking the convex hull of the resulting points, can be written as the sum of exactly n lattice points in P. This property plays an important role in the theory of toric varieties, where it corresponds to projective normality of the toric variety determined by P. Normal polytopes have popularity in algebraic combinatorics. These polytopes also represent the homogeneous case of the Hilbert bases of finite positive rational cones and the connection to algebraic geometry is that they define projectively normal embeddings of toric varieties.

Gordan's lemma is a lemma in convex geometry and algebraic geometry. It can be stated in several ways.

In algebraic geometry, a morphism between algebraic varieties is a function between the varieties that is given locally by polynomials. It is also called a regular map. A morphism from an algebraic variety to the affine line is also called a regular function. A regular map whose inverse is also regular is called biregular, and the biregular maps are the isomorphisms of algebraic varieties. Because regular and biregular are very restrictive conditions – there are no non-constant regular functions on projective varieties – the concepts of rational and birational maps are widely used as well; they are partial functions that are defined locally by rational fractions instead of polynomials.

polymake is software for the algorithmic treatment of convex polyhedra.

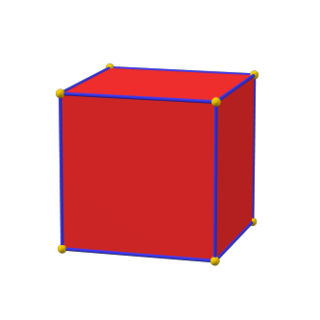
In geometry and polyhedral combinatorics, an integral polytope is a convex polytope whose vertices all have integer Cartesian coordinates. That is, it is a polytope that equals the convex hull of its integer points. Integral polytopes are also called lattice polytopes or Z-polytopes. The special cases of two- and three-dimensional integral polytopes may be called polygons or polyhedra instead of polytopes, respectively.

This is a glossary of algebraic geometry.

In abstract algebra, a branch of mathematics, an affine monoid is a commutative monoid that is finitely generated, and is isomorphic to a submonoid of a free abelian group $. Affine monoids are closely connected to convex polyhedra, and their associated algebras are of much use in the algebraic study of these geometric objects.$

A purely combinatorial approach to mirror symmetry was suggested by Victor Batyrev using the polar duality for $-dimensional convex polyhedra. The most famous examples of the polar duality provide Platonic solids: e.g., the cube is dual to octahedron, the dodecahedron is dual to icosahedron. There is a natural bijection between the -dimensional faces of a -dimensional convex polyhedron and -dimensional faces of the dual polyhedron and one has . In Batyrev's combinatorial approach to mirror symmetry the polar duality is applied to special -dimensional convex lattice polytopes which are called reflexive polytopes.$

In mathematics, and especially differential and algebraic geometry, K-stability is an algebro-geometric stability condition, for complex manifolds and complex algebraic varieties. The notion of K-stability was first introduced by Gang Tian and reformulated more algebraically later by Simon Donaldson. The definition was inspired by a comparison to geometric invariant theory (GIT) stability. In the special case of Fano varieties, K-stability precisely characterises the existence of Kähler–Einstein metrics. More generally, on any compact complex manifold, K-stability is conjectured to be equivalent to the existence of constant scalar curvature Kähler metrics.

References

Cox, David (2003), "What is a toric variety?", Topics in algebraic geometry and geometric modeling, Contemp. Math., vol. 334, Providence, R.I.: Amer. Math. Soc., pp. 203–223, MR 2039974
Cox, David A.; Little, John B.; Schenck, Hal, Toric varieties
Danilov, V. I. (1978), "The geometry of toric varieties", Akademiya Nauk SSSR I Moskovskoe Matematicheskoe Obshchestvo. Uspekhi Matematicheskikh Nauk, 33 (2): 85–134, doi:10.1070/RM1978v033n02ABEH002305, ISSN 0042-1316, MR 0495499
Fulton, William (1993), Introduction to toric varieties, Princeton University Press, ISBN 978-0-691-00049-7
Kempf, G.; Knudsen, Finn Faye; Mumford, David; Saint-Donat, B. (1973), Toroidal embeddings. I, Lecture Notes in Mathematics, vol. 339, Berlin, New York: Springer-Verlag, doi: 10.1007/BFb0070318 , ISBN 978-3-540-06432-9, MR 0335518
Miller, Ezra (2008), "What is ... a toric variety?" (PDF), Notices of the American Mathematical Society , 55 (5): 586–587, ISSN 0002-9920, MR 2404030
Oda, Tadao (1988), Convex bodies and algebraic geometry, Ergebnisse der Mathematik und ihrer Grenzgebiete (3) [Results in Mathematics and Related Areas (3)], vol. 15, Berlin, New York: Springer-Verlag, ISBN 978-3-540-17600-8, MR 0922894

External links

Home page of D. A. Cox, with several lectures on toric varieties