Arlie Petters

Last updated
Arlie Petters
Petters 2011.jpg
Born
Arlie Oswald Petters

(1964-02-08) February 8, 1964 (age 59)
Dangriga, Belize
(formerly Stann Creek Town, British Honduras)
Alma mater Hunter College
Massachusetts Institute of Technology
Known forMathematical theory of gravitational lensing
AwardsAlfred P. Sloan Fellowship
NSF CAREER Award
Blackwell-Tapia Prize
Most Excellent Order of the British Empire
Scientific career
Doctoral advisors Bertram Kostant
David Spergel

Arlie Oswald Petters, MBE (born February 8, 1964) is a Belizean-American mathematical physicist, who is the Benjamin Powell Professor of mathematics and a professor of physics and economics at Duke University. [1] Petters became the provost at New York University Abu Dhabi effective September 1, 2020. [2] Petters is a founder of mathematical astronomy, focusing on problems connected to the interplay of gravity and light and employing tools from astrophysics, cosmology, general relativity, high energy physics, differential geometry, singularities, and probability theory. [3] His monograph "Singularity Theory and Gravitational Lensing" developed a mathematical theory of gravitational lensing. Petters was also the dean of academic affairs for Trinity College of Arts and Sciences and associate vice provost for undergraduate education at Duke University (2016-2019). [4]

Contents

Biography

Petters was raised by his grandparents in the rural community of Dangriga, Belize (formerly Stann Creek Town, British Honduras). His mother immigrated to Brooklyn, New York, and married a U.S. citizen, with Arlie joining them when he was 14 years old. [5]

Petters earned a B.A./M.A. in Mathematics and Physics from Hunter College, CUNY in 1986 with a thesis on "The Mathematical Theory of General Relativity", and began his Ph.D. at the Massachusetts Institute of Technology Department of Mathematics in the same year. After two years of doctoral studies, he became an exchange scholar in the Princeton University Department of Physics in absentia from MIT. Petters earned his Ph.D. in mathematics in 1991 under advisors Bertram Kostant (MIT) and David Spergel (Princeton University). He remained at MIT for two years as an instructor of pure mathematics (1991–1993) and then joined the faculty at Princeton University in the Department of Mathematics. He was an assistant professor at Princeton for five years (1993–1998) before moving to Duke University. [4] [6]

Many media outlets have profiled Arlie Petters and his scholarship, including The New York Times , [5] NOVA, [7] The HistoryMakers (a digital archive of oral histories featuring African-Americans and preserved at the Library of Congress [8] ), [9] Big Think, [10] and Duke University's news outlet, The Chronicle. [11]

Research

Petters is known for his work in the mathematical theory of gravitational lensing.

Over the ten-year period from 1991 to 2001, Petters systematically developed a mathematical theory of weak-deflection gravitational lensing, beginning with his 1991 MIT Ph.D. thesis on "Singularities in Gravitational Microlensing". [12] In a series of papers, he and his collaborators resolved an array of theoretical problems in weak-deflection gravitational lensing covering image counting, fixed-point images, image magnification, image time delays, local geometry of caustics, global geometry of caustics, wavefronts, caustic surfaces, and caustic surfing. [13] His work culminated in book, entitled Singularity Theory and Gravitational Lensing (Springer 2012), which he co-authored with Harold Levine and Joachim Wambganns. This book, which addressed the question, "What is the universe made of?", systematically created a framework of stability and genericity for k-plane gravitational lensing. [14] The book drew upon powerful tools from the theory of singularities and put the subject of weak-deflection k-plane gravitational lensing on a rigorous and unified mathematical foundation. [14]

Following his 1991–2001 body of mathematical lensing work, Petters turned to more astrophysical lensing issues from 2002 to 2005. In collaboration with astronomers, he applied some of the mathematical theory in [AP13] to help develop a practical diagnostic test for the presence of dark substructures in galaxies lensing quasars; [15] [16] classify the local astrometric (centroid) and photometric curves of an extended source when it crosses fold and cusp caustics due to generic lenses; [17] [18] predict the quantitative astrometric curve's shape produced by Galactic binary lenses. [17] [18] The classified local properties of the astrometric curves revealed a characteristic S-shape for fold crossings, parabolic and swallowtail features for cusp crossings, and a jump discontinuity for crossings over the fold arcs merging into a cusp. Petters, Levine, and Wambgamnns also developed a formula to calculate the size of the jump.

During the period from 2005 to 2007, Petters collaborated with astronomers and physicists to explore gravitational lensing in directions beyond its traditional confines in astronomy. In a series of three mathematical physics papers published written with the astronomer Charles R. Keeton, he utilized higher-order gravitational lensing effects by compact bodies to test different theories of gravity with the general theory of relativity of Einstein among them. These papers computed beyond the standard weak-deflection limit the first- and second-order corrections to the image positions, magnifications, and time delays due to lensing in general relativity and alternative gravitational theories describable within the PPN formalism, [19] and even determined lensing invariants for the PPN family of models. [20] Their findings were applied to the Galactic black hole, binary pulsars, and gravitational microlensing scenarios to make testable predictions about lensed images and their time delays. [19] Another paper took on the difficult issue of how to test hyperspace models like braneworld gravity that postulate an extra dimension to physical space. The paper developed a semi-classical wave theory of braneworld black hole lensing and used that theory along with braneworld cosmology to predict a testable signature of microscopic braneworld black holes on gamma-ray light. [21] [22] Additionally, in a 2007 paper, Petters and M.C. Werner found a system of equations that can be applied to test the Cosmic Censorship Hypothesis observationally using the realistic case of lensing by a Kerr black hole. [23] [24]

Petters's previous work (1991–2007) dealt with non-random gravitational lensing. Starting in 2008, his research program focused on developing a mathematical theory of random (stochastic) gravitational lensing. In two papers, Petters, Rider, and Teguia took first steps in creating a mathematical theory of stochastic gravitational microlensing. They characterized to several asymptotic orders the probability densities of random time delay functions, lensing maps, and shear maps in stochastic microlensing and determined a Kac-Rice type formula for the global expected number of images due to a general stochastic lens system. [25] [26] The work forms a concrete framework from which extensions to more general random maps can be made. In two additional papers, he and Aazami found geometric universal magnification invariants of higher-order caustics occurring in lensing and caustics produced by generic general maps up to codimension five. [27] [28] [29] The invariants hold with a probability of 1 for random lenses and thereby form important consistency checks for research on random image magnifications of sources near stable caustics.

Social outreach

Petters has served as director of the Reginaldo Howard Memorial Scholarship program at Duke University. [30] He has also been active in the African-American community particularly through his mentoring, recruiting, and lecturing. [31] [32] He has received several community service awards for his social outreach. [33] [34] Petters is the first tenured African-American professor in mathematics at Duke University. [33] He is very involved in the Belizean community and founded the Petters Research Institute [35] in 2005 to help train Belizean young people in STEM fields and foster STEM entrepreneurship. [36] [37] He has written five books, three of them science and mathematics problem-solving books for Belizean students. [38] [39] Some of his entrepreneurial work was conducted while he was a professor of business administration at Duke's Fuqua School of Business (2008–2017). [4] Petters also served the Government of Belize as chairman of the Council of Science Advisers to the prime minister of Belize (2010–2013). [40] [4]

Awards and honors

Petters has received numerous awards and honors. [41] He was won an Alfred P. Sloan Research Fellowship in Mathematics (1998), [42] and a CAREER award from the National Science Foundation (1998), [43] and was the first winner of the Blackwell-Tapia Prize (2002). [44] He was selected in 2006 by the National Academy of Sciences to be part of a permanent Portrait Collection of Outstanding African-Americans in Science, Engineering, and Medicine. [45] In 2008 Petters was also included among the Human Relations Associates' list of "The Twenty-Five Greatest Scientists of African Ancestry,"going back to the eighteenth century. [46] He received an honorary Doctor of Science from his alma mater, Hunter College, in 2008. [47] Petters was named by the Queen of the United Kingdom in 2008 to membership in the Most Excellent Order of the British Empire. [48] In recognition of his scientific accomplishments and service to society, Petters's birthplace—Dangriga, Belize—honored him in 2009 by naming a road Dr. Arlie Petters Street. [49] He became in 2011 the first Belizean to receive the Caribbean American Heritage Award for Excellence in Science and Technology. [50] In 2012 he became a fellow of the American Mathematical Society [51] and the first Belizean American to be Grand Marshal of the Central American Day Parade in Los Angeles, where he received honors from the mayor and from the Confederation Centroamericana (COFECA). [52] [4] Petters was recognized by Mathematically Gifted & Black as a Black History Month 2017 Honoree. [53]

Related Research Articles

<span class="mw-page-title-main">General relativity</span> Theory of gravitation as curved spacetime

General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics. General relativity generalises special relativity and refines Newton's law of universal gravitation, providing a unified description of gravity as a geometric property of space and time or four-dimensional spacetime. In particular, the curvature of spacetime is directly related to the energy and momentum of whatever matter and radiation are present. The relation is specified by the Einstein field equations, a system of second order partial differential equations.

<span class="mw-page-title-main">Gravitational lens</span> Light bending by mass between source and observer

A gravitational lens is a distribution of matter or a point particle between a distant light source and an observer that is capable of bending the light from the source as the light travels toward the observer. This effect is known as gravitational lensing, and the amount of bending is one of the predictions of Albert Einstein's general theory of relativity. Treating light as corpuscles travelling at the speed of light, Newtonian physics also predicts the bending of light, but only half of that predicted by general relativity.

A massive astrophysical compact halo object (MACHO) is a kind of astronomical body that might explain the apparent presence of dark matter in galaxy halos. A MACHO is a body that emits little or no radiation and drifts through interstellar space unassociated with any planetary system. Since MACHOs are not luminous, they are hard to detect. MACHO candidates include black holes or neutron stars as well as brown dwarfs and unassociated planets. White dwarfs and very faint red dwarfs have also been proposed as candidate MACHOs. The term was coined by astrophysicist Kim Griest.

The Penrose–Hawking singularity theorems are a set of results in general relativity that attempt to answer the question of when gravitation produces singularities. The Penrose singularity theorem is a theorem in semi-Riemannian geometry and its general relativistic interpretation predicts a gravitational singularity in black hole formation. The Hawking singularity theorem is based on the Penrose theorem and it is interpreted as a gravitational singularity in the Big Bang situation. Penrose was awarded the Nobel Prize in Physics in 2020 "for the discovery that black hole formation is a robust prediction of the general theory of relativity", which he shared with Reinhard Genzel and Andrea Ghez.

Cosmic strings are hypothetical 1-dimensional topological defects which may have formed during a symmetry-breaking phase transition in the early universe when the topology of the vacuum manifold associated to this symmetry breaking was not simply connected. Their existence was first contemplated by the theoretical physicist Tom Kibble in the 1970s.

A variable speed of light (VSL) is a feature of a family of hypotheses stating that the speed of light may in some way not be constant, for example, that it varies in space or time, or depending on frequency. Accepted classical theories of physics, and in particular general relativity, predict a constant speed of light in any local frame of reference and in some situations these predict apparent variations of the speed of light depending on frame of reference, but this article does not refer to this as a variable speed of light. Various alternative theories of gravitation and cosmology, many of them non-mainstream, incorporate variations in the local speed of light.

<span class="mw-page-title-main">Dark matter halo</span> Theoretical cosmological structure

According to modern models of physical cosmology, a dark matter halo is a basic unit of cosmological structure. It is a hypothetical region that has decoupled from cosmic expansion and contains gravitationally bound matter. A single dark matter halo may contain multiple virialized clumps of dark matter bound together by gravity, known as subhalos. Modern cosmological models, such as ΛCDM, propose that dark matter halos and subhalos may contain galaxies. The dark matter halo of a galaxy envelops the galactic disc and extends well beyond the edge of the visible galaxy. Thought to consist of dark matter, halos have not been observed directly. Their existence is inferred through observations of their effects on the motions of stars and gas in galaxies and gravitational lensing. Dark matter halos play a key role in current models of galaxy formation and evolution. Theories that attempt to explain the nature of dark matter halos with varying degrees of success include cold dark matter (CDM), warm dark matter, and massive compact halo objects (MACHOs).

<span class="mw-page-title-main">Gravitational microlensing</span> Astronomical phenomenon due to the gravitational lens effect

Gravitational microlensing is an astronomical phenomenon due to the gravitational lens effect. It can be used to detect objects that range from the mass of a planet to the mass of a star, regardless of the light they emit. Typically, astronomers can only detect bright objects that emit much light (stars) or large objects that block background light. These objects make up only a minor portion of the mass of a galaxy. Microlensing allows the study of objects that emit little or no light. Gravitational microlensing was first theorised by Refstal (1964) and first discovered by Irwin et al (1988). The first object in the sky where it was discovered was the Einstein cross or Huchra lens 2237 +0305. The initial lightcurve of the object was published by Corrigan et al (1991). In Corrigan et al (1991) they calculated that the object causing the microlensing was a Jupiter sized object. This was the first discovery of a planet in another galaxy.

<span class="mw-page-title-main">Optical Gravitational Lensing Experiment</span> Long-term variability sky survey

The Optical Gravitational Lensing Experiment (OGLE) is a Polish astronomical project based at the University of Warsaw that runs a long-term variability sky survey (1992–present). The main goals are the detection and classification of variable stars, discovery of microlensing events, dwarf novae, and studies of the structure of the Galaxy and the Magellanic Clouds. Since the project began in 1992, it has discovered a multitude of extrasolar planets, together with the first planet discovered using the transit method (OGLE-TR-56b) and gravitational microlensing. The project has been led by professor Andrzej Udalski since its inception.

<span class="mw-page-title-main">Microlensing Observations in Astrophysics</span>

Microlensing Observations in Astrophysics (MOA) is a collaborative project between researchers in New Zealand and Japan, led by Professor Yasushi Muraki of Nagoya University. They use microlensing to observe dark matter, extra-solar planets, and stellar atmospheres from the Southern Hemisphere. The group concentrates especially on the detection and observation of gravitational microlensing events of high magnification, of order 100 or more, as these provide the greatest sensitivity to extrasolar planets. They work with other groups in Australia, the United States and elsewhere. Observations are conducted at New Zealand's Mt. John University Observatory using a 1.8 m (70.9 in) reflector telescope built for the project.

<span class="mw-page-title-main">Bullet Cluster</span> Two colliding clusters of galaxies in constellation Carina

The Bullet Cluster consists of two colliding clusters of galaxies. Strictly speaking, the name Bullet Cluster refers to the smaller subcluster, moving away from the larger one. It is at a comoving radial distance of 1.141 Gpc.

Xallarap is a variation in a gravitational lensing observation caused by the orbital motion of the source. A more traditional and similar effect, parallax, is the variation caused by motion of the Earth around the Sun. Since the two effects are converses of each other, this led to the name xallarap, which is parallax spelled backwards. A survey of microlensing attributes the first use in print to Bennett in 1998, though informal usage likely preceded this.

<span class="mw-page-title-main">Jürgen Ehlers</span> German physicist

Jürgen Ehlers was a German physicist who contributed to the understanding of Albert Einstein's theory of general relativity. From graduate and postgraduate work in Pascual Jordan's relativity research group at Hamburg University, he held various posts as a lecturer and, later, as a professor before joining the Max Planck Institute for Astrophysics in Munich as a director. In 1995, he became the founding director of the newly created Max Planck Institute for Gravitational Physics in Potsdam, Germany.

Modified Newtonian dynamics (MOND) is a hypothesis that proposes a modification of Newton's law of universal gravitation to account for observed properties of galaxies. It is an alternative to the hypothesis of dark matter in terms of explaining why galaxies do not appear to obey the currently understood laws of physics.

The Cloverleaf quasar is a bright, gravitationally lensed quasar.

The Microlensing Follow-Up Network is an informal group of observers who monitor high magnification gravitational microlensing events in the Milky Way's Galactic Bulge. Its goal is to detect extrasolar planets via microlensing of the parent star by the planet. μFUN is a follow-up network - they monitor microlensing events identified by survey groups such as OGLE and Microlensing Observations in Astrophysics (MOA).

<span class="mw-page-title-main">Relativistic images</span> Images of gravitational lensing

Relativistic images are images of gravitational lensing which result due to light deflections by angles .

Robert J. Nemiroff is an Astrophysicist and Professor of Physics at Michigan Technological University. He received his Ph.D. from the University of Pennsylvania in Astronomy and Astrophysics in 1987 and his B.S. from Lehigh University in Engineering Physics in 1982. He is an active researcher with interests that include gamma-ray bursts, gravitational lensing, and cosmology, and is the cofounder and coeditor of Astronomy Picture of the Day (APOD), the home page of which receives over a million hits a day, approximately 20% of nasa.gov traffic. He is married and has one daughter.

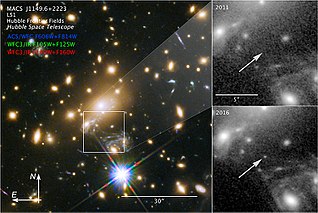
<span class="mw-page-title-main">MACS J1149 Lensed Star 1</span> Blue supergiant and second most distant star from earth detected in the constellation Leo

MACS J1149 Lensed Star 1, also known as Icarus, is a blue supergiant star observed through a gravitational lens. It is the second most distant individual star to have been detected so far, at approximately 14 billion light-years from Earth. Light from the star was emitted 4.4 billion years after the Big Bang. According to co-discoverer Patrick Kelly, the star is at least a hundred times more distant than the next-farthest non-supernova star observed, SDSS J1229+1122, and is the first magnified individual star seen.

Dr. Sun Hong Rhie was a Korean–American astrophysicist best known for her foundational contributions to the theory of gravitational microlensing, a technique for the discovery of exoplanets.

References

  1. ↑ "Arlie O. Petters, Benjamin Powell Professor & Professor of Mathematics, Physics, and Business Administration and Bass Fellow and Dean of Academic Affairs of Trinity College of Arts and Sciences and Professor of Economics". fds.duke.edu.
  2. ↑ Dhabi, NYU Abu. "Arlie Petters Selected as Provost of NYU Abu Dhabi". New York University Abu Dhabi. Retrieved 2020-07-24.
  3. ↑ "Arlie O. Petters - Mathematicians of the African Diaspora". www.math.buffalo.edu.
  4. 1 2 3 4 5 "Curriculum Vitae of Arlie O. Petters, Department of Mathematics, Duke University" (PDF). Archived from the original (PDF) on 2012-07-21. Retrieved 2012-08-10.
  5. 1 2 Claudia Dreifus. A CONVERSATION WITH: ARLIE PETTERS: A Journey to Bridge Math and the Cosmos, The New York Times , May 27, 2003.
  6. ↑ Arlie Petters at the Mathematics Genealogy Project
  7. ↑ "Profile: Arlie Petters". www.pbs.org. 24 July 2007.
  8. ↑ "About | The HistoryMakers". www.thehistorymakers.org. Archived from the original on 2020-06-21. Retrieved 2020-06-10.
  9. ↑ "Arlie Petters's Biography". The HistoryMakers.
  10. ↑ "Big Think".
  11. ↑ "Arlie Petters is one of the few tenured black mathematicians. He wants to diversify his field". The Chronicle.
  12. ↑ A. O. Petters, Ph.D. Thesis, MIT, Department of Mathematics (1991): "Singularities in Gravitational Microlensing."
  13. ↑ Section A, "Research Period 1991–2001," Curriculum Vitae of Arlie O. Petters, Department of Mathematics, Duke University
  14. 1 2 Singularity Theory and Gravitational Lensing, A. O. Petters, H. Levine, and J. Wambsganss (Birkhauser, Boston, 2001). Amazon.com
  15. ↑ "Identifying Lenses with Small-Scale Structure I. Cusp Lenses," C. Keeton, S. Gaudi, and A. O. Petters, Astrophys. J., 598, 138 (2003); arXiv : astro-ph/0210318.
  16. ↑ "Identifying Lenses with Small-Scale Structure II. Fold Lenses," C. Keeton, S. Gaudi, and A. O. Petters, Astrophys. J., 635, 35 (2005); arXiv : astro-ph/0503452.
  17. 1 2 "Gravitational Microlensing Near Caustics I: Folds," B. S. Gaudi and A. O. Petters, Astrophys. J., 574, 970 (2002); arXiv : astro-ph/0112531.
  18. 1 2 "Gravitational Microlensing Near Caustics II: Cusps," B. S. Gaudi and A. O. Petters, Astrophys. J., 580, 468 (2002); arXiv : astro-ph/0206162.
  19. 1 2 "Formalism for Testing Theories of Gravity Using Lensing by Compact Objects. I. Static, Spherically Symmetric Case," C. Keeton and A. O. Petters, Phys. Rev. D, 72, 104006 (2005); arXiv : gr-qc/0511019.
  20. ↑ "Formalism for Testing Theories of Gravity Using Lensing by Compact Objects. II. Probing Post-Post-Newtonian Metrics," C. Keeton and A. O. Petters, Phys. Rev. D 73, 044024 (2006); arXiv : gr-qc/0601053
  21. ↑ "Formalism for Testing Theories of Gravity Using Lensing by Compact Objects. III. Braneworld Gravity," C. Keeton and A. O. Petters, Phys. Rev. D 73, 104032 (2006) arXiv : gr-qc/0603061
  22. ↑ "Scientist Predict How to Detect a Fourth Dimension," Duke News Archived September 29, 2009, at the Wayback Machine
  23. ↑ "Magnification Relations for Kerr Lensing and Testing Cosmic Censorship," M. C. Werner and A. O. Petters, Phys. Rev. D 76, 064024 (2007); arXiv : gr-qc/0706.0132
  24. ↑ ""Seeking Objects Weirder than Black Holes," Duke News".
  25. ↑ "A Mathematical Theory of Stochastic Microlensing I. Random Time Delay Functions and Lensing Maps," A. O. Petters, B. Rider, and A. M. Teguia, J. Math Phys., 50, 072503 (2009); astro-ph arXiv:0807.0232v2
  26. ↑ "A Mathematical Theory of Stochastic Microlensing II. Random Images, Shear, and the Kac-Rice Formula," A. O. Petters, B. Rider, and A. M. Teguia (2008); astro-ph arXiv:0807.4984
  27. ↑ "A Universal Magnification Theorem for Higher-Order Caustic Singularities," A. B. Aazami and A. O. Petters, J. Math. Phys. 50, 032501 (2009); astro-ph arXiv:0811.3447v2
  28. ↑ "A Universal Magnification Theorem II. Caustics up to Codimension Five," A. B. Aazami and A. O. Petters, 50, 082501 (2009); math-ph arXiv:0904.2236v4
  29. ↑ National Science Foundation, Science360 News Server, April 16, 2009
  30. ↑ The Reginaldo Howard Memorial Scholarship program, Duke University
  31. ↑ Prabhu, Malavika (December 4, 2002). "Math Professor Reaches Out to Minorities". Duke Chronicle. Archived from the original on 2008-09-07. Retrieved 2018-12-08.{{cite web}}: CS1 maint: unfit URL (link)
  32. ↑ Arlie O. Petters, Curriculum Vitae--Service to Underrepresented Minority Community, Department of Mathematics, Duke University
  33. 1 2 Duke Magazine, "Star Professor" Archived August 29, 2008, at the Wayback Machine
  34. ↑ "Arlie O. Petters". services.math.duke.edu.
  35. ↑ "Home - Petters Research Institute". www.pribelize.org. Archived from the original on 2009-06-23. Retrieved 2009-03-29.
  36. ↑ 7 News Belize
  37. ↑ Computer assembly course for Belizean students by Petters Research Institute and the Belize Defence Force Archived 2009-01-07 at the Wayback Machine
  38. ↑ "Amandala Newspaper | "Belize's Leading Newspaper"". amandala.com.bz.
  39. ↑ Publications of Arlie O. Petters, Department of Mathematics, Duke University
  40. ↑ Government of Belize Official Press Release, April 28, 2010,
  41. ↑ Arlie Petters, official Curriculum Vitae, Department of Mathematics, Duke University
  42. ↑ "Alfred P. Sloan Foundation, list of past fellows". Archived from the original on May 19, 2011.
  43. ↑ "National Science Foundation, FY 98 CAREER Awardes (New Jersey)". Archived from the original on 2016-03-03. Retrieved 2018-04-06.
  44. ↑ "SIAM News".
  45. ↑ "National Academy of Sciences, African-American History Program". Archived from the original on June 29, 2010.
  46. ↑ Human Relations Associates, The Twenty-Five Greatest Scientists of African Ancestry http://hrassoc.net/blog/modules/news/article.php?storyid%3D17 . Retrieved 2009-09-22.{{cite web}}: Missing or empty |title= (help)
  47. ↑ "Hunter Headlines — Hunter College". www.hunter.cuny.edu.
  48. ↑ London Gazette, Duke Today Archived September 19, 2008, at the Wayback Machine
  49. ↑ Bates, Karl Leif (January 13, 2010). "Duke Research Blog: Putting His Name on the Map".
  50. ↑ Louisville, ky Says. "Arlie Petters wins award | Channel5Belize.com".
  51. ↑ List of Fellows of the American Mathematical Society, retrieved 2013-05-05.
  52. ↑ "Confederacion Centroamericana". Confederacion Centroamericana.
  53. ↑ "Arlie Petters". Mathematically Gifted & Black. Archived from the original on 2018-02-07.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.