Guy Terjanian is a French mathematician who has worked on algebraic number theory. He achieved his Ph.D. under Claude Chevalley in 1966, [1] and at that time published a counterexample [2] to the original form of a conjecture of Emil Artin, which suitably modified had just been proved as the Ax-Kochen theorem.
In 1977, he proved that if p is an odd prime number, and the natural numbers x, y and z satisfy , then 2p must divide x or y. [3]
In abstract algebra, an alternative algebra is an algebra in which multiplication need not be associative, only alternative. That is, one must have
In mathematics, a Diophantine equation is an equation, typically a polynomial equation in two or more unknowns with integer coefficients, such that the only solutions of interest are the integer ones. A linear Diophantine equation equates to a constant the sum of two or more monomials, each of degree one. An exponential Diophantine equation is one in which unknowns can appear in exponents.
In number theory, the law of quadratic reciprocity is a theorem about modular arithmetic that gives conditions for the solvability of quadratic equations modulo prime numbers. Due to its subtlety, it has many formulations, but the most standard statement is:
The modularity theorem states that elliptic curves over the field of rational numbers are related to modular forms. Andrew Wiles proved the modularity theorem for semistable elliptic curves, which was enough to imply Fermat's Last Theorem. Later, a series of papers by Wiles's former students Brian Conrad, Fred Diamond and Richard Taylor, culminating in a joint paper with Christophe Breuil, extended Wiles's techniques to prove the full modularity theorem in 2001.
Algebraic number theory is a branch of number theory that uses the techniques of abstract algebra to study the integers, rational numbers, and their generalizations. Number-theoretic questions are expressed in terms of properties of algebraic objects such as algebraic number fields and their rings of integers, finite fields, and function fields. These properties, such as whether a ring admits unique factorization, the behavior of ideals, and the Galois groups of fields, can resolve questions of primary importance in number theory, like the existence of solutions to Diophantine equations.
In mathematics, the Weil conjectures were highly influential proposals by André Weil (1949). They led to a successful multi-decade program to prove them, in which many leading researchers developed the framework of modern algebraic geometry and number theory.
In mathematics, the étale cohomology groups of an algebraic variety or scheme are algebraic analogues of the usual cohomology groups with finite coefficients of a topological space, introduced by Grothendieck in order to prove the Weil conjectures. Étale cohomology theory can be used to construct ℓ-adic cohomology, which is an example of a Weil cohomology theory in algebraic geometry. This has many applications, such as the proof of the Weil conjectures and the construction of representations of finite groups of Lie type.
In mathematics, there are several conjectures made by Emil Artin:
In mathematics, specifically transcendental number theory, Schanuel's conjecture is a conjecture made by Stephen Schanuel in the 1960s concerning the transcendence degree of certain field extensions of the rational numbers.
In number theory, Artin's conjecture on primitive roots states that a given integer a that is neither a square number nor −1 is a primitive root modulo infinitely many primes p. The conjecture also ascribes an asymptotic density to these primes. This conjectural density equals Artin's constant or a rational multiple thereof.
In mathematics, a field F is called quasi-algebraically closed (or C1) if every non-constant homogeneous polynomial P over F has a non-trivial zero provided the number of its variables is more than its degree. The idea of quasi-algebraically closed fields was investigated by C. C. Tsen, a student of Emmy Noether, in a 1936 paper (Tsen 1936); and later by Serge Lang in his 1951 Princeton University dissertation and in his 1952 paper (Lang 1952). The idea itself is attributed to Lang's advisor Emil Artin.
In number theory, the Chevalley–Warning theorem implies that certain polynomial equations in sufficiently many variables over a finite field have solutions. It was proved by Ewald Warning (1935) and a slightly weaker form of the theorem, known as Chevalley's theorem, was proved by Chevalley (1935). Chevalley's theorem implied Artin's and Dickson's conjecture that finite fields are quasi-algebraically closed fields.
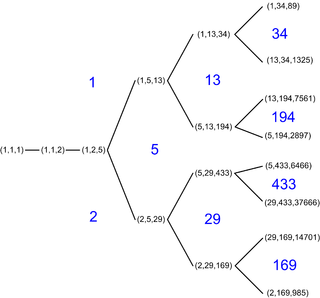
A Markov number or Markoff number is a positive integer x, y or z that is part of a solution to the Markov Diophantine equation
In mathematics, specifically in algebraic geometry and algebraic topology, the Lefschetz hyperplane theorem is a precise statement of certain relations between the shape of an algebraic variety and the shape of its subvarieties. More precisely, the theorem says that for a variety X embedded in projective space and a hyperplane section Y, the homology, cohomology, and homotopy groups of X determine those of Y. A result of this kind was first stated by Solomon Lefschetz for homology groups of complex algebraic varieties. Similar results have since been found for homotopy groups, in positive characteristic, and in other homology and cohomology theories.
In mathematics, Brauer's theorem, named for Richard Brauer, is a result on the representability of 0 by forms over certain fields in sufficiently many variables.
The Ax–Kochen theorem, named for James Ax and Simon B. Kochen, states that for each positive integer d there is a finite set Yd of prime numbers, such that if p is any prime not in Yd then every homogeneous polynomial of degree d over the p-adic numbers in at least d2 + 1 variables has a nontrivial zero.
In complex analysis, a branch of mathematics, the Gauss–Lucas theorem gives a geometric relation between the roots of a polynomial P and the roots of its derivative P'. The set of roots of a real or complex polynomial is a set of points in the complex plane. The theorem states that the roots of P' all lie within the convex hull of the roots of P, that is the smallest convex polygon containing the roots of P. When P has a single root then this convex hull is a single point and when the roots lie on a line then the convex hull is a segment of this line. The Gauss–Lucas theorem, named after Carl Friedrich Gauss and Félix Lucas, is similar in spirit to Rolle's theorem.
In number theory, Fermat's Last Theorem states that no three positive integers a, b, and c satisfy the equation an + bn = cn for any integer value of n greater than 2. The cases n = 1 and n = 2 have been known since antiquity to have infinitely many solutions.
Fermat's Last Theorem is a theorem in number theory, originally stated by Pierre de Fermat in 1637 and proved by Andrew Wiles in 1995. The statement of the theorem involves an integer exponent n larger than 2. In the centuries following the initial statement of the result and before its general proof, various proofs were devised for particular values of the exponent n. Several of these proofs are described below, including Fermat's proof in the case n = 4, which is an early example of the method of infinite descent.
Simon Bernhard Kochen is a Canadian mathematician, working in the fields of model theory, number theory and quantum mechanics.
| International | |
|---|---|
| National | |
| Academics | |
| Other | |
 | This article about a French mathematician is a stub. You can help Wikipedia by expanding it. |
