Inelastic mean free path

Last updated August 13, 2023

The inelastic mean free path (IMFP) is an index of how far an electron on average travels through a solid before losing energy.

If a monochromatic, primary beam of electrons is incident on a solid surface, the majority of incident electrons lose their energy because they interact strongly with matter, leading to plasmon excitation, electron-hole pair formation, and vibrational excitation.^[2] The intensity of the primary electrons, $I 0$ , is damped as a function of the distance, $d$ , into the solid. The intensity decay can be expressed as follows:

I(d)=I_{0}\ e^{-d\ /\lambda (E)}

where $I (d)$ is the intensity after the primary electron beam has traveled through the solid to a distance $d$ . The parameter $λ(E)$ , termed the inelastic mean free path (IMFP), is defined as the distance an electron beam can travel before its intensity decays to $1/ e$ of its initial value. (Note that this is equation is closely related to the Beer–Lambert law.)

The inelastic mean free path of electrons can roughly be described by a universal curve that is the same for all materials.^[1]^[3]

The knowledge of the IMFP is indispensable for several electron spectroscopy and microscopy measurements.^[4]

Applications of the IMFP in XPS

Following,^[5] the IMFP is employed to calculate the effective attenuation length (EAL), the mean escape depth (MED) and the information depth (ID). Besides, one can utilize the IMFP to make matrix corrections for the relative sensitivity factor in quantitative surface analysis. Moreover, the IMFP is an important parameter in Monte Carlo simulations of photoelectron transport in matter.

Calculations of the IMFP

Calculations of the IMFP are mostly based on the algorithm (full Penn algorithm, FPA) developed by Penn,^[6] experimental optical constants or calculated optical data (for compounds).^[5] The FPA considers an inelastic scattering event and the dependence of the energy-loss function (EFL) on momentum transfer which describes the probability for inelastic scattering as a function of momentum transfer.^[5]

Experimental measurements of the IMFP

To measure the IMFP, one well known method is elastic-peak electron spectroscopy (EPES).^[5]^[7] This method measures the intensity of elastically backscattered electrons with a certain energy from a sample material in a certain direction. Applying a similar technique to materials whose IMFP is known, the measurements are compared with the results from the Monte Carlo simulations under the same conditions. Thus, one obtains the IMFP of a certain material in a certain energy spectrum. EPES measurements show a root-mean-square (RMS) difference between 12% and 17% from the theoretical expected values.^[5] Calculated and experimental results show higher agreement for higher energies.^[5]

For electron energies in the range 30 keV – 1 MeV, IMFP can be directly measured by electron energy loss spectroscopy inside a transmission electron microscope, provided the sample thickness is known. Such measurements reveal that IMFP in elemental solids is not a smooth, but an oscillatory function of the atomic number.^[8]

For energies below 100 eV, IMFP can be evaluated in high-energy secondary electron yield (SEY) experiments.^[9] Therefore, the SEY for an arbitrary incident energy between 0.1 keV-10 keV is analyzed. According to these experiments, a Monte Carlo model can be used to simulate the SEYs and determine the IMFP below 100 eV.

Predictive formulas

Using the dielectric formalism,^[4] the IMFP $\lambda ^{-1}$ can be calculated by solving the following integral:

\lambda ^{-1}={\frac {1}{\pi E}}\int _{\omega _{\mathrm {min} }}^{\omega _{\mathrm {max} }}\,d\omega \int _{k_{-}}^{k_{+}}\mathrm {Im} \left({\frac {-1}{\epsilon (k,\omega )}}\right)\,{\frac {dk}{k}}

(1)

with the minimum (maximum) energy loss $\omega _{\mathrm {min} }$ ( $\omega _{\mathrm {max} }$ ), the dielectric function $\epsilon$ , the energy loss function (ELF) $\mathrm {Im} ({\frac {-1}{\epsilon (k,\omega )}})$ and the smallest and largest momentum transfer $k_{\pm }={\sqrt {2E}}\pm {\sqrt {2(E-\omega )}}$ . In general, solving this integral is quite challenging and only applies for energies above 100 eV. Thus, (semi)empirical formulas were introduced to determine the IMFP.

A first approach is to calculate the IMFP by an approximate form of the relativistic Bethe equation for inelastic scattering of electrons in matter.^[5]^[10] Equation 2 holds for energies between 50 eV and 200 keV:

\lambda ^{-1}={\frac {\alpha (E)E}{E_{p}^{2}(\beta \ln {(\gamma \alpha (E)E)-(C/E)+(D/E)^{2}})}}

(2)

with

\alpha (E)={\frac {1+{\frac {E}{2m_{e}c^{2}}}}{1+{\frac {E}{m_{e}c^{2}}}}}={\frac {1+{\frac {E}{1021}}999.8}{(1+{\frac {E}{510}}998.9)^{2}}}

and

E_{p}=28.816\left({\frac {N_{\nu }\rho }{M}}\right)^{0.5}(\mathrm {eV} )

and the electron energy $E$ in eV above the Fermi level (conductors) or above the bottom of the conduction band (non-conductors). $m_{e}$ is the electron mass, $c$ the vacuum velocity of light, $N_{\nu }$ is the number of valence electrons per atom or molecule, $\rho$ describes the density (in $\mathrm {\frac {g}{cm^{3}}}$ ), $M$ is the atomic or molecular weight and $\beta$ , $\gamma$ , $C$ and $D$ are parameters determined in the following. Equation 2 calculates the IMFP and its dependence on the electron energy in condensed matter.

Equation 2 was further developed^[5]^[11] to find the relations for the parameters $\beta$ , $\gamma$ , $C$ and $D$ for energies between 50 eV and 2 keV:

\beta =-1.0+9.44/(E_{p}^{2}+E_{g}^{2})^{0.5}+0.69\rho ^{0.1}(\mathrm {eV^{-1}} \mathrm {nm^{-1}} )

(3)

$\gamma =0.191\rho ^{-0.5}(\mathrm {eV^{-1}} )$
$C=19.7-9.1U(\mathrm {nm^{-1}} )$
$D=534-208U(\mathrm {eV} \mathrm {nm^{-1}} )$
$U={\frac {N_{\nu }\rho }{M}}=(E_{p}/28.816)^{2}$

Here, the bandgap energy $E_{g}$ is given in eV. Equation 2 an 3 are also known as the TTP-2M equations and are in general applicable for energies between 50 eV and 200 keV. Neglecting a few materials (diamond, graphite, Cs, cubic-BN and hexagonal BN) that are not following these equations (due to deviations in $\beta$ ), the TTP-2M equations show precise agreement with the measurements.

Another approach based on Equation 2 to determine the IMFP is the S1 formula.^[5]^[12] This formula can be applied for energies between 100 eV and 10 keV:

\lambda ^{-1}={\frac {(4+0.44Z^{0.5}+0.104E^{0.872})a^{1.7}}{Z^{0.3}(1-W)}}

with the atomic number $Z$ (average atomic number for a compound), $W=0.06H$ or $W=0.02E_{g}$ ( $H$ is the heat of formation of a compound in eV per atom) and the average atomic spacing $a$ :

a^{3}={\frac {10^{21}M}{\rho N_{A}(g+h)}}(\mathrm {nm^{3}} )

with the Avogadro constant $N_{A}$ and the stoichiometric coefficients $g$ and $h$ describing binary compounds $G_{g}H_{h}$ . In this case, the atomic number becomes

Z={\frac {gZ_{g}+hZ_{h}}{g+h}}

with the atomic numbers $Z_{g}$ and $Z_{h}$ of the two constituents. This S1 formula shows higher agreement with measurements compared to Equation 2 .^[5]

Calculating the IMFP with either the TTP-2M formula or the S1 formula requires different knowledge of some parameters.^[5] Applying the TTP-2M formula one needs to know $M$ , $\rho$ and $N_{\nu }$ for conducting materials (and also $E_{g}$ for non-conductors). Employing S1 formula, knowledge of the atomic number $Z$ (average atomic number for compounds), $M$ and $\rho$ is required for conductors. If non-conducting materials are considered, one also needs to know either $E_{g}$ or $H$ .

An analytical formula for calculating the IMFP down to 50 eV was proposed in 2021.^[4] Therefore, an exponential term was added to an analytical formula already derived from 1 that was applicible for energies down to 500 eV:

\lambda ^{-1}={\frac {1}{2\pi a_{0}E}}\left(A\ln {\frac {4E}{I}}-{\frac {7C}{4E}}(1-\exp {(-AE/2C)})\right)

(4)

For relativistic electrons it holds:

\lambda ^{-1}={\frac {1}{\pi a_{0}v^{2}}}\left(A\ln {\frac {2v^{2}}{I}}-{\frac {7C}{2v^{2}}}(1-\exp {(-Av^{2}/4C)})\right)

(5)

with the electron velocity $v$ , $v^{2}=c^{2}\tau (\tau +2)/(\tau +1)^{2}$ and $\tau =E/c^{2}$ . $c$ denotes the velocity of light. $\lambda$ and $a_{0}$ are given in nanometers. The constants in 4 and 5 are defined as following:

$A=\int _{0}^{\infty }\mathrm {Im} \left({\frac {-1}{\epsilon (\omega )}}\right)\,d\omega$
$A\ln {(I)}=\int _{0}^{\infty }\mathrm {Im} \left({\frac {-1}{\epsilon (\omega )}}\right)\ln {(\omega )}\,d\omega$
$C=\int _{0}^{\infty }\mathrm {Im} \left({\frac {-1}{\epsilon (\omega )}}\right)\omega \,d\omega$

IMFP data

IMFP data can be collected from the National Institute of Standards and Technology (NIST) Electron Inelastic-Mean-Free-Path Database^[13] or the NIST Database for the Simulation of Electron Spectra for Surface Analysis (SESSA).^[14] The data contains IMFPs determined by EPES for energies below 2 keV. Otherwise, IMFPs can be determined from the TPP-2M or the S1 formula.^[5]

Related Research Articles

A centripetal force is a force that makes a body follow a curved path. The direction of the centripetal force is always orthogonal to the motion of the body and towards the fixed point of the instantaneous center of curvature of the path. Isaac Newton described it as "a force by which bodies are drawn or impelled, or in any way tend, towards a point as to a centre". In the theory of Newtonian mechanics, gravity provides the centripetal force causing astronomical orbits.

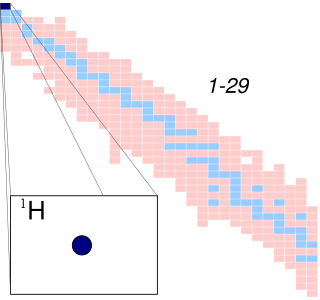
A hydrogen atom is an atom of the chemical element hydrogen. The electrically neutral atom contains a single positively charged proton and a single negatively charged electron bound to the nucleus by the Coulomb force. Atomic hydrogen constitutes about 75% of the baryonic mass of the universe.

<span class="mw-page-title-main">Bremsstrahlung</span> Electromagnetic radiation due to deceleration of charged particles

In particle physics, bremsstrahlung is electromagnetic radiation produced by the deceleration of a charged particle when deflected by another charged particle, typically an electron by an atomic nucleus. The moving particle loses kinetic energy, which is converted into radiation, thus satisfying the law of conservation of energy. The term is also used to refer to the process of producing the radiation. Bremsstrahlung has a continuous spectrum, which becomes more intense and whose peak intensity shifts toward higher frequencies as the change of the energy of the decelerated particles increases.

<span class="mw-page-title-main">X-ray photoelectron spectroscopy</span> Spectroscopic technique

X-ray photoelectron spectroscopy (XPS) is a surface-sensitive quantitative spectroscopic technique based on the photoelectric effect that can identify the elements that exist within a material or are covering its surface, as well as their chemical state, and the overall electronic structure and density of the electronic states in the material. XPS is a powerful measurement technique because it not only shows what elements are present, but also what other elements they are bonded to. The technique can be used in line profiling of the elemental composition across the surface, or in depth profiling when paired with ion-beam etching. It is often applied to study chemical processes in the materials in their as-received state or after cleavage, scraping, exposure to heat, reactive gasses or solutions, ultraviolet light, or during ion implantation.

In physics, screening is the damping of electric fields caused by the presence of mobile charge carriers. It is an important part of the behavior of charge-carrying fluids, such as ionized gases, electrolytes, and charge carriers in electronic conductors . In a fluid, with a given permittivity $ε$ , composed of electrically charged constituent particles, each pair of particles interact through the Coulomb force as

Acoustic impedance and specific acoustic impedance are measures of the opposition that a system presents to the acoustic flow resulting from an acoustic pressure applied to the system. The SI unit of acoustic impedance is the pascal-second per cubic metre, or in the MKS system the rayl per square metre, while that of specific acoustic impedance is the pascal-second per metre, or in the MKS system the rayl. There is a close analogy with electrical impedance, which measures the opposition that a system presents to the electric current resulting from a voltage applied to the system.

<span class="mw-page-title-main">Equipartition theorem</span> Theorem in classical statistical mechanics

In classical statistical mechanics, the equipartition theorem relates the temperature of a system to its average energies. The equipartition theorem is also known as the law of equipartition, equipartition of energy, or simply equipartition. The original idea of equipartition was that, in thermal equilibrium, energy is shared equally among all of its various forms; for example, the average kinetic energy per degree of freedom in translational motion of a molecule should equal that in rotational motion.

Plasma oscillations, also known as Langmuir waves, are rapid oscillations of the electron density in conducting media such as plasmas or metals in the ultraviolet region. The oscillations can be described as an instability in the dielectric function of a free electron gas. The frequency depends only weakly on the wavelength of the oscillation. The quasiparticle resulting from the quantization of these oscillations is the plasmon.

In mesoscopic physics, ballistic conduction is the unimpeded flow of charge carriers, or energy-carrying particles, over relatively long distances in a material. In general, the resistivity of a material exists because an electron, while moving inside a medium, is scattered by impurities, defects, thermal fluctuations of ions in a crystalline solid, or, generally, by any freely-moving atom/molecule composing a gas or liquid. Without scattering, electrons simply obey Newton's second law of motion at non-relativistic speeds.

A hydrogen-like atom (or hydrogenic atom) is any atom or ion with a single valence electron. These atoms are isoelectronic with hydrogen. Examples of hydrogen-like atoms include, but are not limited to, hydrogen itself, all alkali metals such as Rb and Cs, singly ionized alkaline earth metals such as Ca⁺ and Sr⁺ and other ions such as He⁺, Li²⁺, and Be³⁺ and isotopes of any of the above. A hydrogen-like atom includes a positively charged core consisting of the atomic nucleus and any core electrons as well as a single valence electron. Because helium is common in the universe, the spectroscopy of singly ionized helium is important in EUV astronomy, for example, of DO white dwarf stars.

In mathematics – specifically, in stochastic analysis – an Itô diffusion is a solution to a specific type of stochastic differential equation. That equation is similar to the Langevin equation used in physics to describe the Brownian motion of a particle subjected to a potential in a viscous fluid. Itô diffusions are named after the Japanese mathematician Kiyosi Itô.

The linear attenuation coefficient, attenuation coefficient, or narrow-beam attenuation coefficient characterizes how easily a volume of material can be penetrated by a beam of light, sound, particles, or other energy or matter. A coefficient value that is large represents a beam becoming 'attenuated' as it passes through a given medium, while a small value represents that the medium had little effect on loss. The SI unit of attenuation coefficient is the reciprocal metre (m⁻¹). Extinction coefficient is another term for this quantity, often used in meteorology and climatology. Most commonly, the quantity measures the exponential decay of intensity, that is, the value of downward e-folding distance of the original intensity as the energy of the intensity passes through a unit thickness of material, so that an attenuation coefficient of 1 m⁻¹ means that after passing through 1 metre, the radiation will be reduced by a factor of e, and for material with a coefficient of 2 m⁻¹, it will be reduced twice by e, or e². Other measures may use a different factor than e, such as the decadic attenuation coefficient below. The broad-beam attenuation coefficient counts forward-scattered radiation as transmitted rather than attenuated, and is more applicable to radiation shielding.

In fluid dynamics, Airy wave theory gives a linearised description of the propagation of gravity waves on the surface of a homogeneous fluid layer. The theory assumes that the fluid layer has a uniform mean depth, and that the fluid flow is inviscid, incompressible and irrotational. This theory was first published, in correct form, by George Biddell Airy in the 19th century.

Defect types include atom vacancies, adatoms, steps, and kinks that occur most frequently at surfaces due to the finite material size causing crystal discontinuity. What all types of defects have in common, whether surface or bulk defects, is that they produce dangling bonds that have specific electron energy levels different from those of the bulk. This difference occurs because these states cannot be described with periodic Bloch waves due to the change in electron potential energy caused by the missing ion cores just outside the surface. Hence, these are localized states that require separate solutions to the Schrödinger equation so that electron energies can be properly described. The break in periodicity results in a decrease in conductivity due to defect scattering.

An electric dipole transition is the dominant effect of an interaction of an electron in an atom with the electromagnetic field.

The Maxwell–Bloch equations, also called the optical Bloch equations describe the dynamics of a two-state quantum system interacting with the electromagnetic mode of an optical resonator. They are analogous to the Bloch equations which describe the motion of the nuclear magnetic moment in an electromagnetic field. The equations can be derived either semiclassically or with the field fully quantized when certain approximations are made.

Heat transfer physics describes the kinetics of energy storage, transport, and energy transformation by principal energy carriers: phonons, electrons, fluid particles, and photons. Heat is energy stored in temperature-dependent motion of particles including electrons, atomic nuclei, individual atoms, and molecules. Heat is transferred to and from matter by the principal energy carriers. The state of energy stored within matter, or transported by the carriers, is described by a combination of classical and quantum statistical mechanics. The energy is different made (converted) among various carriers. The heat transfer processes are governed by the rates at which various related physical phenomena occur, such as the rate of particle collisions in classical mechanics. These various states and kinetics determine the heat transfer, i.e., the net rate of energy storage or transport. Governing these process from the atomic level to macroscale are the laws of thermodynamics, including conservation of energy.

Quantum stochastic calculus is a generalization of stochastic calculus to noncommuting variables. The tools provided by quantum stochastic calculus are of great use for modeling the random evolution of systems undergoing measurement, as in quantum trajectories. Just as the Lindblad master equation provides a quantum generalization to the Fokker–Planck equation, quantum stochastic calculus allows for the derivation of quantum stochastic differential equations (QSDE) that are analogous to classical Langevin equations.

The Bohr–Sommerfeld model was an extension of the Bohr model to allow elliptical orbits of electrons around an atomic nucleus. Bohr–Sommerfeld theory is named after Danish physicist Niels Bohr and German physicist Arnold Sommerfeld. Sommerfeld argued that if electronic orbits could be elliptical instead of circular, the energy of the electron would be the same, except in the presence of a magnetic field, introducing what is now known as quantum degeneracy.

In quantum probability, the Belavkin equation, also known as Belavkin-Schrödinger equation, quantum filtering equation, stochastic master equation, is a quantum stochastic differential equation describing the dynamics of a quantum system undergoing observation in continuous time. It was derived and henceforth studied by Viacheslav Belavkin in 1988.

References

1 2 Seah, M. P.; Dench, W. A. (1979), "Quantitative electron spectroscopy of surfaces: A standard data base for electron inelastic mean free paths in solids", Surface and Interface Analysis, 1: 2–11, doi:10.1002/sia.740010103
↑ Egerton, R. F. (1996) Electron energy-loss spectroscopy in the electron microscope (Second Edition, Plenum Press, NY) ISBN 0-306-45223-5
↑ Werner, Wolfgang S. M. (2001), "Review of electron transport in solids", Surface and Interface Analysis, 31 (3): 141–176, doi:10.1002/sia.973, S2CID 95869994
1 2 3 Le, Dai-Nam; Nguyen-Truong, Hieu T. (2021). "Analytical Formula for the Electron Inelastic Mean Free Path". The Journal of Physical Chemistry C. 125 (34): 18946–18951. doi:10.1021/acs.jpcc.1c05212. S2CID 238685492.
1 2 3 4 5 6 7 8 9 10 11 12 Powell, Cedric J. (2020). "Practical guide for inelastic mean free paths, effective attenuation lengths, mean escape depths, and information depths in x-ray photoelectron spectroscopy". Journal of Vacuum Science & Technology A. 38 (23209).
↑ Penn, D. R. (1987). "Electron mean-free-path calculations using a model dielectric function". Phys. Rev. B. 35 (482): 482–486. Bibcode:1987PhRvB..35..482P. doi:10.1103/PhysRevB.35.482. PMID 9941428.
↑ Powell, C. J.; Jablonski, A. (1999). "Evaluation of Calculated and Measured Electron Inelastic Mean Free Paths Near Solid Surfaces". J. Phys. Chem. Ref. Data. 28 (1): 19–28. Bibcode:1999JPCRD..28...19P. doi:10.1063/1.556035.
↑ Iakoubovskii, Konstantin; Mitsuishi, Kazutaka; Nakayama, Yoshiko; Furuya, Kazuo (2008). "Mean free path of inelastic electron scattering in elemental solids and oxides using transmission electron microscopy: Atomic number dependent oscillatory behavior". Physical Review B. 77 (10): 104102. Bibcode:2008PhRvB..77j4102I. doi:10.1103/PhysRevB.77.104102.
↑ Ridzel, Olga Yu.; Astasauskas, Vytautas; Werner, Wolfgang S. M. (2020). "Low energy electron inelastic mean free path values determined from analysis of secondary electron yields in the incident energy range of 0.1–10 keV". Journal of Electron Spectroscopy and Related Phenomena. 241: 146824. doi: 10.1016/j.elspec.2019.02.003 . S2CID 104369752.
↑ Shinotsuka, H.; Tanuma, S.; Powell, C. J.; Penn, D. R. (2015). "Calculations of electron inelastic mean free paths. X. Data for 41 elemental solids over the 50 eV to 200 keV range with the relativistic full Penn algorithm". Surface and Interface Analysis. 47 (9): 871. doi:10.1002/sia.5789. S2CID 93935648.
↑ Tanuma, S.; Powell, C. J.; Penn, D. R. (1994). "Calculations of electron inelastic mean free paths. V. Data for 14 organic compounds over the 50–2000 eV range". Surface and Interface Analysis. 21 (3): 165-176. doi:10.1002/sia.740210302.
↑ Seah, M. P. (2012). "An accurate and simple universal curve for the energy-dependent electron inelastic mean free path". Surface and Interface Analysis. 44 (4): 497. doi:10.1002/sia.4816. S2CID 93786577.
↑ Powell, C. J.; Jablonski, A. (2000). "NIST Electron Inelastic-Mean-Free-Path Database". NIST Standard Reference Database 71.
↑ Werner, W. S. M.; Smekal, W.; Powell, C. J. (2018). "NIST Database for the Simulation of Electron Spectra for Surface Analysis, Version 2.1". NIST NSRDS 100.

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[Seah-1] 1 2 Seah, M. P.; Dench, W. A. (1979), "Quantitative electron spectroscopy of surfaces: A standard data base for electron inelastic mean free paths in solids", Surface and Interface Analysis, 1: 2–11, doi:10.1002/sia.740010103

[2] Egerton, R. F. (1996) Electron energy-loss spectroscopy in the electron microscope (Second Edition, Plenum Press, NY) ISBN 0-306-45223-5

[Werner-3] Werner, Wolfgang S. M. (2001), "Review of electron transport in solids", Surface and Interface Analysis, 31 (3): 141–176, doi:10.1002/sia.973, S2CID 95869994

[Le,_Nguyen-Truong-4] 1 2 3 Le, Dai-Nam; Nguyen-Truong, Hieu T. (2021). "Analytical Formula for the Electron Inelastic Mean Free Path". The Journal of Physical Chemistry C. 125 (34): 18946–18951. doi:10.1021/acs.jpcc.1c05212. S2CID 238685492.

[Powell-5] 1 2 3 4 5 6 7 8 9 10 11 12 Powell, Cedric J. (2020). "Practical guide for inelastic mean free paths, effective attenuation lengths, mean escape depths, and information depths in x-ray photoelectron spectroscopy". Journal of Vacuum Science & Technology A. 38 (23209).

[Penn-6] Penn, D. R. (1987). "Electron mean-free-path calculations using a model dielectric function". Phys. Rev. B. 35 (482): 482–486. Bibcode:1987PhRvB..35..482P. doi:10.1103/PhysRevB.35.482. PMID 9941428.

[7] Powell, C. J.; Jablonski, A. (1999). "Evaluation of Calculated and Measured Electron Inelastic Mean Free Paths Near Solid Surfaces". J. Phys. Chem. Ref. Data. 28 (1): 19–28. Bibcode:1999JPCRD..28...19P. doi:10.1063/1.556035.

[8] Iakoubovskii, Konstantin; Mitsuishi, Kazutaka; Nakayama, Yoshiko; Furuya, Kazuo (2008). "Mean free path of inelastic electron scattering in elemental solids and oxides using transmission electron microscopy: Atomic number dependent oscillatory behavior". Physical Review B. 77 (10): 104102. Bibcode:2008PhRvB..77j4102I. doi:10.1103/PhysRevB.77.104102.

[9] Ridzel, Olga Yu.; Astasauskas, Vytautas; Werner, Wolfgang S. M. (2020). "Low energy electron inelastic mean free path values determined from analysis of secondary electron yields in the incident energy range of 0.1–10 keV". Journal of Electron Spectroscopy and Related Phenomena. 241: 146824. doi: 10.1016/j.elspec.2019.02.003 . S2CID 104369752.

[10] Shinotsuka, H.; Tanuma, S.; Powell, C. J.; Penn, D. R. (2015). "Calculations of electron inelastic mean free paths. X. Data for 41 elemental solids over the 50 eV to 200 keV range with the relativistic full Penn algorithm". Surface and Interface Analysis. 47 (9): 871. doi:10.1002/sia.5789. S2CID 93935648.

[11] Tanuma, S.; Powell, C. J.; Penn, D. R. (1994). "Calculations of electron inelastic mean free paths. V. Data for 14 organic compounds over the 50–2000 eV range". Surface and Interface Analysis. 21 (3): 165-176. doi:10.1002/sia.740210302.

[12] Seah, M. P. (2012). "An accurate and simple universal curve for the energy-dependent electron inelastic mean free path". Surface and Interface Analysis. 44 (4): 497. doi:10.1002/sia.4816. S2CID 93786577.

[13] Powell, C. J.; Jablonski, A. (2000). "NIST Electron Inelastic-Mean-Free-Path Database". NIST Standard Reference Database 71.

[14] Werner, W. S. M.; Smekal, W.; Powell, C. J. (2018). "NIST Database for the Simulation of Electron Spectra for Surface Analysis, Version 2.1". NIST NSRDS 100.

[2]

[1]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[13]

[14]