The Riemann zeta function or Euler–Riemann zeta function, denoted by the Greek letter ζ (zeta), is a mathematical function of a complex variable defined as
Goldbach's conjecture is one of the oldest and best-known unsolved problems in number theory and all of mathematics. It states that every even natural number greater than 2 is the sum of two prime numbers.
In number theory, Goldbach's weak conjecture, also known as the odd Goldbach conjecture, the ternary Goldbach problem, or the 3-primes problem, states that
The Riemann hypothesis is one of the most important conjectures in mathematics. It is a statement about the zeros of the Riemann zeta function. Various geometrical and arithmetical objects can be described by so-called global L-functions, which are formally similar to the Riemann zeta-function. One can then ask the same question about the zeros of these L-functions, yielding various generalizations of the Riemann hypothesis. Many mathematicians believe these generalizations of the Riemann hypothesis to be true. The only cases of these conjectures which have been proven occur in the algebraic function field case.
In mathematics, the n-th harmonic number is the sum of the reciprocals of the first n natural numbers:
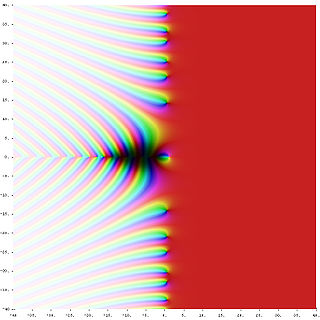
In mathematics, analytic number theory is a branch of number theory that uses methods from mathematical analysis to solve problems about the integers. It is often said to have begun with Peter Gustav Lejeune Dirichlet's 1837 introduction of Dirichlet L-functions to give the first proof of Dirichlet's theorem on arithmetic progressions. It is well known for its results on prime numbers and additive number theory.
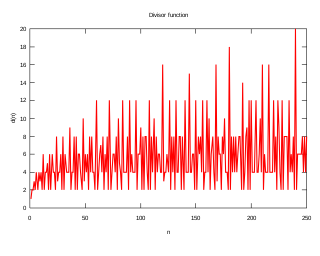
In mathematics, and specifically in number theory, a divisor function is an arithmetic function related to the divisors of an integer. When referred to as the divisor function, it counts the number of divisors of an integer. It appears in a number of remarkable identities, including relationships on the Riemann zeta function and the Eisenstein series of modular forms. Divisor functions were studied by Ramanujan, who gave a number of important congruences and identities; these are treated separately in the article Ramanujan's sum.
In number theory, the Mertens function is defined for all positive integers n as
In mathematics, the Lindelöf hypothesis is a conjecture by Finnish mathematician Ernst Leonard Lindelöf about the rate of growth of the Riemann zeta function on the critical line. This hypothesis is implied by the Riemann hypothesis. It says that for any ε > 0,
In mathematics, a superabundant number is a certain kind of natural number. A natural number n is called superabundant precisely when, for all m < n:
In number theory, a colossally abundant number is a natural number that, in a particular, rigorous sense, has many divisors. Particularly, it is defined by a ratio between the sum of an integer's divisors and that integer raised to a power higher than one. For any such exponent, whichever integer has the highest ratio is a colossally abundant number. It is a stronger restriction than that of a superabundant number, but not strictly stronger than that of an abundant number.
In mathematics, the explicit formulae for L-functions are relations between sums over the complex number zeroes of an L-function and sums over prime powers, introduced by Riemann (1859) for the Riemann zeta function. Such explicit formulae have been applied also to questions on bounding the discriminant of an algebraic number field, and the conductor of a number field.
In mathematics, a natural number a is a unitary divisor of a number b if a is a divisor of b and if a and are coprime, having no common factor other than 1. Equivalently, a divisor a of b is a unitary divisor if and only if every prime factor of a has the same multiplicity in a as it has in b.
At the 1912 International Congress of Mathematicians, Edmund Landau listed four basic problems about prime numbers. These problems were characterised in his speech as "unattackable at the present state of mathematics" and are now known as Landau's problems. They are as follows:
- Goldbach's conjecture: Can every even integer greater than 2 be written as the sum of two primes?
- Twin prime conjecture: Are there infinitely many primes p such that p + 2 is prime?
- Legendre's conjecture: Does there always exist at least one prime between consecutive perfect squares?
- Are there infinitely many primes p such that p − 1 is a perfect square? In other words: Are there infinitely many primes of the form n2 + 1?
In number theory, the divisor summatory function is a function that is a sum over the divisor function. It frequently occurs in the study of the asymptotic behaviour of the Riemann zeta function. The various studies of the behaviour of the divisor function are sometimes called divisor problems.
Helmut Maier is a German mathematician and professor at the University of Ulm, Germany. He is known for his contributions in analytic number theory and mathematical analysis and particularly for the so-called Maier's matrix method as well as Maier's theorem for primes in short intervals. He has also done important work in exponential sums and trigonometric sums over special sets of integers and the Riemann zeta function.
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part 1/2. Many consider it to be the most important unsolved problem in pure mathematics. It is of great interest in number theory because it implies results about the distribution of prime numbers. It was proposed by Bernhard Riemann, after whom it is named.
Anatoly Alexeyevich Karatsuba was a Russian mathematician working in the field of analytic number theory, p-adic numbers and Dirichlet series.
In mathematics, specifically in number theory, the extremal orders of an arithmetic function are best possible bounds of the given arithmetic function. Specifically, if f(n) is an arithmetic function and m(n) is a non-decreasing function that is ultimately positive and
Cameron Leigh Stewart FRSC is a Canadian mathematician. He is a professor of pure mathematics at the University of Waterloo.
