Related Research Articles

In mathematics, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on the order in which they are written. That is, the group operation is commutative. With addition as an operation, the integers and the real numbers form abelian groups, and the concept of an abelian group may be viewed as a generalization of these examples. Abelian groups are named after early 19th century mathematician Niels Henrik Abel.

In mathematics, specifically group theory, given a prime number p, a p-group is a group in which the order of every element is a power of p. That is, for each element g of a p-group G, there exists a nonnegative integer n such that the product of pn copies of g, and not fewer, is equal to the identity element. The orders of different elements may be different powers of p.
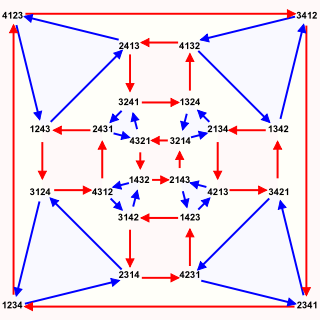
In abstract algebra, the symmetric group defined over any set is the group whose elements are all the bijections from the set to itself, and whose group operation is the composition of functions. In particular, the finite symmetric group defined over a finite set of symbols consists of the permutations that can be performed on the symbols. Since there are such permutation operations, the order of the symmetric group is .

In mathematics, a simple group is a nontrivial group whose only normal subgroups are the trivial group and the group itself. A group that is not simple can be broken into two smaller groups, namely a nontrivial normal subgroup and the corresponding quotient group. This process can be repeated, and for finite groups one eventually arrives at uniquely determined simple groups, by the Jordan–Hölder theorem.

In group theory, a branch of abstract algebra in pure mathematics, a cyclic group or monogenous group is a group, denoted Cn, that is generated by a single element. That is, it is a set of invertible elements with a single associative binary operation, and it contains an element g such that every other element of the group may be obtained by repeatedly applying the group operation to g or its inverse. Each element can be written as an integer power of g in multiplicative notation, or as an integer multiple of g in additive notation. This element g is called a generator of the group.

In mathematics, specifically in the field of finite group theory, the Sylow theorems are a collection of theorems named after the Norwegian mathematician Peter Ludwig Sylow that give detailed information about the number of subgroups of fixed order that a given finite group contains. The Sylow theorems form a fundamental part of finite group theory and have very important applications in the classification of finite simple groups.

In mathematics, the free groupFS over a given set S consists of all words that can be built from members of S, considering two words to be different unless their equality follows from the group axioms. The members of S are called generators of FS, and the number of generators is the rank of the free group. An arbitrary group G is called free if it is isomorphic to FS for some subset S of G, that is, if there is a subset S of G such that every element of G can be written in exactly one way as a product of finitely many elements of S and their inverses.

In mathematics, specifically group theory, a nilpotent groupG is a group that has an upper central series that terminates with G. Equivalently, its central series is of finite length or its lower central series terminates with {1}.

A group is a set together with an associative operation which admits an identity element and such that every element has an inverse.
In mathematics, an amenable group is a locally compact topological group G carrying a kind of averaging operation on bounded functions that is invariant under translation by group elements. The original definition, in terms of a finitely additive measure on subsets of G, was introduced by John von Neumann in 1929 under the German name "messbar" in response to the Banach–Tarski paradox. In 1949 Mahlon M. Day introduced the English translation "amenable", apparently as a pun on "mean".
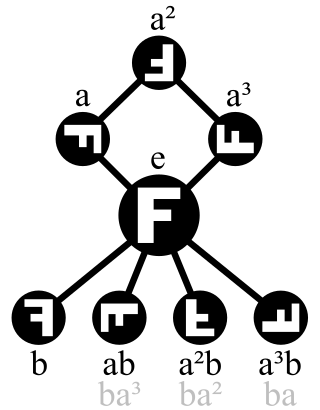
In algebra, a finitely generated group is a group G that has some finite generating set S so that every element of G can be written as the combination of finitely many elements of S and of inverses of such elements.
In mathematics, the Feit–Thompson theorem, or odd order theorem, states that every finite group of odd order is solvable. It was proved by Walter Feit and John Griggs Thompson.
In group theory, a branch of mathematics, a torsion group or a periodic group is a group in which every element has finite order. The exponent of such a group, if it exists, is the least common multiple of the orders of the elements.
In mathematics, George Glauberman's Z* theorem is stated as follows:
Z* theorem: Let G be a finite group, with O(G) being its maximal normal subgroup of odd order. If T is a Sylow 2-subgroup of G containing an involution not conjugate in G to any other element of T, then the involution lies in Z*(G), which is the inverse image in G of the center of G/O(G).
In mathematics, especially in the area of algebra known as group theory, the term Z-group refers to a number of distinct types of groups:
In abstract algebra, the focal subgroup theorem describes the fusion of elements in a Sylow subgroup of a finite group. The focal subgroup theorem was introduced in and is the "first major application of the transfer" according to. The focal subgroup theorem relates the ideas of transfer and fusion such as described in. Various applications of these ideas include local criteria for p-nilpotence and various non-simplicity criteria focussing on showing that a finite group has a normal subgroup of index p.
In mathematics, the height of an element g of an abelian group A is an invariant that captures its divisibility properties: it is the largest natural number N such that the equation Nx = g has a solution x ∈ A, or the symbol ∞ if there is no such N. The p-height considers only divisibility properties by the powers of a fixed prime number p. The notion of height admits a refinement so that the p-height becomes an ordinal number. Height plays an important role in Prüfer theorems and also in Ulm's theorem, which describes the classification of certain infinite abelian groups in terms of their Ulm factors or Ulm invariants.

Sergei Nikolaevich Chernikov was a Russian mathematician who contributed significantly to the development of infinite group theory and linear inequalities.
References
- ↑ Dixon, M. R.; Kirichenko, V. V.; Kurdachenko, L. A.; Otal, J.; Semko, N. N.; Shemetkov, L. A.; Subbotin, I. Ya. (2012). "S. N. Chernikov and the development of infinite group theory". Algebra and Discrete Mathematics. 13 (2): 169–208.
- ↑ Curtis, Charles; Reiner, Irving (1962), Representation Theory of Finite Groups and Associated Algebras, John Wiley & Sons, pp. 256–262
- Dixon, Martyn R. (1994), Sylow theory, formations and Fitting classes in locally finite groups, Series in Algebra, vol. 2, River Edge, NJ: World Scientific Publishing Co. Inc., ISBN 978-981-02-1795-2, MR 1313499
- Robinson, Derek John Scott (1996), A course in the theory of groups, Berlin, New York: Springer-Verlag, ISBN 978-0-387-94461-6