Related Research Articles

An algebraic number is a number that is a root of a non-zero polynomial in one variable with integer coefficients. For example, the golden ratio, , is an algebraic number, because it is a root of the polynomial x2 − x − 1. That is, it is a value for x for which the polynomial evaluates to zero. As another example, the complex number is algebraic because it is a root of x4 + 4.

Number theory is a branch of pure mathematics devoted primarily to the study of the integers and integer-valued functions. German mathematician Carl Friedrich Gauss (1777–1855) said, "Mathematics is the queen of the sciences—and number theory is the queen of mathematics." Number theorists study prime numbers as well as the properties of mathematical objects made out of integers or defined as generalizations of the integers.
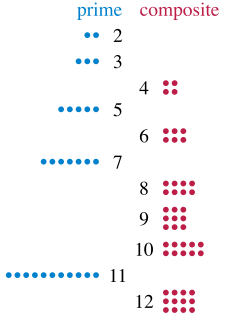
A prime number is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only ways of writing it as a product, 1 × 5 or 5 × 1, involve 5 itself. However, 4 is composite because it is a product in which both numbers are smaller than 4. Primes are central in number theory because of the fundamental theorem of arithmetic: every natural number greater than 1 is either a prime itself or can be factorized as a product of primes that is unique up to their order.

In arithmetic geometry, the Mordell conjecture is the conjecture made by Louis Mordell that a curve of genus greater than 1 over the field Q of rational numbers has only finitely many rational points. In 1983 it was proved by Gerd Faltings, and is now known as Faltings's theorem. The conjecture was later generalized by replacing Q by any number field.
The modularity theorem states that elliptic curves over the field of rational numbers are related to modular forms. Andrew Wiles proved the modularity theorem for semistable elliptic curves, which was enough to imply Fermat's Last Theorem. Later, a series of papers by Wiles's former students Brian Conrad, Fred Diamond and Richard Taylor, culminating in a joint paper with Christophe Breuil, extended Wiles's techniques to prove the full modularity theorem in 2001.

Algebraic number theory is a branch of number theory that uses the techniques of abstract algebra to study the integers, rational numbers, and their generalizations. Number-theoretic questions are expressed in terms of properties of algebraic objects such as algebraic number fields and their rings of integers, finite fields, and function fields. These properties, such as whether a ring admits unique factorization, the behavior of ideals, and the Galois groups of fields, can resolve questions of primary importance in number theory, like the existence of solutions to Diophantine equations.
Gorō Shimura was a Japanese mathematician and Michael Henry Strater Professor Emeritus of Mathematics at Princeton University who worked in number theory, automorphic forms, and arithmetic geometry. He was known for developing the theory of complex multiplication of abelian varieties and Shimura varieties, as well as posing the Taniyama–Shimura conjecture which ultimately led to the proof of Fermat's Last Theorem.

Jean-Pierre Serre is a French mathematician who has made contributions to algebraic topology, algebraic geometry, and algebraic number theory. He was awarded the Fields Medal in 1954, the Wolf Prize in 2000 and the inaugural Abel Prize in 2003.

John Torrence Tate Jr. was an American mathematician, distinguished for many fundamental contributions in algebraic number theory, arithmetic geometry and related areas in algebraic geometry. He was awarded the Abel Prize in 2010.
Ribet's theorem is part of number theory. It concerns properties of Galois representations associated with modular forms. It was proposed by Jean-Pierre Serre and proven by Ken Ribet. The proof was a significant step towards the proof of Fermat's Last Theorem (FLT). As shown by Serre and Ribet, the Taniyama–Shimura conjecture and the epsilon conjecture together imply that FLT is true.

The Disquisitiones Arithmeticae is a textbook of number theory written in Latin by Carl Friedrich Gauss in 1798 when Gauss was 21 and first published in 1801 when he was 24. It is notable for having had a revolutionary impact on the field of number theory as it not only made the field truly rigorous and systematic but also paved the path for modern number theory. In this book Gauss brought together and reconciled results in number theory obtained by mathematicians such as Fermat, Euler, Lagrange, and Legendre and added many profound and original results of his own.

Serge Lang was a French-American mathematician and activist who taught at Yale University for most of his career. He is known for his work in number theory and for his mathematics textbooks, including the influential Algebra. He received the Frank Nelson Cole Prize in 1960 and was a member of the Bourbaki group.

In mathematics, arithmetic geometry is roughly the application of techniques from algebraic geometry to problems in number theory. Arithmetic geometry is centered around Diophantine geometry, the study of rational points of algebraic varieties.
This is a glossary of arithmetic and diophantine geometry in mathematics, areas growing out of the traditional study of Diophantine equations to encompass large parts of number theory and algebraic geometry. Much of the theory is in the form of proposed conjectures, which can be related at various levels of generality.
A height function is a function that quantifies the complexity of mathematical objects. In Diophantine geometry, height functions quantify the size of solutions to Diophantine equations and are typically functions from a set of points on algebraic varieties to the real numbers.

In number theory, Fermat's Last Theorem states that no three positive integers a, b, and c satisfy the equation an + bn = cn for any integer value of n greater than 2. The cases n = 1 and n = 2 have been known since antiquity to have infinitely many solutions.

Wiles's proof of Fermat's Last Theorem is a proof by British mathematician Andrew Wiles of a special case of the modularity theorem for elliptic curves. Together with Ribet's theorem, it provides a proof for Fermat's Last Theorem. Both Fermat's Last Theorem and the modularity theorem were almost universally considered inaccessible to prove by contemporaneous mathematicians, meaning that they were believed to be impossible to prove using current knowledge.
Harold Mortimer Edwards, Jr. was an American mathematician working in number theory, algebra, and the history and philosophy of mathematics.
References
- ↑ for example, Weil's essay on Gaussian sums and cyclotomic fields, La cyclotomie jadis et naguère, 1974
- ↑ American Mathematical Monthly. volume 102, number 6, June/July 1995, pp. 495–505.
- ↑ Reviews of A Classical Introduction to Modern Number Theory:
- Childs, Lindsay N. (1983), "(review)", Mathematical Reviews , MR 0661047
- Odoni, R.W.K. (January 1984), "(review)", Bulletin of the London Mathematical Society, 16 (1): 59–60, doi:10.1112/blms/16.1.59b
- Roberts, Joseph B. (May 1984), "(review)", The American Mathematical Monthly , 91 (5): 319, doi:10.2307/2322691, JSTOR 2322691
- Abbott, S.J. (July 1992), "(review)", The Mathematical Gazette, 76 (476): 316, doi:10.2307/3619180, JSTOR 3619180
- Stevens, Glenn (1992), "(review)", Mathematical Reviews , Graduate Texts in Mathematics, 84, doi:10.1007/978-1-4757-2103-4, ISBN 978-1-4419-3094-1, MR 1070716
- Gouvêa, Fernando Q. (January 2006), "(review)", MAA Reviews
- ↑ Reviews of Number Theory in Function Fields:
- Gekeler, Ernst-Ulrich (2003), "(review)", Mathematical Reviews , Graduate Texts in Mathematics, 210, doi:10.1007/978-1-4757-6046-0, ISBN 978-1-4419-2954-9, MR 1876657 (featured review)
- Goss, David (2004), "(review)", Bulletin of the American Mathematical Society , 41 (1): 127–133, doi: 10.1090/s0273-0979-03-00999-6