Plausible reasoning

Last updated September 18, 2023

Plausible reasoning is a method of deriving new conclusions from given known premises, a method different from the classical syllogistic argumentation methods of Aristotelian two-valued logic. The syllogistic style of argumentation is illustrated by the oft-quoted argument "All men are mortal, Socrates is a man, and therefore, Socrates is mortal." In contrast, consider the statement "if it is raining then it is cloudy." The only logical inference that one can draw from this is that "if it is not cloudy then it is not raining." But ordinary people in their everyday lives would conclude that "if it is not raining then being cloudy is less plausible," or "if it is cloudy then rain is more plausible." The unstated and unconsciously applied reasoning, arguably incorrect, that made people come to their conclusions is typical of plausible reasoning^{[ citation needed ]}.

Plausibility reasoning in ancient Greece
George Polya and plausible reasoning
Volume I: Induction and analogy in mathematics
Volume II: Patterns of Plausible Inference
Characteristics of plausible argument
Some of the problems associated with the formulation of a theory of plausible reasoning
References
Further reading

As another example, look at this scenario:^[1] "Suppose some dark night a policeman walks down a street, apparently deserted; but suddenly he hears a burglar alarm, looks across the street, and sees a jewellery store with a broken window. Then a person wearing a mask comes crawling out through the broken window, carrying a bag which turns out to be full of expensive jewellery. The policeman immediately concludes that this person is stealing the jewellery." By what reasoning process does the policeman arrive at this conclusion?

Clearly the policeman's conclusion was not a logical deduction from the evidence. There may be a perfectly valid explanation for everything. For example, it might be that this person was the owner of the jewellery store and he was coming home from a fancy dress competition, and he didn't have the key with him. But just as he walked by his store a passing truck threw a stone through the window; and he was only protecting his own property and not stealing the jewellery. Now whatever be the policeman's reasoning process, it has a certain degree of validity. The evidence did not prove that the person was stealing jewellery, but it did make it extremely plausible. This is an example of a kind of reasoning, often referred to as plausible reasoning, in which most people are generally very proficient.

Plausibility reasoning in ancient Greece

During the fifth century B.C.E.,^[2] judicial orators in Greek Sicily developed a method for successfully pleading their cases in such instances in which no eyewitnesses or written documents or other such direct evidence could be produced. They began to base their arguments on the internal or external probability or plausibility of their statements. This new way of arguing was commonly labeled with the Greek term eikós, a term that has been variously rendered as similarity, likelihood, probability or plausibility. The success of the argument depends on the oratorical skills of the speaker, arguments by eikós have often been accused of lack of truthfulness. Here is a classical example of argument by plausible reasoning presented by Aristotle in his Rhetoric:

"If the accused is not open to the charge – for instance if a weakling be tried for violent assault – the defence is that he was not likely (eikós) to do such a thing. But if he is open to the charge – that is, if he is a strong man – the defence is still that he was not likely (eikós) to do such a thing, since he could be sure that people would think he was likely (eikós) to do it."

The sophists, a sort of mendicant academicians were said to have been experts in this type of argumentation and they are said to have taught wealthy young Greeks these methods for a hefty fee. Plato and Aristotle strongly denounced these methods and the method came to acquire a lot of bad repute. Sophistic argumentation styles were equated with fallacious arguments.

George Polya and plausible reasoning

George Polya in his two volume book titled Mathematics and Plausible Reasoning ^[3]^[4] presents plausible reasoning as a way of generating new mathematical conjectures. To Polya, “a mathematical proof is demonstrative reasoning but the inductive evidence of the physicist, the circumstantial evidence of the lawyer, the documentary evidence of the historian and the statistical evidence of the economist all belong to plausible reasoning”. Polya’s intention is to teach students the art of guessing new results in mathematics for which he marshals such notions as induction and analogy as possible sources for plausible reasoning. The first volume of the book is devoted to an extensive discussion of these ideas with several examples drawn from various field of mathematics.

In the Preface to Volume 1 of the book Polya exhorts all interested students of mathematics thus: "Certainly, let us learn proving, but also let us learn guessing." P. R. Halmos reviewing the book summarized the central thesis of the book thus: ". . . a good guess is as important as a good proof."^[5]

Volume I: Induction and analogy in mathematics

Polya begins Volume I with a discussion on inductive reasoning (not mathematical induction) as a way of guessing new results. He shows how the chance observations of a few results of the form 4 = 2 + 2, 6 = 3 + 3, 8 = 3 + 5, 10 = 3 + 7, etc., may prompt a sharp mind to formulate the conjecture that every even number greater than 4 can be represented as the sum of two odd prime numbers. This is the well known Goldbach's conjecture. The first problem in the first chapter is to guess the rule according to which the successive terms of the following sequence are chosen: 11, 31, 41, 61, 71, 101, 131, . . . In the next chapter the techniques of generalization, specialization and analogy are presented as possible strategies for plausible reasoning. In the remaining chapters, these ideas are illustrated by discussing the discovery of several results in various fields of mathematics like number theory, geometry, etc. and also in physical sciences.

Volume II: Patterns of Plausible Inference

This volume attempts to formulate certain patterns of plausible reasoning. The relations of these patterns with the calculus of probability are also investigated. Their relation to mathematical invention and instruction are also discussed. The following are some of the patterns of plausible inference discussed by Polya.

Sl. No.	Premise 1	Premise 2	Premise 3	Plausible conclusion
1	A implies B	B true	-	A more credible
2	A implies B_n+1	B_n+1 very different from the formerly verified consequences B₁, B₂, . . ., B_n of A	B_n+1 true	A much more credible
3	A implies B_n+1	B_n+1 very similar to the formerly verified consequences B₁, B₂, . . ., B_n of A	B_n+1 true	A just a little more credible
4	A implies B	B very improbable in itself	B true	A very much more credible
5	A implies B	B quite probable in itself	B true	A just a little more credible
6	A analogous to B	B true	-	A more credible
7	A analogous to B	B more credible	--	A somewhat more credible
8	A is implied B	B false	--	A less credible
9	A is incompatible with B	B false	--	A more credible

Characteristics of plausible argument

After a detailed analysis of several paradigmatic examples drawn from ancient Greek texts, D Walton and others formulated the following eleven properties as the defining characteristics of plausible reasoning.^[6]

Plausible reasoning proceeds from premises that are more plausible to a conclusion that was less plausible before the plausible argument.
Something is found plausible when hearers have examples in their own minds.
Plausible reasoning is based on common knowledge.
Plausible reasoning is defeasible.
Plausible reasoning is based on the way things generally go in familiar situations.
Plausible reasoning can be used to fill in implicit premises in incomplete arguments.
Plausible reasoning is commonly based on appearances from perception.
Stability is an important characteristic of plausible reasoning.
Plausible reasoning can be tested, and by this means, confirmed or refuted.
Probing into plausible reasoning in a dialogue is a way of testing it.
Plausible reasoning admits of degrees by testing, but of a kind different from those the standard probability values and Bayesian rules used in Pascalian probability

Some of the problems associated with the formulation of a theory of plausible reasoning

Allan M. Collins, a recognized authority on intelligent tutoring systems and plausible reasoning, presenting a core theory of the logic of plausible reasoning identified some of the important problems in the formulation of such a theory.^[7]

1. Representing degree of belief.

This is the problem of representing the differences in the strengths of beliefs indicated by the phrases “entirely certain” and “would guess”.

2. Evaluating the strength of arguments.

We need a computational scheme to calculate and compare different levels and strengths of belief.

3. Applying rules of general but not universal validity.

Standard logic justifies the use of universally quantified rules; rules that are always true without exception. Much commonsense inference relies on applying default rules that hold in general but not always.

4. Avoiding the enumeration of all the conditions on a rule.

It often happens that a plausible commonsense rule, when examined closely, has an almost unlimited number of possible types of exceptions. The problem of dealing with all these potential exceptions is known as the qualification problem.

5. Inference from the absence of information.

It is often reasonable to infer that a statement A is false from the fact that one does not know A to be true, or from the fact that it is not stated to be true in a problem statement.

6. Limiting the extent of inference.

Many intuitively appealing sets of axioms have the property that the first few inferences all seem to be reasonable and to have reasonable conclusions, but that, as the inferences get further and further from the starting axioms, the conclusions seem less and less sensible, and they eventually end up in pure nonsense.

7. Inference using vague concepts.

Inferences that involve reasoning near the boundaries of a vague concept are often uncertain.

8. Finding expected utility.

This is the problem of choosing between actions whose consequences are uncertain. In such a case, a choice may be made based on the likelihoods of the various outcomes with their desirability.

9. Inferring an explanation.

Commonsense reasoners try to explain the reasons underlying their observations. If I observe that the street is wet, I infer that it rained. If I observe that the sidewalk is not wet, I may decide instead that the street-cleaners have been by.

10. Schema-Based Inference.

Many useful commonsense concepts correspond to large systems of relations that are instantiated in many separate instances in the world. Such concepts are called schemas or frames.

11. Inferring a general rule from examples.

People are always on the lookout for general rules that encapsulate their observations.

Related Research Articles

<span class="mw-page-title-main">George Pólya</span> Hungarian mathematician (1887–1985)

George Pólya was a Hungarian mathematician. He was a professor of mathematics from 1914 to 1940 at ETH Zürich and from 1940 to 1953 at Stanford University. He made fundamental contributions to combinatorics, number theory, numerical analysis and probability theory. He is also noted for his work in heuristics and mathematics education. He has been described as one of The Martians, an informal category which included one of his most famous students at ETH Zurich, John von Neumann.

In propositional logic, modus ponens, also known as modus ponendo ponens, implication elimination, or affirming the antecedent, is a deductive argument form and rule of inference. It can be summarized as "P implies Q.P is true. Therefore Q must also be true."

The scientific method is an empirical method for acquiring knowledge that has characterized the development of science since at least the 17th century It involves careful observation, applying rigorous skepticism about what is observed, given that cognitive assumptions can distort how one interprets the observation. It involves formulating hypotheses, via induction, based on such observations; the testability of hypotheses, experimental and the measurement-based statistical testing of deductions drawn from the hypotheses; and refinement of the hypotheses based on the experimental findings. These are principles of the scientific method, as distinguished from a definitive series of steps applicable to all scientific enterprises.

Abductive reasoning is a form of logical inference that seeks the simplest and most likely conclusion from a set of observations. It was formulated and advanced by American philosopher Charles Sanders Peirce beginning in the last third of the 19th century.

Deductive reasoning is the mental process of drawing deductive inferences. An inference is deductively valid if its conclusion follows logically from its premises, i.e. it is impossible for the premises to be true and the conclusion to be false.

A mathematical proof is a deductive argument for a mathematical statement, showing that the stated assumptions logically guarantee the conclusion. The argument may use other previously established statements, such as theorems; but every proof can, in principle, be constructed using only certain basic or original assumptions known as axioms, along with the accepted rules of inference. Proofs are examples of exhaustive deductive reasoning which establish logical certainty, to be distinguished from empirical arguments or non-exhaustive inductive reasoning which establish "reasonable expectation". Presenting many cases in which the statement holds is not enough for a proof, which must demonstrate that the statement is true in all possible cases. A proposition that has not been proved but is believed to be true is known as a conjecture, or a hypothesis if frequently used as an assumption for further mathematical work.

First formulated by David Hume, the problem of induction questions our reasons for believing that the future will resemble the past, or more broadly it questions predictions about unobserved things based on previous observations. This inference from the observed to the unobserved is known as "inductive inferences", and Hume, while acknowledging that everyone does and must make such inferences, argued that there is no non-circular way to justify them, thereby undermining one of the Enlightenment pillars of rationality.

Inferences are steps in reasoning, moving from premises to logical consequences; etymologically, the word infer means to "carry forward". Inference is theoretically traditionally divided into deduction and induction, a distinction that in Europe dates at least to Aristotle. Deduction is inference deriving logical conclusions from premises known or assumed to be true, with the laws of valid inference being studied in logic. Induction is inference from particular evidence to a universal conclusion. A third type of inference is sometimes distinguished, notably by Charles Sanders Peirce, contradistinguishing abduction from induction.

Inductive reasoning is a method of reasoning in which a general principle is derived from a body of observations. It consists of making broad generalizations based on specific observations. Inductive reasoning is distinct from deductive reasoning, where the conclusion of a deductive argument is certain given the premises are correct; in contrast, the truth of the conclusion of an inductive argument is probable, based upon the evidence given.

Logical reasoning is a mental activity that aims to arrive at a conclusion in a rigorous way. It happens in the form of inferences or arguments by starting from a set of premises and reasoning to a conclusion supported by these premises. The premises and the conclusion are propositions, i.e. true or false claims about what is the case. Together, they form an argument. Logical reasoning is norm-governed in the sense that it aims to formulate correct arguments that any rational person would find convincing. The main discipline studying logical reasoning is called logic.

In artificial intelligence (AI), commonsense reasoning is a human-like ability to make presumptions about the type and essence of ordinary situations humans encounter every day. These assumptions include judgments about the nature of physical objects, taxonomic properties, and peoples' intentions. A device that exhibits commonsense reasoning might be capable of drawing conclusions that are similar to humans' folk psychology and naive physics.

An inquiry is any process that has the aim of augmenting knowledge, resolving doubt, or solving a problem. A theory of inquiry is an account of the various types of inquiry and a treatment of the ways that each type of inquiry achieves its aim.

Probabilistic logic involves the use of probability and logic to deal with uncertain situations. Probabilistic logic extends traditional logic truth tables with probabilistic expressions. A difficulty of probabilistic logics is their tendency to multiply the computational complexities of their probabilistic and logical components. Other difficulties include the possibility of counter-intuitive results, such as in case of belief fusion in Dempster–Shafer theory. Source trust and epistemic uncertainty about the probabilities they provide, such as defined in subjective logic, are additional elements to consider. The need to deal with a broad variety of contexts and issues has led to many different proposals.

An argument is a series of sentences, statements or propositions some of which are called premises and one is the conclusion. The purpose of an argument is to give reasons for one's conclusion via justification, explanation, and/or persuasion.

The psychology of reasoning is the study of how people reason, often broadly defined as the process of drawing conclusions to inform how people solve problems and make decisions. It overlaps with psychology, philosophy, linguistics, cognitive science, artificial intelligence, logic, and probability theory.

Philosophy of logic is the area of philosophy that studies the scope and nature of logic. It investigates the philosophical problems raised by logic, such as the presuppositions often implicitly at work in theories of logic and in their application. This involves questions about how logic is to be defined and how different logical systems are connected to each other. It includes the study of the nature of the fundamental concepts used by logic and the relation of logic to other disciplines. According to a common characterization, philosophical logic is the part of the philosophy of logic that studies the application of logical methods to philosophical problems, often in the form of extended logical systems like modal logic. But other theorists draw the distinction between the philosophy of logic and philosophical logic differently or not at all. Metalogic is closely related to the philosophy of logic as the discipline investigating the properties of formal logical systems, like consistency and completeness.

Argument from analogy or false analogy is a special type of inductive argument, where perceived similarities are used as a basis to infer some further similarity that has not been observed yet. Analogical reasoning is one of the most common methods by which human beings try to understand the world and make decisions. When a person has a bad experience with a product and decides not to buy anything further from the producer, this is often a case of analogical reasoning since the two products share a maker and are therefore both perceived as "bad". It is also the basis of much of science; for instance, experiments on laboratory rats are based on the fact that some physiological similarities between rats and humans implies some further similarity.

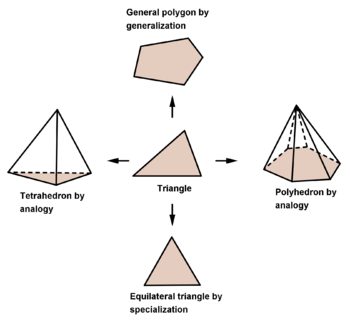
Mathematics and Plausible Reasoning is a two-volume book by the mathematician George Pólya describing various methods for being a good guesser of new mathematical results. In the Preface to Volume 1 of the book Pólya exhorts all interested students of mathematics thus: "Certainly, let us learn proving, but also let us learn guessing." P. R. Halmos reviewing the book summarised the central thesis of the book thus: ".. . a good guess is as important as a good proof."

Logic is the study of correct reasoning. It includes both formal and informal logic. Formal logic is the science of deductively valid inferences or logical truths. It studies how conclusions follow from premises due to the structure of arguments alone, independent of their topic and content. Informal logic is associated with informal fallacies, critical thinking, and argumentation theory. It examines arguments expressed in natural language while formal logic uses formal language. When used as a countable noun, the term "a logic" refers to a logical formal system that articulates a proof system. Logic plays a central role in many fields, such as philosophy, mathematics, computer science, and linguistics.

As the study of argument is of clear importance to the reasons that we hold things to be true, logic is of essential importance to rationality. Arguments may be logical if they are "conducted or assessed according to strict principles of validity", while they are rational according to the broader requirement that they are based on reason and knowledge.

References

↑ E.T. Jaynes (2003). Probability Theory: The Logic of Science. Cambridge University Press. p. 3.
↑ Manfred Kraus. "Early Greek Probability Arguments and Common Ground in Dissensus". In H. V. Hansen; et al. (eds.). Dissensus and the Search for Common Ground. Windsor, ON: OSSA. pp. 1–11. Retrieved 21 February 2015.
↑ George Polya (1954). Mathematics and Plausible Reasoning Volume I: Induction and Analogy in Mathematics. Princeton University Press.
↑ George Polya (1954). Mathematics and Plausible reasoning Volume II: Patterns of Plausible Inference. Princeton University Press.
↑ P. R. Halmos (1955). "Review: G. Polya, Mathematics and plausible reasoning". Bulletin of the American Mathematical Society. 61 (3): 243–245. doi:10.1090/s0002-9904-1955-09904-x . Retrieved 21 February 2015.
↑ D. Walton; C. W. Tindale; T. F. Gordon (2014). "Applying recent argumentation methods to some ancient examples of plausible reasoning" (PDF). Argumentation. 28 (1): 85–119. doi:10.1007/s10503-013-9306-y. S2CID 17870888. Archived from the original (PDF) on 21 February 2015. Retrieved 21 February 2015.
↑ Allan Collins (1989). "The Logic of Plausible Reasoning: A Core Theory". Cognitive Science. 13: 1–49. doi: 10.1207/s15516709cog1301_1 .