In number theory, an arithmetic, arithmetical, or number-theoretic function is generally any function f(n) whose domain is the positive integers and whose range is a subset of the complex numbers. Hardy & Wright include in their definition the requirement that an arithmetical function "expresses some arithmetical property of n". There is a larger class of number-theoretic functions that do not fit this definition, for example, the prime-counting functions. This article provides links to functions of both classes.
In number theory, the Legendre symbol is a multiplicative function with values 1, −1, 0 that is a quadratic character modulo of an odd prime number p: its value at a (nonzero) quadratic residue mod p is 1 and at a non-quadratic residue (non-residue) is −1. Its value at zero is 0.

In number theory, the law of quadratic reciprocity is a theorem about modular arithmetic that gives conditions for the solvability of quadratic equations modulo prime numbers. Due to its subtlety, it has many formulations, but the most standard statement is:
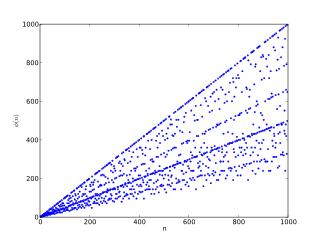
In number theory, Euler's totient function counts the positive integers up to a given integer n that are relatively prime to n. It is written using the Greek letter phi as or , and may also be called Euler's phi function. In other words, it is the number of integers k in the range 1 ≤ k ≤ n for which the greatest common divisor gcd(n, k) is equal to 1. The integers k of this form are sometimes referred to as totatives of n.

The Jacobi symbol is a generalization of the Legendre symbol. Introduced by Jacobi in 1837, it is of theoretical interest in modular arithmetic and other branches of number theory, but its main use is in computational number theory, especially primality testing and integer factorization; these in turn are important in cryptography.
In number theory, Euler's criterion is a formula for determining whether an integer is a quadratic residue modulo a prime. Precisely,
In analytic number theory and related branches of mathematics, a complex-valued arithmetic function :\mathbb {Z} \rightarrow \mathbb {C} } is a Dirichlet character of modulus if for all integers and :
- that is, is completely multiplicative.
- ; that is, is periodic with period .
In number theory, an integer q is called a quadratic residue modulo n if it is congruent to a perfect square modulo n; i.e., if there exists an integer x such that:
In mathematics, a reciprocity law is a generalization of the law of quadratic reciprocity to arbitrary monic irreducible polynomials with integer coefficients. Recall that first reciprocity law, quadratic reciprocity, determines when an irreducible polynomial splits into linear terms when reduced mod . That is, it determines for which prime numbers the relation
In mathematics, in the area of number theory, a Gaussian period is a certain kind of sum of roots of unity. The periods permit explicit calculations in cyclotomic fields connected with Galois theory and with harmonic analysis. They are basic in the classical theory called cyclotomy. Closely related is the Gauss sum, a type of exponential sum which is a linear combination of periods.
In number theory, the Kronecker symbol, written as or , is a generalization of the Jacobi symbol to all integers . It was introduced by Leopold Kronecker.
Gauss's lemma in number theory gives a condition for an integer to be a quadratic residue. Although it is not useful computationally, it has theoretical significance, being involved in some proofs of quadratic reciprocity.
In mathematics, a Kloosterman sum is a particular kind of exponential sum. They are named for the Dutch mathematician Hendrik Kloosterman, who introduced them in 1926 when he adapted the Hardy–Littlewood circle method to tackle a problem involving positive definite diagonal quadratic forms in four variables, strengthening his 1924 dissertation research on five or more variables.
In number theory, the law of quadratic reciprocity, like the Pythagorean theorem, has lent itself to an unusually large number of proofs. Several hundred proofs of the law of quadratic reciprocity have been published.
In mathematics, a natural number a is a unitary divisor of a number b if a is a divisor of b and if a and are coprime, having no common factor other than 1. Equivalently, a divisor a of b is a unitary divisor if and only if every prime factor of a has the same multiplicity in a as it has in b.
In number theory, Ramanujan's sum, usually denoted cq(n), is a function of two positive integer variables q and n defined by the formula
Cubic reciprocity is a collection of theorems in elementary and algebraic number theory that state conditions under which the congruence x3 ≡ p (mod q) is solvable; the word "reciprocity" comes from the form of the main theorem, which states that if p and q are primary numbers in the ring of Eisenstein integers, both coprime to 3, the congruence x3 ≡ p is solvable if and only if x3 ≡ q is solvable.
Quartic or biquadratic reciprocity is a collection of theorems in elementary and algebraic number theory that state conditions under which the congruence x4 ≡ p is solvable; the word "reciprocity" comes from the form of some of these theorems, in that they relate the solvability of the congruence x4 ≡ p to that of x4 ≡ q.
In algebraic number theory Eisenstein's reciprocity law is a reciprocity law that extends the law of quadratic reciprocity and the cubic reciprocity law to residues of higher powers. It is one of the earliest and simplest of the higher reciprocity laws, and is a consequence of several later and stronger reciprocity laws such as the Artin reciprocity law. It was introduced by Eisenstein, though Jacobi had previously announced a similar result for the special cases of 5th, 8th and 12th powers in 1839.































