In geometry, the incircle of the medial triangle of a triangle is the Spieker circle, named after 19th-century German geometer Theodor Spieker. [1] Its center, the Spieker center, in addition to being the incenter of the medial triangle, is the center of mass of the uniform-density boundary of triangle. [1] The Spieker center is also the point where all three cleavers of the triangle (perimeter bisectors with an endpoint at a side's midpoint) intersect each other. [1]
The Spieker circle and Spieker center are named after Theodor Spieker, a mathematician and professor from Potsdam, Germany. In 1862, he published Lehrbuch der ebenen geometrie mit übungsaufgaben für höhere lehranstalten, dealing with planar geometry. Due to this publication, influential in the lives of many famous scientists and mathematicians including Albert Einstein, Spieker became the mathematician for whom the Spieker circle and center were named. [1]
To find the Spieker circle of a triangle, the medial triangle must first be constructed from the midpoints of each side of the original triangle. [1] The circle is then constructed in such a way that each side of the medial triangle is tangent to the circle within the medial triangle, creating the incircle. [1] This circle center is named the Spieker center.
Spieker circles also have relations to Nagel points. The incenter of the triangle and the Nagel point form a line within the Spieker circle. The middle of this line segment is the Spieker center. [1] The Nagel line is formed by the incenter of the triangle, the Nagel point, and the centroid of the triangle. [1] The Spieker center will always lie on this line. [1]
Spieker circles were first found to be very similar to nine-point circles by Julian Coolidge. At this time, it was not yet identified as the Spieker circle, but is referred to as the "P circle" throughout the book. [2] The nine-point circle with the Euler line and the Spieker circle with the Nagel line are analogous to each other, but are not duals, only having dual-like similarities. [1] One similarity between the nine-point circle and the Spieker circle deals with their construction. The nine-point circle is the circumscribed circle of the medial triangle, while the Spieker circle is the inscribed circle of the medial triangle. [2] With relation to their associated lines, the incenter for the Nagel line relates to the circumcenter for the Euler line. [1] Another analogous point is the Nagel point and the othocenter, with the Nagel point associated with the Spieker circle and the orthocenter associated with the nine-point circle. [1] Each circle meets the sides of the medial triangle where the lines from the orthocenter, or the Nagel point, to the vertices of the original triangle meet the sides of the medial triangle. [2]
The nine-point circle with the Euler line was generalized into the nine-point conic. [1] Through a similar process, due to the analogous properties of the two circles, the Spieker circle was also able to be generalized into the Spieker conic. [1] The Spieker conic is still found within the medial triangle and touches each side of the medial triangle, however it does not meet those sides of the triangle at the same points. If lines are constructed from each vertex of the medial triangle to the Nagel point, then the midpoint of each of those lines can be found. [3] Also, the midpoints of each side of the medial triangle are found and connected to the midpoint of the opposite line through the Nagel point. [3] Each of these lines share a common midpoint, S. [3] With each of these lines reflected through S, the result is 6 points within the medial triangle. Draw a conic through any 5 of these reflected points and the conic will touch the final point. [1] This was proven by de Villiers in 2006. [1]
The Spieker radical circle is the circle, centered at the Spieker center, which is orthogonal to the three excircles of the medial triangle. [4] [5]
In geometry, an altitude of a triangle is a line segment through a vertex and perpendicular to a line containing the side opposite the vertex. This line containing the opposite side is called the extended base of the altitude. The intersection of the extended base and the altitude is called the foot of the altitude. The length of the altitude, often simply called "the altitude", is the distance between the extended base and the vertex. The process of drawing the altitude from the vertex to the foot is known as dropping the altitude at that vertex. It is a special case of orthogonal projection.
In geometry, the nine-point circle is a circle that can be constructed for any given triangle. It is so named because it passes through nine significant concyclic points defined from the triangle. These nine points are:
In geometry, the incircle or inscribed circle of a triangle is the largest circle that can be contained in the triangle; it touches the three sides. The center of the incircle is a triangle center called the triangle's incenter.
In geometry, an orthocentric system is a set of four points on a plane, one of which is the orthocenter of the triangle formed by the other three. Equivalently, the lines passing through disjoint pairs among the points are perpendicular, and the four circles passing through any three of the four points have the same radius.
In geometry, the Euler line, named after Leonhard Euler, is a line determined from any triangle that is not equilateral. It is a central line of the triangle, and it passes through several important points determined from the triangle, including the orthocenter, the circumcenter, the centroid, the Exeter point and the center of the nine-point circle of the triangle.
In geometry, the incenter of a triangle is a triangle center, a point defined for any triangle in a way that is independent of the triangle's placement or scale. The incenter may be equivalently defined as the point where the internal angle bisectors of the triangle cross, as the point equidistant from the triangle's sides, as the junction point of the medial axis and innermost point of the grassfire transform of the triangle, and as the center point of the inscribed circle of the triangle.
In the geometry of triangles, the incircle and nine-point circle of a triangle are internally tangent to each other at the Feuerbach point of the triangle. The Feuerbach point is a triangle center, meaning that its definition does not depend on the placement and scale of the triangle. It is listed as X(11) in Clark Kimberling's Encyclopedia of Triangle Centers, and is named after Karl Wilhelm Feuerbach.
In geometry, the semiperimeter of a polygon is half its perimeter. Although it has such a simple derivation from the perimeter, the semiperimeter appears frequently enough in formulas for triangles and other figures that it is given a separate name. When the semiperimeter occurs as part of a formula, it is typically denoted by the letter s.
In geometry, lines in a plane or higher-dimensional space are concurrent if they intersect at a single point.
In geometry, collinearity of a set of points is the property of their lying on a single line. A set of points with this property is said to be collinear. In greater generality, the term has been used for aligned objects, that is, things being "in a line" or "in a row".
In Euclidean geometry, the medial triangle or midpoint triangle of a triangle △ABC is the triangle with vertices at the midpoints of the triangle's sides AB, AC, BC. It is the n = 3 case of the midpoint polygon of a polygon with n sides. The medial triangle is not the same thing as the median triangle, which is the triangle whose sides have the same lengths as the medians of △ABC.
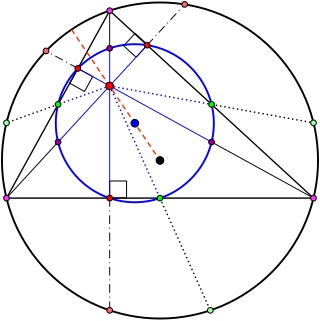
In geometry, the nine-point center is a triangle center, a point defined from a given triangle in a way that does not depend on the placement or scale of the triangle. It is so called because it is the center of the nine-point circle, a circle that passes through nine significant points of the triangle: the midpoints of the three edges, the feet of the three altitudes, and the points halfway between the orthocenter and each of the three vertices. The nine-point center is listed as point X(5) in Clark Kimberling's Encyclopedia of Triangle Centers.
In geometry, the Nagel point is a triangle center, one of the points associated with a given triangle whose definition does not depend on the placement or scale of the triangle. It is the point of concurrency of all three of the triangle's splitters.
In geometry, the mittenpunkt of a triangle is a triangle center: a point defined from the triangle that is invariant under Euclidean transformations of the triangle. It was identified in 1836 by Christian Heinrich von Nagel as the symmedian point of the excentral triangle of the given triangle.
In Euclidean geometry with triangle △ABC, the nine-point hyperbola is an instance of the nine-point conic described by American mathematician Maxime Bôcher in 1892. The celebrated nine-point circle is a separate instance of Bôcher's conic:
In geometry, the Spieker center is a special point associated with a plane triangle. It is defined as the center of mass of the perimeter of the triangle. The Spieker center of a triangle △ABC is the center of gravity of a homogeneous wire frame in the shape of △ABC. The point is named in honor of the 19th-century German geometer Theodor Spieker. The Spieker center is a triangle center and it is listed as the point X(10) in Clark Kimberling's Encyclopedia of Triangle Centers.
In geometry, central lines are certain special straight lines that lie in the plane of a triangle. The special property that distinguishes a straight line as a central line is manifested via the equation of the line in trilinear coordinates. This special property is related to the concept of triangle center also. The concept of a central line was introduced by Clark Kimberling in a paper published in 1994.
In geometry, the nine-point conic of a complete quadrangle is a conic that passes through the three diagonal points and the six midpoints of sides of the complete quadrangle.
In geometry, the Bevan point, named after Benjamin Bevan, is a triangle center. It is defined as center of the Bevan circle, that is the circle through the centers of the three excircles of a triangle.