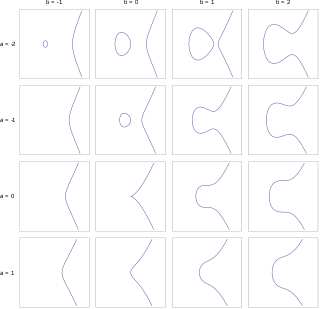
In mathematics, an elliptic curve is a smooth, projective, algebraic curve of genus one, on which there is a specified point O. An elliptic curve is defined over a field K and describes points in K2, the Cartesian product of K with itself. If the field's characteristic is different from 2 and 3, then the curve can be described as a plane algebraic curve which consists of solutions (x, y) for:
The Lenstra elliptic-curve factorization or the elliptic-curve factorization method (ECM) is a fast, sub-exponential running time, algorithm for integer factorization, which employs elliptic curves. For general-purpose factoring, ECM is the third-fastest known factoring method. The second-fastest is the multiple polynomial quadratic sieve, and the fastest is the general number field sieve. The Lenstra elliptic-curve factorization is named after Hendrik Lenstra.

Faltings's theorem is a result in arithmetic geometry, according to which a curve of genus greater than 1 over the field of rational numbers has only finitely many rational points. This was conjectured in 1922 by Louis Mordell, and known as the Mordell conjecture until its 1983 proof by Gerd Faltings. The conjecture was later generalized by replacing by any number field.
The modularity theorem states that elliptic curves over the field of rational numbers are related to modular forms in a particular way. Andrew Wiles and Richard Taylor proved the modularity theorem for semistable elliptic curves, which was enough to imply Fermat's Last Theorem. Later, a series of papers by Wiles's former students Brian Conrad, Fred Diamond and Richard Taylor, culminating in a joint paper with Christophe Breuil, extended Wiles's techniques to prove the full modularity theorem in 2001.
In number theory, the local zeta functionZ(V, s) (sometimes called the congruent zeta function or the Hasse–Weil zeta function) is defined as
In number theory, Iwasawa theory is the study of objects of arithmetic interest over infinite towers of number fields. It began as a Galois module theory of ideal class groups, initiated by Kenkichi Iwasawa, as part of the theory of cyclotomic fields. In the early 1970s, Barry Mazur considered generalizations of Iwasawa theory to abelian varieties. More recently, Ralph Greenberg has proposed an Iwasawa theory for motives.
In mathematics, the Birch and Swinnerton-Dyer conjecture describes the set of rational solutions to equations defining an elliptic curve. It is an open problem in the field of number theory and is widely recognized as one of the most challenging mathematical problems. It is named after mathematicians Bryan John Birch and Peter Swinnerton-Dyer, who developed the conjecture during the first half of the 1960s with the help of machine computation. As of 2024, only special cases of the conjecture have been proven.
In mathematics, complex multiplication (CM) is the theory of elliptic curves E that have an endomorphism ring larger than the integers. Put another way, it contains the theory of elliptic functions with extra symmetries, such as are visible when the period lattice is the Gaussian integer lattice or Eisenstein integer lattice.
In number theory and algebraic geometry, a modular curveY(Γ) is a Riemann surface, or the corresponding algebraic curve, constructed as a quotient of the complex upper half-plane H by the action of a congruence subgroup Γ of the modular group of integral 2×2 matrices SL(2, Z). The term modular curve can also be used to refer to the compactified modular curvesX(Γ) which are compactifications obtained by adding finitely many points to this quotient. The points of a modular curve parametrize isomorphism classes of elliptic curves, together with some additional structure depending on the group Γ. This interpretation allows one to give a purely algebraic definition of modular curves, without reference to complex numbers, and, moreover, prove that modular curves are defined either over the field of rational numbers Q or a cyclotomic field Q(ζn). The latter fact and its generalizations are of fundamental importance in number theory.
In mathematics, the Hasse–Weil zeta function attached to an algebraic variety V defined over an algebraic number field K is a meromorphic function on the complex plane defined in terms of the number of points on the variety after reducing modulo each prime number p. It is a global L-function defined as an Euler product of local zeta functions.

In mathematics, arithmetic geometry is roughly the application of techniques from algebraic geometry to problems in number theory. Arithmetic geometry is centered around Diophantine geometry, the study of rational points of algebraic varieties.
In number theory and algebraic geometry, a rational point of an algebraic variety is a point whose coordinates belong to a given field. If the field is not mentioned, the field of rational numbers is generally understood. If the field is the field of real numbers, a rational point is more commonly called a real point.
In arithmetic geometry, the Mordell–Weil group is an abelian group associated to any abelian variety defined over a number field , it is an arithmetic invariant of the Abelian variety. It is simply the group of -points of , so is the Mordell–Weil grouppg 207. The main structure theorem about this group is the Mordell–Weil theorem which shows this group is in fact a finitely-generated abelian group. Moreover, there are many conjectures related to this group, such as the Birch and Swinnerton-Dyer conjecture which relates the rank of to the zero of the associated L-function at a special point.
In number theory, the Néron–Tate height is a quadratic form on the Mordell–Weil group of rational points of an abelian variety defined over a global field. It is named after André Néron and John Tate.
Lehmer's conjecture, also known as the Lehmer's Mahler measure problem, is a problem in number theory raised by Derrick Henry Lehmer. The conjecture asserts that there is an absolute constant such that every polynomial with integer coefficients satisfies one of the following properties:
In mathematics the division polynomials provide a way to calculate multiples of points on elliptic curves and to study the fields generated by torsion points. They play a central role in the study of counting points on elliptic curves in Schoof's algorithm.
In mathematics, the main conjecture of Iwasawa theory is a deep relationship between p-adic L-functions and ideal class groups of cyclotomic fields, proved by Kenkichi Iwasawa for primes satisfying the Kummer–Vandiver conjecture and proved for all primes by Mazur and Wiles. The Herbrand–Ribet theorem and the Gras conjecture are both easy consequences of the main conjecture. There are several generalizations of the main conjecture, to totally real fields, CM fields, elliptic curves, and so on.
In mathematics, the Mordell–Weil theorem states that for an abelian variety over a number field , the group of K-rational points of is a finitely-generated abelian group, called the Mordell–Weil group. The case with an elliptic curve and the field of rational numbers is Mordell's theorem, answering a question apparently posed by Henri Poincaré around 1901; it was proved by Louis Mordell in 1922. It is a foundational theorem of Diophantine geometry and the arithmetic of abelian varieties.
In mathematics, the conductor of an elliptic curve over the field of rational numbers is an integral ideal, which is analogous to the Artin conductor of a Galois representation. It is given as a product of prime ideals, together with associated exponents, which encode the ramification in the field extensions generated by the points of finite order in the group law of the elliptic curve. The primes involved in the conductor are precisely the primes of bad reduction of the curve: this is the Néron–Ogg–Shafarevich criterion.
In mathematics, the rank of an elliptic curve is the rational Mordell–Weil rank of an elliptic curve defined over the field of rational numbers. Mordell's theorem says the group of rational points on an elliptic curve has a finite basis. This means that for any elliptic curve there is a finite subset of the rational points on the curve, from which all further rational points may be generated. If the number of rational points on a curve is infinite then some point in a finite basis must have infinite order. The number of independent basis points with infinite order is the rank of the curve.





























