In mathematics, specifically general topology, compactness is a property that seeks to generalize the notion of a closed and bounded subset of Euclidean space. The idea is that a compact space has no "punctures" or "missing endpoints", i.e., it includes all limiting values of points. For example, the open interval (0,1) would not be compact because it excludes the limiting values of 0 and 1, whereas the closed interval [0,1] would be compact. Similarly, the space of rational numbers is not compact, because it has infinitely many "punctures" corresponding to the irrational numbers, and the space of real numbers is not compact either, because it excludes the two limiting values and . However, the extended real number linewould be compact, since it contains both infinities. There are many ways to make this heuristic notion precise. These ways usually agree in a metric space, but may not be equivalent in other topological spaces.

In topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint non-empty open subsets. Connectedness is one of the principal topological properties that are used to distinguish topological spaces.
In topology and related branches of mathematics, a Hausdorff space ( HOWS-dorf, HOWZ-dorf), separated space or T2 space is a topological space where, for any two distinct points, there exist neighbourhoods of each which are disjoint from each other. Of the many separation axioms that can be imposed on a topological space, the "Hausdorff condition" (T2) is the most frequently used and discussed. It implies the uniqueness of limits of sequences, nets, and filters.
This is a glossary of some terms used in the branch of mathematics known as topology. Although there is no absolute distinction between different areas of topology, the focus here is on general topology. The following definitions are also fundamental to algebraic topology, differential topology and geometric topology.
In topology, the Tietze extension theorem states that continuous functions on a closed subset of a normal topological space can be extended to the entire space, preserving boundedness if necessary.

In mathematics, an open set is a generalization of an open interval in the real line.
In mathematics, a topological vector space is one of the basic structures investigated in functional analysis. A topological vector space is a vector space that is also a topological space with the property that the vector space operations are also continuous functions. Such a topology is called a vector topology and every topological vector space has a uniform topological structure, allowing a notion of uniform convergence and completeness. Some authors also require that the space is a Hausdorff space. One of the most widely studied categories of TVSs are locally convex topological vector spaces. This article focuses on TVSs that are not necessarily locally convex. Banach spaces, Hilbert spaces and Sobolev spaces are other well-known examples of TVSs.
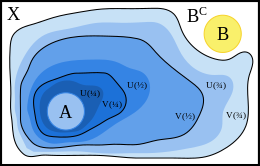
In topology and related branches of mathematics, a normal space is a topological space X that satisfies Axiom T4: every two disjoint closed sets of X have disjoint open neighborhoods. A normal Hausdorff space is also called a T4 space. These conditions are examples of separation axioms and their further strengthenings define completely normal Hausdorff spaces, or T5 spaces, and perfectly normal Hausdorff spaces, or T6 spaces.
In mathematics, a paracompact space is a topological space in which every open cover has an open refinement that is locally finite. These spaces were introduced by Dieudonné (1944). Every compact space is paracompact. Every paracompact Hausdorff space is normal, and a Hausdorff space is paracompact if and only if it admits partitions of unity subordinate to any open cover. Sometimes paracompact spaces are defined so as to always be Hausdorff.
In topology and related branches of mathematics, separated sets are pairs of subsets of a given topological space that are related to each other in a certain way: roughly speaking, neither overlapping nor touching. The notion of when two sets are separated or not is important both to the notion of connected spaces as well as to the separation axioms for topological spaces.

In mathematics, general topology is the branch of topology that deals with the basic set-theoretic definitions and constructions used in topology. It is the foundation of most other branches of topology, including differential topology, geometric topology, and algebraic topology.
In mathematics, the Sierpiński space is a finite topological space with two points, only one of which is closed. It is the smallest example of a topological space which is neither trivial nor discrete. It is named after Wacław Sierpiński.
In topology and related areas of mathematics, a topological property or topological invariant is a property of a topological space that is invariant under homeomorphisms. Alternatively, a topological property is a proper class of topological spaces which is closed under homeomorphisms. That is, a property of spaces is a topological property if whenever a space X possesses that property every space homeomorphic to X possesses that property. Informally, a topological property is a property of the space that can be expressed using open sets.
In topology and related fields of mathematics, a sequential space is a topological space whose topology can be completely characterized by its convergent/divergent sequences. They can be thought of as spaces that satisfy a very weak axiom of countability, and all first-countable spaces are sequential.

In geometry, the hyperplane separation theorem is a theorem about disjoint convex sets in n-dimensional Euclidean space. There are several rather similar versions. In one version of the theorem, if both these sets are closed and at least one of them is compact, then there is a hyperplane in between them and even two parallel hyperplanes in between them separated by a gap. In another version, if both disjoint convex sets are open, then there is a hyperplane in between them, but not necessarily any gap. An axis which is orthogonal to a separating hyperplane is a separating axis, because the orthogonal projections of the convex bodies onto the axis are disjoint.

In topology and other branches of mathematics, a topological space X is locally connected if every point admits a neighbourhood basis consisting entirely of open, connected sets.
In the mathematical field of topology, a hyperconnected space or irreducible space is a topological space X that cannot be written as the union of two proper closed sets. The name irreducible space is preferred in algebraic geometry.
In topology and related areas of mathematics, a subset A of a topological space X is said to be dense in X if every point of X either belongs to A or else is arbitrarily "close" to a member of A — for instance, the rational numbers are a dense subset of the real numbers because every real number either is a rational number or has a rational number arbitrarily close to it. Formally, is dense in if the smallest closed subset of containing is itself.
In the field of topology, a Fréchet–Urysohn space is a topological space with the property that for every subset the closure of in is identical to the sequential closure of in Fréchet–Urysohn spaces are a special type of sequential space.
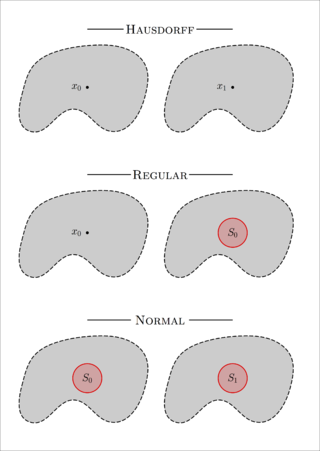
In topology and related fields of mathematics, there are several restrictions that one often makes on the kinds of topological spaces that one wishes to consider. Some of these restrictions are given by the separation axioms. These are sometimes called Tychonoff separation axioms, after Andrey Tychonoff.