In mathematics, the harmonic mean is one of several kinds of average, and in particular, one of the Pythagorean means. It is sometimes appropriate for situations when the average rate is desired.

In numerical analysis, a quadrature rule is an approximation of the definite integral of a function, usually stated as a weighted sum of function values at specified points within the domain of integration. An n-point Gaussian quadrature rule, named after Carl Friedrich Gauss, is a quadrature rule constructed to yield an exact result for polynomials of degree 2n − 1 or less by a suitable choice of the nodes xi and weights wi for i = 1, ..., n. The modern formulation using orthogonal polynomials was developed by Carl Gustav Jacobi in 1826. The most common domain of integration for such a rule is taken as [−1, 1], so the rule is stated as
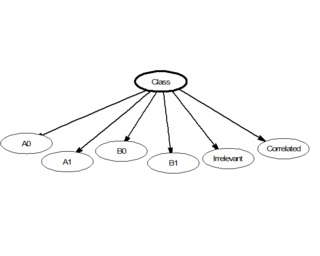
In statistics, naive Bayes classifiers are a family of simple "probabilistic classifiers" based on applying Bayes' theorem with strong (naive) independence assumptions between the features. They are among the simplest Bayesian network models, but coupled with kernel density estimation, they can achieve high accuracy levels.

In transcendental number theory, the Lindemann–Weierstrass theorem is a result that is very useful in establishing the transcendence of numbers. It states the following:

In mathematics, especially in the field of algebra, a polynomial ring or polynomial algebra is a ring formed from the set of polynomials in one or more indeterminates with coefficients in another ring, often a field.

Faà di Bruno's formula is an identity in mathematics generalizing the chain rule to higher derivatives. It is named after Francesco Faà di Bruno, although he was not the first to state or prove the formula. In 1800, more than 50 years before Faà di Bruno, the French mathematician Louis François Antoine Arbogast had stated the formula in a calculus textbook, which is considered to be the first published reference on the subject.
In combinatorial mathematics, the Bell polynomials, named in honor of Eric Temple Bell, are used in the study of set partitions. They are related to Stirling and Bell numbers. They also occur in many applications, such as in the Faà di Bruno's formula.
In commutative algebra and field theory, the Frobenius endomorphism is a special endomorphism of commutative rings with prime characteristic p, an important class which includes finite fields. The endomorphism maps every element to its p-th power. In certain contexts it is an automorphism, but this is not true in general.

In probability and statistics, the Dirichlet distribution (after Peter Gustav Lejeune Dirichlet), often denoted , is a family of continuous multivariate probability distributions parameterized by a vector of positive reals. It is a multivariate generalization of the beta distribution, hence its alternative name of multivariate beta distribution (MBD). Dirichlet distributions are commonly used as prior distributions in Bayesian statistics, and in fact, the Dirichlet distribution is the conjugate prior of the categorical distribution and multinomial distribution.
A bankruptcy problem, also called a claims problem, is a problem of distributing a homogeneous divisible good among people with different claims. The focus is on the case where the amount is insufficient to satisfy all the claims.

Wick's theorem is a method of reducing high-order derivatives to a combinatorics problem. It is named after Italian physicist Gian-Carlo Wick. It is used extensively in quantum field theory to reduce arbitrary products of creation and annihilation operators to sums of products of pairs of these operators. This allows for the use of Green's function methods, and consequently the use of Feynman diagrams in the field under study. A more general idea in probability theory is Isserlis' theorem.
In statistics, the jackknife is a cross-validation technique and, therefore, a form of resampling. It is especially useful for bias and variance estimation. The jackknife pre-dates other common resampling methods such as the bootstrap. Given a sample of size , a jackknife estimator can be built by aggregating the parameter estimates from each subsample of size obtained by omitting one observation.
In mathematics, in the area of additive number theory, the Erdős–Fuchs theorem is a statement about the number of ways that numbers can be represented as a sum of elements of a given additive basis, stating that the average order of this number cannot be too close to being a linear function.
Least-squares support-vector machines (LS-SVM) for statistics and in statistical modeling, are least-squares versions of support-vector machines (SVM), which are a set of related supervised learning methods that analyze data and recognize patterns, and which are used for classification and regression analysis. In this version one finds the solution by solving a set of linear equations instead of a convex quadratic programming (QP) problem for classical SVMs. Least-squares SVM classifiers were proposed by Johan Suykens and Joos Vandewalle. LS-SVMs are a class of kernel-based learning methods.
Transshipment problems form a subgroup of transportation problems, where transshipment is allowed. In transshipment, transportation may or must go through intermediate nodes, possibly changing modes of transport.
Fair river sharing is a kind of a fair division problem in which the waters of a river has to be divided among countries located along the river. It differs from other fair division problems in that the resource to be divided—the water—flows in one direction—from upstream countries to downstream countries. To attain any desired division, it may be required to limit the consumption of upstream countries, but this may require to give these countries some monetary compensation.
Constrained equal awards(CEA), also called constrained equal gains, is a division rule for solving bankruptcy problems. According to this rule, each claimant should receive an equal amount, except that no claimant should receive more than his/her claim. In the context of taxation, it is known as leveling tax.
Constrained equal losses(CEL) is a division rule for solving bankruptcy problems. According to this rule, each claimant should lose an equal amount from his or her claim, except that no claimant should receive a negative amount. In the context of taxation, it is known as poll tax.
The proportional rule is a division rule for solving bankruptcy problems. According to this rule, each claimant should receive an amount proportional to their claim. In the context of taxation, it corresponds to a proportional tax.
A strategic bankruptcy problem is a variant of a bankruptcy problem in which claimants may act strategically, that is, they may manipulate their claims or their behavior. There are various kinds of strategic bankruptcy problems, differing in the assumptions about the possible ways in which claimants may manipulate.














































