
In geometry, an Archimedean solid is one of 13 convex polyhedra whose faces are regular polygons and whose vertices are all symmetric to each other. They were first enumerated by Archimedes. They belong to the class of convex uniform polyhedra, the convex polyhedra with regular faces and symmetric vertices, which is divided into the Archimedean solids, the five Platonic solids, and the two infinite families of prisms and antiprisms. The pseudorhombicuboctahedron is an extra polyhedron with regular faces and congruent vertices, but it is not generally counted as an Archimedean solid because it is not vertex-transitive. An even larger class than the convex uniform polyhedra is the Johnson solids, whose regular polygonal faces do not need to meet in identical vertices.
In geometry, a dodecahedron or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagons as faces, which is a Platonic solid. There are also three regular star dodecahedra, which are constructed as stellations of the convex form. All of these have icosahedral symmetry, order 120.

In geometry, a polyhedron is a three-dimensional shape with flat polygonal faces, straight edges and sharp corners or vertices.

Quilting is the process of joining a minimum of three layers of fabric together either through stitching manually using a needle and thread, or mechanically with a sewing machine or specialised longarm quilting system. An array of stitches is passed through all layers of the fabric to create a three-dimensional padded surface. The three layers are typically referred to as the top fabric or quilt top, batting or insulating material, and the backing.

Patchwork or "pieced work" is a form of needlework that involves sewing together pieces of fabric into a larger design. The larger design is usually based on repeating patterns built up with different fabric shapes. These shapes are carefully measured and cut, basic geometric shapes making them easy to piece together.

A quilt is a multi-layered textile, traditionally composed of two or more layers of fabric or fiber. Commonly three layers are used with a filler material. These layers traditionally include a woven cloth top, a layer of batting or wadding, and a woven back combined using the techniques of quilting. This is the process of sewing on the face of the fabric, and not just the edges, to combine the three layers together to reinforce the material. Stitching patterns can be a decorative element. A single piece of fabric can be used for the top of a quilt, but in many cases the top is created from smaller fabric pieces joined, or patchwork. The pattern and color of these pieces creates the design. Quilts may contain valuable historical information about their creators, "visualizing particular segments of history in tangible, textured ways".

A tessellation or tiling is the covering of a surface, often a plane, using one or more geometric shapes, called tiles, with no overlaps and no gaps. In mathematics, tessellation can be generalized to higher dimensions and a variety of geometries.

A pincushion is a small, stuffed cushion, typically 3–5 cm (1.2–2.0 in) across, which is used in sewing to store pins or needles with their heads protruding to take hold of them easily, collect them, and keep them organized.

Sail components include the features that define a sail's shape and function, plus its constituent parts from which it is manufactured. A sail may be classified in a variety of ways, including by its orientation to the vessel and its shape,. Sails are typically constructed out of flexible material that is shaped by various means, while in use, to offer an appropriate airfoil, according to the strength and apparent direction of the wind. A variety of features and fittings allow the sail to be attached to lines and spars.

A bed sheet is a rectangular piece of cloth used either singly or in a pair as bedding, which is larger in length and width than a mattress, and which is placed immediately above a mattress or bed, but below blankets and other bedding. A bottom sheet is laid above the mattress, and may be either a flat sheet or a fitted sheet. A top sheet, in the many countries where they are used, is a flat sheet, which is placed above a bottom sheet and below other bedding.

A billiard table or billiards table is a bounded table on which cue sports are played. In the modern era, all billiards tables provide a flat surface usually made of quarried slate, that is covered with cloth, and surrounded by vulcanized rubber cushions, with the whole thing elevated above the floor. More specific terms are used for specific sports, such as snooker table and pool table, and different-sized billiard balls are used on these table types. An obsolete term is billiard board, used in the 16th and 17th centuries.
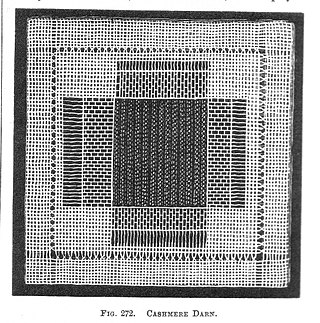
Darning is a sewing technique for repairing holes or worn areas in fabric or knitting using needle and thread alone. It is often done by hand, but using a sewing machine is also possible. Hand darning employs the darning stitch, a simple running stitch in which the thread is "woven" in rows along the grain of the fabric, with the stitcher reversing direction at the end of each row, and then filling in the framework thus created, as if weaving. Darning is a traditional method for repairing fabric damage or holes that do not run along a seam, and where patching is impractical or would create discomfort for the wearer, such as on the heel of a sock.

Korean embroidery techniques and artifacts have a long history, but there is the most evidence from the Joseon Dynasty, after the 14th century in Korea. This article talks about the history, styles, preservation, artists, and examples of screens, costumes, and domestic wares of this exacting and beautiful art form.

Armenian needlelace is a pure form of needle lace made using only a needle, thread and pair of scissors.
The manufacture of textiles is one of the oldest of human technologies. To make textiles, the first requirement is a source of fiber from which a yarn can be made, primarily by spinning. The yarn is processed by knitting or weaving, which turns it into cloth. The machine used for weaving is the loom. For decoration, the process of colouring yarn or the finished material is dyeing. For more information of the various steps, see textile manufacturing.

Cutwork or cut work, also known as punto tagliato in Italian, is a needlework technique in which portions of a textile, typically cotton or linen, are cut away and the resulting "hole" is reinforced and filled with embroidery or needle lace.
Sewing is the craft of fastening or attaching objects using stitches made with needle and thread. Sewing is one of the oldest of the textile arts, arising in the Paleolithic Era. Although usually associated with clothing and household linens, sewing is used in a variety of crafts and industries, including shoemaking, upholstery, sailmaking, bookbinding and the manufacturing of some kinds of sporting goods. Sewing is the fundamental process underlying a variety of textile arts and crafts, including embroidery, tapestry, quilting, appliqué and patchwork.
The Alexandrov uniqueness theorem is a rigidity theorem in mathematics, describing three-dimensional convex polyhedra in terms of the distances between points on their surfaces. It implies that convex polyhedra with distinct shapes from each other also have distinct metric spaces of surface distances, and it characterizes the metric spaces that come from the surface distances on polyhedra. It is named after Soviet mathematician Aleksandr Danilovich Aleksandrov, who published it in the 1940s.

Kuba textiles are a type of raffia cloth unique to the Democratic Republic of the Congo, formerly Zaire, and noted for their elaboration and complexity of design and surface decoration. Most textiles are a variation on rectangular or square pieces of woven palm leaf fiber enhanced by geometric designs executed in linear embroidery and other stitches, which are cut to form pile surfaces resembling velvet. Traditionally, men weave the raffia cloth, and women are responsible for transforming it into various forms of textiles, including ceremonial skirts, ‘velvet’ tribute cloths, headdresses and basketry.

Kazakh clothing, worn by the Kazakh people, is often made of materials suited to the region's extreme climate and the people's nomadic lifestyle. It is commonly decorated with elaborate ornaments made from bird beaks, animal horns, hooves and feet. Although contemporary Kazakhs usually wear Western dress, the Turkic people wear more traditional clothing for holidays and special occasions.
















