Proof
Denote the area of the quadrilateral by K. Then we have

Therefore


The law of cosines implies that

because both sides equal the square of the length of the diagonal BD. This can be rewritten as

Adding this to the above formula for 4K2 yields

Note that:  (a trigonometric identity true for all
(a trigonometric identity true for all  )
)
Following the same steps as in Brahmagupta's formula, this can be written as

Introducing the semiperimeter

the above becomes


and Bretschneider's formula follows after taking the square root of both sides:

The second form is given by using the cosine half-angle identity

yielding

Emmanuel García has used the generalized half angle formulas to give an alternative proof. [1]

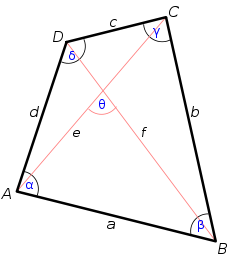
In geometry a quadrilateral is a four-sided polygon, having four edges (sides) and four corners (vertices). The word is derived from the Latin words quadri, a variant of four, and latus, meaning "side". It is also called a tetragon, derived from Greek "tetra" meaning "four" and "gon" meaning "corner" or "angle", in analogy to other polygons. Since "gon" means "angle", it is analogously called a quadrangle, or 4-angle. A quadrilateral with vertices , , and is sometimes denoted as .

In trigonometry, the law of sines, sine law, sine formula, or sine rule is an equation relating the lengths of the sides of any triangle to the sines of its angles. According to the law, where a, b, and c are the lengths of the sides of a triangle, and α, β, and γ are the opposite angles, while R is the radius of the triangle's circumcircle. When the last part of the equation is not used, the law is sometimes stated using the reciprocals; The law of sines can be used to compute the remaining sides of a triangle when two angles and a side are known—a technique known as triangulation. It can also be used when two sides and one of the non-enclosed angles are known. In some such cases, the triangle is not uniquely determined by this data and the technique gives two possible values for the enclosed angle.
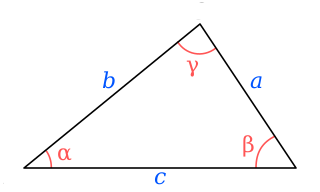
In geometry, Heron's formula gives the area of a triangle in terms of the three side lengths Letting be the semiperimeter of the triangle, the area is
In Euclidean geometry, Brahmagupta's formula, named after the 7th century Indian mathematician, is used to find the area of any convex cyclic quadrilateral given the lengths of the sides. Its generalized version, Bretschneider's formula, can be used with non-cyclic quadrilateral. Heron's formula can be thought as a special case of the Brahmagupta's formula for triangles.

In Euclidean geometry, a cyclic quadrilateral or inscribed quadrilateral is a quadrilateral whose vertices all lie on a single circle. This circle is called the circumcircle or circumscribed circle, and the vertices are said to be concyclic. The center of the circle and its radius are called the circumcenter and the circumradius respectively. Other names for these quadrilaterals are concyclic quadrilateral and chordal quadrilateral, the latter since the sides of the quadrilateral are chords of the circumcircle. Usually the quadrilateral is assumed to be convex, but there are also crossed cyclic quadrilaterals. The formulas and properties given below are valid in the convex case.

In trigonometry, the law of tangents or tangent rule is a statement about the relationship between the tangents of two angles of a triangle and the lengths of the opposing sides.
In geometry, the semiperimeter of a polygon is half its perimeter. Although it has such a simple derivation from the perimeter, the semiperimeter appears frequently enough in formulas for triangles and other figures that it is given a separate name. When the semiperimeter occurs as part of a formula, it is typically denoted by the letter s.
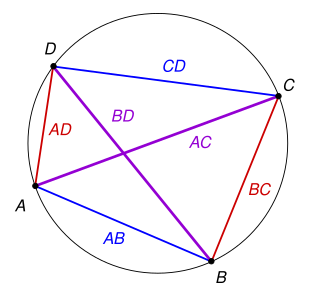
In Euclidean geometry, Ptolemy's theorem is a relation between the four sides and two diagonals of a cyclic quadrilateral. The theorem is named after the Greek astronomer and mathematician Ptolemy. Ptolemy used the theorem as an aid to creating his table of chords, a trigonometric table that he applied to astronomy.
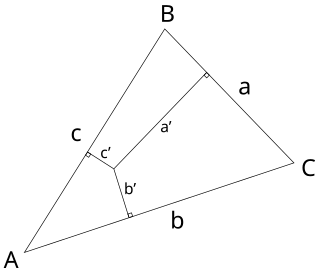
In geometry, the trilinear coordinatesx : y : z of a point relative to a given triangle describe the relative directed distances from the three sidelines of the triangle. Trilinear coordinates are an example of homogeneous coordinates. The ratio x : y is the ratio of the perpendicular distances from the point to the sides opposite vertices A and B respectively; the ratio y : z is the ratio of the perpendicular distances from the point to the sidelines opposite vertices B and C respectively; and likewise for z : x and vertices C and A.
Morrie's law is a special trigonometric identity. Its name is due to the physicist Richard Feynman, who used to refer to the identity under that name. Feynman picked that name because he learned it during his childhood from a boy with the name Morrie Jacobs and afterwards remembered it for all of his life.
In mathematics, the Hadwiger–Finsler inequality is a result on the geometry of triangles in the Euclidean plane. It states that if a triangle in the plane has side lengths a, b and c and area T, then

In mathematics, the Struve functionsHα(x), are solutions y(x) of the non-homogeneous Bessel's differential equation:

In trigonometry, the law of cosines relates the lengths of the sides of a triangle to the cosine of one of its angles. For a triangle with sides and opposite respective angles and , the law of cosines states:
In hyperbolic geometry, the "law of cosines" is a pair of theorems relating the sides and angles of triangles on a hyperbolic plane, analogous to the planar law of cosines from plane trigonometry, or the spherical law of cosines in spherical trigonometry. It can also be related to the relativistic velocity addition formula.

In trigonometry, Mollweide's formula is a pair of relationships between sides and angles in a triangle.
Solution of triangles is the main trigonometric problem of finding the characteristics of a triangle, when some of these are known. The triangle can be located on a plane or on a sphere. Applications requiring triangle solutions include geodesy, astronomy, construction, and navigation.
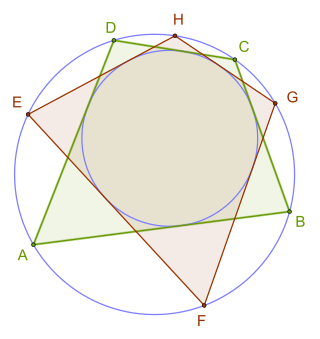
In Euclidean geometry, a bicentric quadrilateral is a convex quadrilateral that has both an incircle and a circumcircle. The radii and centers of these circles are called inradius and circumradius, and incenter and circumcenter respectively. From the definition it follows that bicentric quadrilaterals have all the properties of both tangential quadrilaterals and cyclic quadrilaterals. Other names for these quadrilaterals are chord-tangent quadrilateral and inscribed and circumscribed quadrilateral. It has also rarely been called a double circle quadrilateral and double scribed quadrilateral.

Carl Anton Bretschneider was a mathematician from Gotha, Germany. Bretschneider worked in geometry, number theory, and history of geometry. He also worked on logarithmic integrals and mathematical tables. He was one of the first mathematicians to use the symbol for Euler's constant when he published his 1837 paper. He is best known for his discovery of Bretschneider's formula for the area of a general quadrilateral on a plane,

In trigonometry, the law of cotangents is a relationship among the lengths of the sides of a triangle and the cotangents of the halves of the three angles.