Christopher Cherniak

Last updated

Christopher Cherniak (born 1945) is an American neuroscientist, a member of the University of Maryland Philosophy Department. Cherniak's research trajectory started in theory of knowledge and led into computational neuroanatomy and genomics. The underlying linkage between the areas concerns models of the agent: The work began with more realistic, bounded-resource models of rationality. From this epistemology in turn stemmed a research program concerning optimal-wiring models of global brain and genome anatomy, a structuralist approach.

Contents

Minimal agents

Cherniak's monograph Minimal Rationality states that [1] [2] perhaps the most fundamental psychological law is that humans are finite beings. Bounded-resource models of the agent characterize human rationality as falling between nothing and perfection. The aperçu motivating the rationality critiques is conveyed by the realization that standard idealizations entail some deductive omniscience - for instance, triviality of portions of the deductive sciences. Such ideal agent/logicians, if computational, would have to violate Church's Theorem on undecidability of first-order logic. This insight in turn indicates that NP-completeness is of parallel interest: computational intractability of a cosmos-consuming scale is a practical counterpart to traditional absolute uncomputability - another layer of impossibility for the idealizations. [3] This is part of the philosophical significance of computational complexity. [4] [5]

This research program proceeds from a holistic rather than compartmentalized perspective, where in an inherent ambiguity philosophy and science are distinct but inextricably interconnected. For instance, the classical paradoxes of semantics (e.g., the Liar Paradox) and set theory (e.g., Russell's Paradox) can be reexamined not as inherently contradictory, but instead as signs of use of “quick and dirty heuristics” - that is, speed-reliability tradeoffs of correctness for feasibility. Three disparate fields thereby converge: (a) computational complexity theory, (b) empirical psychology of quick and dirty heuristics, (c) philosophical theory of bounded-resource rationality. In this way, the bounded rationality models serve as a foundation of current “behavioral economics”.

Brainwiring optimization

In addition, it is perhaps natural to extend the bounded-resource approach along these lines down from philosophical rationality to the physical brainwiring hardware level (and its associated organic neuroanatomy). In particular, longrange connectivity is a critically constrained neural resource, with strong evolutionary pressure to employ efficiently. Connection minimization seems a first law of brain tractography, an organizing principle driving neuroanatomy. [6]

The Cherniak laboratory has therefore been gauging how well wiring-optimization ideas from computer microchip design apply to brain structure. "Save wire" turns out to be a strongly predictive model. Wiring minimization can be detected at multiple levels (e.g., placement of the entire brain, layout of its ganglia and/or cortex areas, subcellular architecture of dendrite arbors, etc.). Much of this biological structure seems to arise "for free, directly from physics".

A key specific wiring problem is component placement optimization: Given a set of interconnected components, what are the positionings of the components that minimize total connection costs (e.g., wirelength)? This concept seems to account quite precisely for aspects of neuroanatomy at multiple hierarchical levels. For instance, the nervous system of the roundworm Caenorhabditis elegans includes eleven ganglionic components, which have 11! (~40,000,000) alternative possible anteroposterior orderings. In fact, the actual here is the ideal, in that the actual layout turns out to require the minimum total wirelength, [7] a predictive success story. However, such problems are NP-complete; exact solutions generally appear to entail bruteforce searches, with exponentially exploding costs. Despite local minimum traps, this neuroanatomy optimization can be approximated well by “mesh of springs” energy-minimization mechanisms. [8] (Cf. discussion below of the “genomic bottleneck”.)

A corresponding approach can be applied to placement of interconnected functional areas of cerebral cortex. A first strategy is to use a simpler connection cost measure, conformance of a cortex layout to a wire-saving Adjacency Heuristic: If components are connected, then they are placed adjacent to each other. Sampling of all possible layouts is still required to verify the best ones. For 17 core visual areas of macaque cortex, the actual layout of this subsystem ranks in the top 10−7 layouts best minimizing their adjacency costing. [9] Similar high optimality rankings also hold for the core set of visual areas of cat cortex, and also for rat olfactory cortex and amygdala.

In addition, a “Size Law” appears to apply to systems with such local-global tradeoffs: If a complete system is in fact perfectly optimized, then the smaller the subset of it evaluated by itself, the poorer the optimization tends to appear. (This is a generalization of a related perspective-dependent idea seen in theology, to account for the problem of evil – of apparent imperfections in the Universe with an omnipotent, benevolent deity.) The Size Law applies well to each of the above cortex systems, as well as elsewhere (e.g., for microchip design).

With such a network optimization framework, these “Save wire” results have been extended and replicated for the complete living human cerebrum via fMRI, [10] another predictive success.

Neuron dendrite and axon arbors also appear significantly to approximate minimum-cost Steiner trees. [11] These structures are derivable via fluid dynamics. This seems to constitute some of the most complex biostructure presently obtainable from simple (non-DNA) physical processes. Such a “Physics suffices” account of biological morphogenesis constitutes a “Nongenomic Nativism”. One rationale for the pre-biotic pervading the biotic in this way is to cope with the “genomic bottleneck”: Like other organism systems, the genome has limited capacities. So the more neuroanatomy “for free, directly from physics”, the less the genome information-carrying load.

Another question arises: Neural wiring minimization is of course valuable, but why should it seem to have such a high priority – sometimes apparently near-maximal? The significance of ultra-fine neural optimization remains an open question.

An additional issue concerns how the intentional level of mind meshes with the hardware level of brain. Prima facie, that relationship appears in tension: In some aspects, the brainwiring appears virtually perfectly optimized, yet the rationality has layers of impossibility between it and perfection. [12] Perhaps this is another manifestation of irreducibility of the mental – the well-known poor fit of the two domains with each other.

Genome as nanobrain

A next chapter of this research program: Concepts from the theory of computation can be applied to understand the structure and function of organisms' DNA. The Crick-Watson double-helix model emerged at the same place and time as Turing's final work, namely Cambridge around 1950, so the idea of DNA-as-Turing-machine-tape has floated around for decades.

In particular, the genome can be treated like a "nano-brain” or pico-computer to see whether similar connection minimization strategies also appear in gene networks. As sketched above, for decades, wiring optimization in the brain has been reported that begins to approach some of the most precisely confirmed predictions in neuroscience.

Now a connection-minimization idea is being explored for the human genome. Information transmission may not be cost-free even within a cell, nucleus, or genome. For example, a statistically significant supra-chromosomal homunculus – a global representation of the human body - appears to extend over the entire genome in the nucleus. [13] This is a strategy for connection cost minimization (e.g., cf. body maps reported in sensory and motor cortex since the 19th century). In addition, finer-scale somatotopic mappings seem to occur on individual autosomal chromosomes. [14]

Related Research Articles

<span class="mw-page-title-main">Brain</span> Organ that controls the nervous system in vertebrates and most invertebrates

The brain is an organ that serves as the center of the nervous system in all vertebrate and most invertebrate animals. In vertebrates, a small part of the brain called the hypothalamus is the neural control center for all endocrine systems. The brain is the largest cluster of neurons in the body and is typically located in the head, usually near organs for special senses such as vision, hearing and olfaction. It is the most energy-consuming organ of the body, and the most specialized, responsible for endocrine regulation, sensory perception, motor control, and the development of intelligence.

<span class="mw-page-title-main">Cognitive science</span> Interdisciplinary scientific study of cognitive processes

Cognitive science is the interdisciplinary, scientific study of the mind and its processes with input from linguistics, psychology, neuroscience, philosophy, computer science/artificial intelligence, and anthropology. It examines the nature, the tasks, and the functions of cognition. Cognitive scientists study intelligence and behavior, with a focus on how nervous systems represent, process, and transform information. Mental faculties of concern to cognitive scientists include language, perception, memory, attention, reasoning, and emotion; to understand these faculties, cognitive scientists borrow from fields such as linguistics, psychology, artificial intelligence, philosophy, neuroscience, and anthropology. The typical analysis of cognitive science spans many levels of organization, from learning and decision to logic and planning; from neural circuitry to modular brain organization. One of the fundamental concepts of cognitive science is that "thinking can best be understood in terms of representational structures in the mind and computational procedures that operate on those structures."

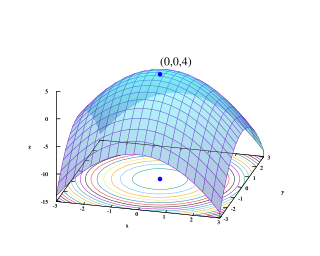
<span class="mw-page-title-main">Mathematical optimization</span> Study of mathematical algorithms for optimization problems

Mathematical optimization or mathematical programming is the selection of a best element, with regard to some criterion, from some set of available alternatives. It is generally divided into two subfields: discrete optimization and continuous optimization. Optimization problems arise in all quantitative disciplines from computer science and engineering to operations research and economics, and the development of solution methods has been of interest in mathematics for centuries.

<span class="mw-page-title-main">Neuropil</span> Type of area in the nervous system

Neuropil is any area in the nervous system composed of mostly unmyelinated axons, dendrites and glial cell processes that forms a synaptically dense region containing a relatively low number of cell bodies. The most prevalent anatomical region of neuropil is the brain which, although not completely composed of neuropil, does have the largest and highest synaptically concentrated areas of neuropil in the body. For example, the neocortex and olfactory bulb both contain neuropil.

Bounded rationality is the idea that rationality is limited when individuals make decisions, and under these limitations, rational individuals will select a decision that is satisfactory rather than optimal.

Computational neuroscience is a branch of neuroscience which employs mathematics, computer science, theoretical analysis and abstractions of the brain to understand the principles that govern the development, structure, physiology and cognitive abilities of the nervous system.

In cognitive psychology, information processing is an approach to the goal of understanding human thinking that treats cognition as essentially computational in nature, with the mind being the software and the brain being the hardware. It arose in the 1940s and 1950s, after World War II. The information processing approach in psychology is closely allied to the computational theory of mind in philosophy; it is also related to cognitivism in psychology and functionalism in philosophy.

Neurophilosophy or philosophy of neuroscience is the interdisciplinary study of neuroscience and philosophy that explores the relevance of neuroscientific studies to the arguments traditionally categorized as philosophy of mind. The philosophy of neuroscience attempts to clarify neuroscientific methods and results using the conceptual rigor and methods of philosophy of science.

<span class="mw-page-title-main">Thalamocortical radiations</span> Neural pathways between the thalamus and cerebral cortex

In neuroanatomy, thalamocortical radiations, also known as thalamocortical fibres, are the efferent fibres that project from the thalamus to distinct areas of the cerebral cortex. They form fibre bundles that emerge from the lateral surface of the thalamus.

Motor control is the regulation of movements in organisms that possess a nervous system. Motor control includes conscious voluntary movements, subconscious muscle memory and involuntary reflexes, as well as instinctual taxis.

In mathematical optimization, the ellipsoid method is an iterative method for minimizing convex functions over convex sets. The ellipsoid method generates a sequence of ellipsoids whose volume uniformly decreases at every step, thus enclosing a minimizer of a convex function.

Group method of data handling (GMDH) is a family of inductive algorithms for computer-based mathematical modeling of multi-parametric datasets that features fully automatic structural and parametric optimization of models.

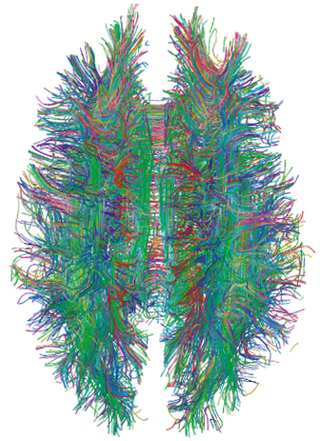
<span class="mw-page-title-main">Connectome</span> Comprehensive map of neural connections in the brain

A connectome is a comprehensive map of neural connections in the brain, and may be thought of as its "wiring diagram". An organism's nervous system is made up of neurons which communicate through synapses. A connectome is constructed by tracing the neuron in a nervous system and mapping where neurons are connected through synapses.

Visual perception is the ability to interpret the surrounding environment through photopic vision, color vision, scotopic vision, and mesopic vision, using light in the visible spectrum reflected by objects in the environment. This is different from visual acuity, which refers to how clearly a person sees. A person can have problems with visual perceptual processing even if they have 20/20 vision.

Natural computing, also called natural computation, is a terminology introduced to encompass three classes of methods: 1) those that take inspiration from nature for the development of novel problem-solving techniques; 2) those that are based on the use of computers to synthesize natural phenomena; and 3) those that employ natural materials to compute. The main fields of research that compose these three branches are artificial neural networks, evolutionary algorithms, swarm intelligence, artificial immune systems, fractal geometry, artificial life, DNA computing, and quantum computing, among others.

The network of the human nervous system is composed of nodes that are connected by links. The connectivity may be viewed anatomically, functionally, or electrophysiologically. These are presented in several Wikipedia articles that include Connectionism, Biological neural network, Artificial neural network, Computational neuroscience, as well as in several books by Ascoli, G. A. (2002), Sterratt, D., Graham, B., Gillies, A., & Willshaw, D. (2011), Gerstner, W., & Kistler, W. (2002), and Rumelhart, J. L., McClelland, J. L., and PDP Research Group (1986) among others. The focus of this article is a comprehensive view of modeling a neural network. Once an approach based on the perspective and connectivity is chosen, the models are developed at microscopic, mesoscopic, or macroscopic (system) levels. Computational modeling refers to models that are developed using computing tools.

In neuroscience and motor control, the degrees of freedom problem or motor equivalence problem states that there are multiple ways for humans or animals to perform a movement in order to achieve the same goal. In other words, under normal circumstances, no simple one-to-one correspondence exists between a motor problem and a motor solution to the problem. The motor equivalence problem was first formulated by the Russian neurophysiologist Nikolai Bernstein: "It is clear that the basic difficulties for co-ordination consist precisely in the extreme abundance of degrees of freedom, with which the [nervous] centre is not at first in a position to deal."

The free energy principle is a theoretical framework suggesting that the brain reduces surprise or uncertainty by making predictions based on internal models and updating them using sensory input. It highlights the brain's objective of aligning its internal model with the external world to enhance prediction accuracy. This principle integrates Bayesian inference with active inference, where actions are guided by predictions and sensory feedback refines them. It has wide-ranging implications for comprehending brain function, perception, and action.

Rational analysis is a theoretical framework, methodology, and research program in cognitive science that has been developed by John Anderson. The goal of rational analysis as a research program is to explain the function and purpose of cognitive processes and to discover the structure of the mind. Chater and Oaksford contrast it with the mechanistic explanations of cognition offered by both computational models and neuroscience.

In neuroscience, predictive coding is a theory of brain function which postulates that the brain is constantly generating and updating a "mental model" of the environment. According to the theory, such a mental model is used to predict input signals from the senses that are then compared with the actual input signals from those senses. With the rising popularity of representation learning, the theory is being actively pursued and applied in machine learning and related fields.

References

  1. ↑ Cherniak, Christopher (1986). Minimal rationality . MIT Press. ISBN   978-0-262-03122-6.
  2. ↑ Cherniak, Christopher (2009). Saisho Gorisei. Translated by Shibata, M. Keiso Shobo. ISBN   978-4-326-19953-2.
  3. ↑ Cherniak, Christopher (1984). "Computational complexity and the universal acceptance of logic". Journal of Philosophy. 81 (12): 739–758. doi:10.2307/2026030. JSTOR   2026030.
  4. ↑ Aaronson, S (2013). "Why philosophers should care about computational complexity". In Copeland, J; Posy, C; Shagrir, O (eds.). Computability: Turing, Gödel, Church, & Beyond. MIT Press. pp. 261–327. ISBN   978-0262527484.
  5. ↑ Dean, S W (2016). "Computational complexity theory". In Zalta, E (ed.). Stanford Encyclopedia of Philosophy. MIT Press. pp. 261–327. ISBN   978-0262527484.
  6. ↑ Cherniak, Christopher (1994). "Philosophy and computational neuroanatomy". Philosophical Studies. 73 (2–3): 89–107. doi:10.1007/bf01207659. JSTOR   4320464. S2CID   170744521.
  7. ↑ Cherniak, Christopher (1994). "Component placement optimization in the brain". J. Neurosci. 14 (4): 2418–2427. doi: 10.1523/JNEUROSCI.14-04-02418.1994 . PMC   6577144 . PMID   8158278.
  8. ↑ Cherniak, Christopher; Mokhtarzada, Z; Nodelman, U (2002). "Optimal-wiring models of neuroanatomy". Computational Neuroanatomy: Principles and Methods. Humana Press. pp. 71–82. ISBN   978-1-58829-000-7.
  9. ↑ Cherniak, Christopher; Mokhtarzada, Z; Rodriguez-Esteban, R; Changizi, B (2004). "Global optimization of cerebral cortex layout". Proc. Natl. Acad. Sci. U.S.A. 101 (4): 1081–1086. Bibcode:2004PNAS..101.1081C. doi: 10.1073/pnas.0305212101 . PMC   327154 . PMID   14722353.
  10. ↑ Lewis, S; Christova, P; Jerde, T; Georgopoulos, A (2012). "A compact and realistic cerebral cortical layout derived from prewhitened resting-state fMRI time series: Cherniak's adjacency rule, size law, and metamodule grouping upheld". Front. Neuroanat. 6: 36. doi: 10.3389/fnana.2012.00036 . PMC   3434448 . PMID   22973198.
  11. ↑ Cherniak, Christopher; Changizi, M; Kang, Du Won (1999). "Large-scale optimization of neuron arbors". Physical Review E. 59 (5): 6001–6009. Bibcode:1999PhRvE..59.6001C. doi:10.1103/physreve.59.6001. PMID   11969583.
  12. ↑ Cherniak, Christopher (2009). "Minimal rationality and optimal brain wiring". In Glymour, C; Wei, W; Westerstahl, D (eds.). Logic, Methodology and Philosophy of Science: Proceedings of 13th International Congress. College Publications. pp. 443–454. ISBN   978-1904987451.
  13. ↑ Cherniak, Christopher; Rodriguez-Esteban, Raul (2013). "Body maps on the human genome". Mol. Cytogenet. 6 (1): 61. doi: 10.1186/1755-8166-6-61 . PMC   3905923 . PMID   24354739.
  14. ↑ Cherniak, Christopher; Rodriguez-Esteban, Raul (2015). "Body maps on human chromosomes". UMIACS Tech Report: 2015–04. doi:10.13016/M2MM73. hdl:1903/17177.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.