Mathematical definition
Given the production function then the elasticity of complementarity is defined as
The inverse of elasticity of complementarity is elasticity of substitution.
Elasticity of complementarity (Hamermesh, 1993) is the percentage responsiveness of relative factor prices to a 1 percent change in relative inputs.
Given the production function then the elasticity of complementarity is defined as
The inverse of elasticity of complementarity is elasticity of substitution.
In calculus, the chain rule is a formula to compute the derivative of a composite function. That is, if f and g are differentiable functions, then the chain rule expresses the derivative of their composite f∘g — the function which maps x to — in terms of the derivatives of f and g and the product of functions as follows:

The derivative of a function of a real variable measures the sensitivity to change of the function value with respect to a change in its argument. Derivatives are a fundamental tool of calculus. For example, the derivative of the position of a moving object with respect to time is the object's velocity: this measures how quickly the position of the object changes when time advances.
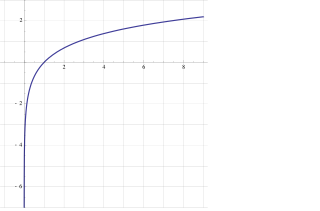
The natural logarithm of a number is its logarithm to the base of the mathematical constant e, where e is an irrational and transcendental number approximately equal to 2.718281828459. The natural logarithm of x is generally written as ln x, logex, or sometimes, if the base e is implicit, simply log x. Parentheses are sometimes added for clarity, giving ln(x), loge(x), or log(x). This is done in particular when the argument to the logarithm is not a single symbol, to prevent ambiguity.
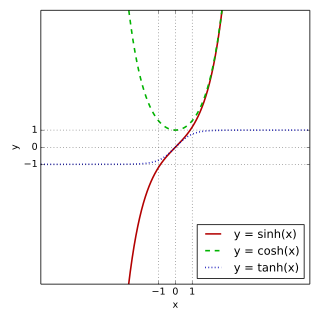
In mathematics, hyperbolic functions are analogs of the ordinary trigonometric functions defined for the hyperbola rather than on the circle: just as the points (cos t, sin t) form a circle with a unit radius, the points (cosh t, sinh t) form the right half of the equilateral hyperbola.
In calculus, the power rule is used to differentiate functions of the form , whenever is a real number. Since differentiation is a linear operation on the space of differentiable functions, polynomials can also be differentiated using this rule. The power rule underlies the Taylor series as it relates a power series with a function's derivatives.
In calculus, the indefinite integral of a given function on a connected domain is only defined up to an additive constant, the constant of integration. This constant expresses an ambiguity inherent in the construction of antiderivatives. If a function is defined on an interval and is an antiderivative of , then the set of all antiderivatives of is given by the functions , where C is an arbitrary constant. The constant of integration is sometimes omitted in lists of integrals for simplicity.

In mathematics, a multiplicative inverse or reciprocal for a number x, denoted by 1/x or x−1, is a number which when multiplied by x yields the multiplicative identity, 1. The multiplicative inverse of a fraction a/b is b/a. For the multiplicative inverse of a real number, divide 1 by the number. For example, the reciprocal of 5 is one fifth, and the reciprocal of 0.25 is 1 divided by 0.25, or 4. The reciprocal function, the function f(x) that maps x to 1/x, is one of the simplest examples of a function which is its own inverse.
Integration is the basic operation in integral calculus. While differentiation has straightforward rules by which the derivative of a complicated function can be found by differentiating its simpler component functions, integration does not, so tables of known integrals are often useful. This page lists some of the most common antiderivatives.

In mathematics, trigonometric substitution is the substitution of trigonometric functions for other expressions. In calculus, trigonometric substitution is a technique for evaluating integrals. Moreover, one may use the trigonometric identities to simplify certain integrals containing radical expressions.
In mathematics, and in particular functional analysis, the shift operator also known as translation operator is an operator that takes a function x ↦ f(x) to its translationx ↦ f(x + a). In time series analysis, the shift operator is called the lag operator.
In mathematics, specifically in calculus and complex analysis, the logarithmic derivative of a function f is defined by the formula
In mathematics, an Euler–Cauchy equation, or Cauchy–Euler equation, or simply Euler's equation is a linear homogeneous ordinary differential equation with variable coefficients. It is sometimes referred to as an equidimensional equation. Because of its particularly simple equidimensional structure the differential equation can be solved explicitly.
In mathematics, there are several integrals known as the Dirichlet integral, after the German mathematician Peter Gustav Lejeune Dirichlet.
In calculus, Leibniz's rule for differentiation under the integral sign, named after Gottfried Leibniz, states that for an integral of the form
Elasticity of substitution is the elasticity of the ratio of two inputs to a production function with respect to the ratio of their marginal products. In a competitive market, it measures the percentage change in the ratio of two inputs used in response to a percentage change in their prices. It measures the curvature of an isoquant and thus, the substitutability between inputs, i.e. how easy it is to substitute one input for the other.
In mathematics, the elasticity or point elasticity of a positive differentiable function f of a positive variable at point a is defined as
This is a summary of differentiation rules, that is, rules for computing the derivative of a function in calculus.
In calculus, the differential represents the principal part of the change in a function y = f(x) with respect to changes in the independent variable. The differential dy is defined by
In mathematical economics, an isoelastic function, sometimes constant elasticity function, is a function that exhibits a constant elasticity, i.e. has a constant elasticity coefficient. The elasticity is the ratio of the percentage change in the dependent variable to the percentage causative change in the independent variable, in the limit as the changes approach zero in magnitude.
| This economics-related article is a stub. You can help Wikipedia by expanding it. |