In algebraic geometry, an exotic affine space is a complex algebraic variety that is diffeomorphic to for some n, but is not isomorphic as an algebraic variety to . [1] [2] [3] An example of an exotic is the Koras–Russell cubic threefold, [4] which is the subset of defined by the polynomial equation
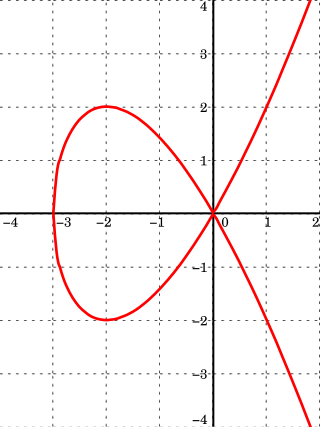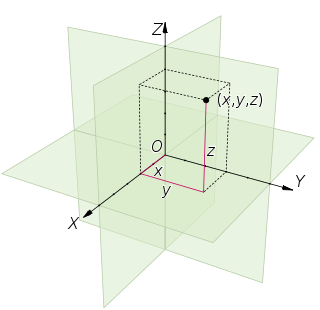
Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, that is, in Euclid's Elements, it was the three-dimensional space of Euclidean geometry, but in modern mathematics there are Euclidean spaces of any positive integer dimension n, which are called Euclidean n-spaces when one wants to specify their dimension. For n equal to one or two, they are commonly called respectively Euclidean lines and Euclidean planes. The qualifier "Euclidean" is used to distinguish Euclidean spaces from other spaces that were later considered in physics and modern mathematics.
In commutative algebra, the prime spectrum of a ring R is the set of all prime ideals of R, and is usually denoted by ; in algebraic geometry it is simultaneously a topological space equipped with the sheaf of rings .
In mathematics, Hilbert's Nullstellensatz is a theorem that establishes a fundamental relationship between geometry and algebra. This relationship is the basis of algebraic geometry. It relates algebraic sets to ideals in polynomial rings over algebraically closed fields. This relationship was discovered by David Hilbert, who proved the Nullstellensatz in his second major paper on invariant theory in 1893.
In mathematics, complex geometry is the study of geometric structures and constructions arising out of, or described by, the complex numbers. In particular, complex geometry is concerned with the study of spaces such as complex manifolds and complex algebraic varieties, functions of several complex variables, and holomorphic constructions such as holomorphic vector bundles and coherent sheaves. Application of transcendental methods to algebraic geometry falls in this category, together with more geometric aspects of complex analysis.
In mathematics, the Hodge conjecture is a major unsolved problem in algebraic geometry and complex geometry that relates the algebraic topology of a non-singular complex algebraic variety to its subvarieties.
In mathematics, an algebraic group is an algebraic variety endowed with a group structure that is compatible with its structure as an algebraic variety. Thus the study of algebraic groups belongs both to algebraic geometry and group theory.
Algebraic varieties are the central objects of study in algebraic geometry, a sub-field of mathematics. Classically, an algebraic variety is defined as the set of solutions of a system of polynomial equations over the real or complex numbers. Modern definitions generalize this concept in several different ways, while attempting to preserve the geometric intuition behind the original definition.
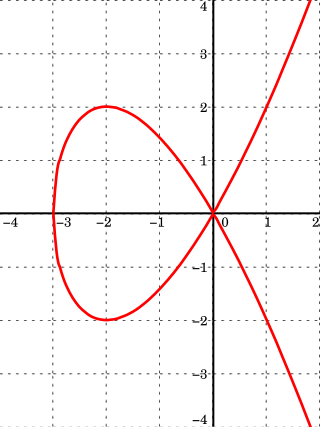
In mathematics, an affine algebraic plane curve is the zero set of a polynomial in two variables. A projective algebraic plane curve is the zero set in a projective plane of a homogeneous polynomial in three variables. An affine algebraic plane curve can be completed in a projective algebraic plane curve by homogenizing its defining polynomial. Conversely, a projective algebraic plane curve of homogeneous equation h(x, y, t) = 0 can be restricted to the affine algebraic plane curve of equation h(x, y, 1) = 0. These two operations are each inverse to the other; therefore, the phrase algebraic plane curve is often used without specifying explicitly whether it is the affine or the projective case that is considered.
In mathematics, an affine space is a geometric structure that generalizes some of the properties of Euclidean spaces in such a way that these are independent of the concepts of distance and measure of angles, keeping only the properties related to parallelism and ratio of lengths for parallel line segments.
In algebraic geometry, a projective variety over an algebraically closed field k is a subset of some projective n-space over k that is the zero-locus of some finite family of homogeneous polynomials of n + 1 variables with coefficients in k, that generate a prime ideal, the defining ideal of the variety. Equivalently, an algebraic variety is projective if it can be embedded as a Zariski closed subvariety of .
In mathematics, a scheme is a mathematical structure that enlarges the notion of algebraic variety in several ways, such as taking account of multiplicities and allowing "varieties" defined over any commutative ring.
In mathematics, birational geometry is a field of algebraic geometry in which the goal is to determine when two algebraic varieties are isomorphic outside lower-dimensional subsets. This amounts to studying mappings that are given by rational functions rather than polynomials; the map may fail to be defined where the rational functions have poles.
Affine geometry, broadly speaking, is the study of the geometrical properties of lines, planes, and their higher dimensional analogs, in which a notion of "parallel" is retained, but no metrical notions of distance or angle are. Affine spaces differ from linear spaces in that they do not have a distinguished choice of origin. So, in the words of Marcel Berger, "An affine space is nothing more than a vector space whose origin we try to forget about, by adding translations to the linear maps." Accordingly, a complex affine space, that is an affine space over the complex numbers, is like a complex vector space, but without a distinguished point to serve as the origin.
In differential geometry, a hypercomplex manifold is a manifold with the tangent bundle equipped with an action by the algebra of quaternions in such a way that the quaternions define integrable almost complex structures.
In mathematics, and in particular differential geometry and complex geometry, a complex analytic variety or complex analytic space is a generalization of a complex manifold which allows the presence of singularities. Complex analytic varieties are locally ringed spaces which are locally isomorphic to local model spaces, where a local model space is an open subset of the vanishing locus of a finite set of holomorphic functions.
In algebraic geometry, the Koras–Russell cubic threefolds are smooth affine complex threefolds diffeomorphic to studied by Koras & Russell (1997). They have a hyperbolic action of a one-dimensional torus with a unique fixed point, such that the quotients of the threefold and the tangent space of the fixed point by this action are isomorphic. They were discovered in the process of proving the Linearization Conjecture in dimension 3. A linear action of on the affine space is one of the form , where and . The Linearization Conjecture in dimension says that every algebraic action of on the complex affine space is linear in some algebraic coordinates on . M. Koras and P. Russell made a key step towards the solution in dimension 3, providing a list of threefolds and proving that the Linearization Conjecture for holds if all those threefolds are exotic affine 3-spaces, that is, none of them is isomorphic to . This was later shown by Kaliman and Makar-Limanov using the ML-invariant of an affine variety, which had been invented exactly for this purpose.
This is a glossary of algebraic geometry.
In mathematics, a derivation of a commutative ring is called a locally nilpotent derivation (LND) if every element of is annihilated by some power of .
In mathematics, the automorphism group of an object X is the group consisting of automorphisms of X under composition of morphisms. For example, if X is a finite-dimensional vector space, then the automorphism group of X is the group of invertible linear transformations from X to itself. If instead X is a group, then its automorphism group is the group consisting of all group automorphisms of X.
In mathematics, mirror symmetry is a conjectural relationship between certain Calabi–Yau manifolds and a constructed "mirror manifold". The conjecture allows one to relate the number of rational curves on a Calabi-Yau manifold to integrals from a family of varieties. In short, this means there is a relation between the number of genus algebraic curves of degree on a Calabi-Yau variety and integrals on a dual variety . These relations were original discovered by Candelas, de la Ossa, Green, and Parkes in a paper studying a generic quintic threefold in as the variety and a construction from the quintic Dwork family giving . Shortly after, Sheldon Katz wrote a summary paper outlining part of their construction and conjectures what the rigorous mathematical interpretation could be.
 | This geometry-related article is a stub. You can help Wikipedia by expanding it. |
