Related Research Articles
Combinatorics is an area of mathematics primarily concerned with counting, both as a means and as an end to obtaining results, and certain properties of finite structures. It is closely related to many other areas of mathematics and has many applications ranging from logic to statistical physics and from evolutionary biology to computer science.

Discrete mathematics is the study of mathematical structures that can be considered "discrete" rather than "continuous". Objects studied in discrete mathematics include integers, graphs, and statements in logic. By contrast, discrete mathematics excludes topics in "continuous mathematics" such as real numbers, calculus or Euclidean geometry. Discrete objects can often be enumerated by integers; more formally, discrete mathematics has been characterized as the branch of mathematics dealing with countable sets. However, there is no exact definition of the term "discrete mathematics".

Differential geometry is a mathematical discipline that studies the geometry of smooth shapes and smooth spaces, otherwise known as smooth manifolds. It uses the techniques of differential calculus, integral calculus, linear algebra and multilinear algebra. The field has its origins in the study of spherical geometry as far back as antiquity. It also relates to astronomy, the geodesy of the Earth, and later the study of hyperbolic geometry by Lobachevsky. The simplest examples of smooth spaces are the plane and space curves and surfaces in the three-dimensional Euclidean space, and the study of these shapes formed the basis for development of modern differential geometry during the 18th and 19th centuries.

Euclidean geometry is a mathematical system attributed to ancient Greek mathematician Euclid, which he described in his textbook on geometry, Elements. Euclid's approach consists in assuming a small set of intuitively appealing axioms (postulates) and deducing many other propositions (theorems) from these. Although many of Euclid's results had been stated earlier, Euclid was the first to organize these propositions into a logical system in which each result is proved from axioms and previously proved theorems.

A shape is a graphical representation of an object's form or its external boundary, outline, or external surface. It is distinct from other object properties, such as color, texture, or material type. In geometry, shape excludes information about the object's position, size, orientation and chirality. A figure is a representation including both shape and size.
In mathematics, projective geometry is the study of geometric properties that are invariant with respect to projective transformations. This means that, compared to elementary Euclidean geometry, projective geometry has a different setting and a selective set of basic geometric concepts. The basic intuitions are that projective space has more points than Euclidean space, for a given dimension, and that geometric transformations are permitted that transform the extra points to Euclidean points, and vice versa.
Synthetic geometry is geometry without the use of coordinates. It relies on the axiomatic method for proving all results from a few basic properties initially called postulates, and at present called axioms.
In mathematics, integral geometry is the theory of measures on a geometrical space invariant under the symmetry group of that space. In more recent times, the meaning has been broadened to include a view of invariant transformations from the space of functions on one geometrical space to the space of functions on another geometrical space. Such transformations often take the form of integral transforms such as the Radon transform and its generalizations.
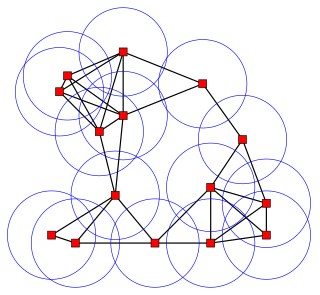
Discrete geometry and combinatorial geometry are branches of geometry that study combinatorial properties and constructive methods of discrete geometric objects. Most questions in discrete geometry involve finite or discrete sets of basic geometric objects, such as points, lines, planes, circles, spheres, polygons, and so forth. The subject focuses on the combinatorial properties of these objects, such as how they intersect one another, or how they may be arranged to cover a larger object.

A spatial network is a graph in which the vertices or edges are spatial elements associated with geometric objects, i.e., the nodes are located in a space equipped with a certain metric. The simplest mathematical realization of spatial network is a lattice or a random geometric graph, where nodes are distributed uniformly at random over a two-dimensional plane; a pair of nodes are connected if the Euclidean distance is smaller than a given neighborhood radius. Transportation and mobility networks, Internet, mobile phone networks, power grids, social and contact networks and biological neural networks are all examples where the underlying space is relevant and where the graph's topology alone does not contain all the information. Characterizing and understanding the structure, resilience and the evolution of spatial networks is crucial for many different fields ranging from urbanism to epidemiology.

In mathematics, a space is a set endowed with a structure defining the relationships among the elements of the set. A subspace is a subset of the parent space which retains the same structure. While modern mathematics uses many types of spaces, such as Euclidean spaces, linear spaces, topological spaces, Hilbert spaces, or probability spaces, it does not define the notion of "space" itself.
Geometry is a branch of mathematics concerned with properties of space such as the distance, shape, size, and relative position of figures. Geometry is, along with arithmetic, one of the oldest branches of mathematics. A mathematician who works in the field of geometry is called a geometer. Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point, line, plane, distance, angle, surface, and curve, as fundamental concepts.
Foundations of geometry is the study of geometries as axiomatic systems. There are several sets of axioms which give rise to Euclidean geometry or to non-Euclidean geometries. These are fundamental to the study and of historical importance, but there are a great many modern geometries that are not Euclidean which can be studied from this viewpoint. The term axiomatic geometry can be applied to any geometry that is developed from an axiom system, but is often used to mean Euclidean geometry studied from this point of view. The completeness and independence of general axiomatic systems are important mathematical considerations, but there are also issues to do with the teaching of geometry which come into play.
In mathematics, stochastic geometry is the study of random spatial patterns. At the heart of the subject lies the study of random point patterns. This leads to the theory of spatial point processes, hence notions of Palm conditioning, which extend to the more abstract setting of random measures.
Edgar Nelson Gilbert was an American mathematician and coding theorist, a longtime researcher at Bell Laboratories whose accomplishments include the Gilbert–Varshamov bound in coding theory, the Gilbert–Elliott model of bursty errors in signal transmission, and the Erdős–Rényi model for random graphs.
In mathematics and probability theory, continuum percolation theory is a branch of mathematics that extends discrete percolation theory to continuous space. More specifically, the underlying points of discrete percolation form types of lattices whereas the underlying points of continuum percolation are often randomly positioned in some continuous space and form a type of point process. For each point, a random shape is frequently placed on it and the shapes overlap each with other to form clumps or components. As in discrete percolation, a common research focus of continuum percolation is studying the conditions of occurrence for infinite or giant components. Other shared concepts and analysis techniques exist in these two types of percolation theory as well as the study of random graphs and random geometric graphs.
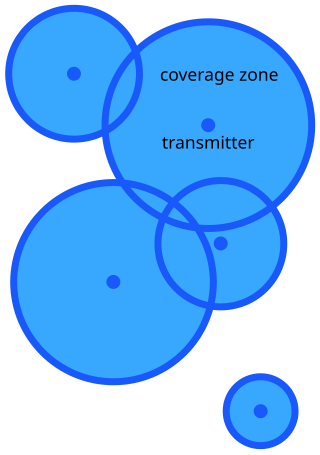
In mathematics and telecommunications, stochastic geometry models of wireless networks refer to mathematical models based on stochastic geometry that are designed to represent aspects of wireless networks. The related research consists of analyzing these models with the aim of better understanding wireless communication networks in order to predict and control various network performance metrics. The models require using techniques from stochastic geometry and related fields including point processes, spatial statistics, geometric probability, percolation theory, as well as methods from more general mathematical disciplines such as geometry, probability theory, stochastic processes, queueing theory, information theory, and Fourier analysis.

In probability theory, statistics and related fields, a Poisson point process is a type of mathematical object that consists of points randomly located on a mathematical space with the essential feature that the points occur independently of one another. The process's name derives from the fact that the number of points in any given finite region follows a Poisson distribution. The process and the distribution are named after French mathematician Siméon Denis Poisson. The process itself was discovered independently and repeatedly in several settings, including experiments on radioactive decay, telephone call arrivals and actuarial science.
Ilona Palásti (1924–1991) was a Hungarian mathematician who worked at the Alfréd Rényi Institute of Mathematics. She is known for her research in discrete geometry, geometric probability, and the theory of random graphs. With Alfréd Rényi and others, she was considered to be one of the members of the Hungarian School of Probability.
References
- ↑ Herbert Solomon (1978). Geometric Probability. Philadelphia, PA: Society for Industrial and Applied Mathematics.
- Daniel A. Klain, Gian-Carlo Rota, Introduction to Geometric Probability.
- Maurice G. Kendall, Patrick A. P. Moran, Geometrical Probability.
- Eugene Seneta, Karen Hunger Parshall, François Jongmans, "Nineteenth-Century Developments in Geometric Probability: J. J. Sylvester, M. W. Crofton, J.-É. Barbier, and J. Bertrand"