Related Research Articles
In statistical physics and mathematics, percolation theory describes the behavior of a network when nodes or links are added. This is a geometric type of phase transition, since at a critical fraction of addition the network of small, disconnected clusters merge into significantly larger connected, so-called spanning clusters. The applications of percolation theory to materials science and in many other disciplines are discussed here and in the articles network theory and percolation.
Hydrothermal circulation in its most general sense is the circulation of hot water. Hydrothermal circulation occurs most often in the vicinity of sources of heat within the Earth's crust. In general, this occurs near volcanic activity, but can occur in the shallow to mid crust along deeply penetrating fault irregularities or in the deep crust related to the intrusion of granite, or as the result of orogeny or metamorphism.
Percolation, in physics, chemistry and materials science, refers to the movement and filtering of fluids through porous materials. It is described by Darcy's law. Broader applications have since been developed that cover connectivity of many systems modeled as lattices or graphs, analogous to connectivity of lattice components in the filtration problem that modulates capacity for percolation.
James Harvie Wilkinson III is an American jurist who serves as a United States circuit judge on the United States Court of Appeals for the Fourth Circuit. His name has been raised at several junctures in the past as a possible nominee to the United States Supreme Court.
Wetting is the ability of a liquid to maintain contact with a solid surface, resulting from intermolecular interactions when the two are brought together. This happens in presence of a gaseous phase or another liquid phase not miscible with the first one. The degree of wetting (wettability) is determined by a force balance between adhesive and cohesive forces.
Critical exponents describe the behavior of physical quantities near continuous phase transitions. It is believed, though not proven, that they are universal, i.e. they do not depend on the details of the physical system, but only on some of its general features. For instance, for ferromagnetic systems, the critical exponents depend only on:
Imbibition is a special type of diffusion that takes place when liquid is absorbed by solids-colloids causing an increase in volume. Water surface potential movement takes place along a concentration gradient; some dry materials absorb water. A gradient between the absorbent and the liquid is essential for imbibition. For a substance to imbibe a liquid, there must first be some attraction between them. Imbibition occurs when a wetting fluid displaces a non-wetting fluid, the opposite of drainage in which a non-wetting phase displaces the wetting fluid. The two processes are governed by different mechanisms. Imbibition is also a type of diffusion since water movement is along the concentration gradient. Seeds and other such materials have almost no water hence they absorb water easily. Water potential gradient between the absorbent and liquid imbibed is essential for imbibition.
In fluid statics, capillary pressure is the pressure between two immiscible fluids in a thin tube, resulting from the interactions of forces between the fluids and solid walls of the tube. Capillary pressure can serve as both an opposing or driving force for fluid transport and is a significant property for research and industrial purposes. It is also observed in natural phenomena.
In fluid dynamics, the Buckley–Leverett equation is a conservation equation used to model two-phase flow in porous media. The Buckley–Leverett equation or the Buckley–Leverett displacement describes an immiscible displacement process, such as the displacement of oil by water, in a one-dimensional or quasi-one-dimensional reservoir. This equation can be derived from the mass conservation equations of two-phase flow, under the assumptions listed below.
In physics, the Young–Laplace equation is an algebraic equation that describes the capillary pressure difference sustained across the interface between two static fluids, such as water and air, due to the phenomenon of surface tension or wall tension, although use of the latter is only applicable if assuming that the wall is very thin. The Young–Laplace equation relates the pressure difference to the shape of the surface or wall and it is fundamentally important in the study of static capillary surfaces. It's a statement of normal stress balance for static fluids meeting at an interface, where the interface is treated as a surface :
Omorashi, sometimes abbreviated as simply omo, is a form of fetish subculture originating and predominately recognized in Japan, in which participants experience arousal from having a full bladder or wetting themselves, wearing a diaper, or from seeing someone else experiencing a full bladder or wetting themselves.
In fluid dynamics, lubrication theory describes the flow of fluids in a geometry in which one dimension is significantly smaller than the others. An example is the flow above air hockey tables, where the thickness of the air layer beneath the puck is much smaller than the dimensions of the puck itself.
The percolation threshold is a mathematical concept in percolation theory that describes the formation of long-range connectivity in random systems. Below the threshold a giant connected component does not exist; while above it, there exists a giant component of the order of system size. In engineering and coffee making, percolation represents the flow of fluids through porous media, but in the mathematics and physics worlds it generally refers to simplified lattice models of random systems or networks (graphs), and the nature of the connectivity in them. The percolation threshold is the critical value of the occupation probability p, or more generally a critical surface for a group of parameters p1, p2, ..., such that infinite connectivity (percolation) first occurs.
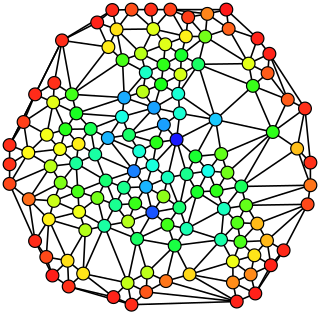
In graph theory, betweenness centrality is a measure of centrality in a graph based on shortest paths. For every pair of vertices in a connected graph, there exists at least one shortest path between the vertices such that either the number of edges that the path passes through or the sum of the weights of the edges is minimized. The betweenness centrality for each vertex is the number of these shortest paths that pass through the vertex.
Peter Grassberger is a professor well known for his work in statistical and particle physics. He is most famous for his contributions to chaos theory, where he introduced the idea of correlation dimension, a means of measuring a type of fractal dimension of the strange attractor.
Water retention on random surfaces is the simulation of catching of water in ponds on a surface of cells of various heights on a regular array such as a square lattice, where water is rained down on every cell in the system. The boundaries of the system are open and allow water to flow out. Water will be trapped in ponds, and eventually all ponds will fill to their maximum height, with any additional water flowing over spillways and out the boundaries of the system. The problem is to find the amount of water trapped or retained for a given surface. This has been studied extensively for random surfaces.
Percolation is a theoretical model used to understand the way activation and diffusion of neural activity occurs within neural networks. Percolation is a model used to explain how neural activity is transmitted across the various connections within the brain. Percolation theory can be easily understood by explaining its use in epidemiology. Individuals whom are infected with a disease can spread the disease through contact with others in their social network. Those who are more social and come into contact with more people will help to propagate the disease quicker than those who are less social. Factors such as occupation and sociability influence the rate of infection. Now, if one were to think of neurons as individuals and synaptic connections as the social bonds between people, then one can determine how easily messages between neurons will spread. When a neuron fires, the message is transmitted along all synaptic connections to other neurons until it can no longer continue. Synaptic connections are considered either open or closed and messages will flow along any and all open connections until they can go no further. Just like occupation and sociability play a key role in the spread of disease, so too do the number of neurons, synaptic plasticity and long-term potentiation when talking about neural percolation.
Phase Transitions and Critical Phenomena is a 20-volume series of books, comprising review articles on phase transitions and critical phenomena, published during 1972-2001. It is "considered the most authoritative series on the topic".
First passage percolation is a mathematical method used to describe the paths reachable in a random medium within a given amount of time.
Percolation surface critical behavior concerns the influence of surfaces on the critical behavior of percolation.
References
- ↑ David Wilkinson and Jorge F Willemsen, "Invasion percolation: a new form of percolation theory", J. Phys. A: Math. Gen. 16 (1983) 3365–3376.
 | This applied mathematics-related article is a stub. You can help Wikipedia by expanding it. |
 | This article about lattice models is a stub. You can help Wikipedia by expanding it. |
 | This fluid dynamics–related article is a stub. You can help Wikipedia by expanding it. |