
In fluid mechanics, hydrostatic equilibrium is the condition of a fluid or plastic solid at rest, which occurs when external forces, such as gravity, are balanced by a pressure-gradient force. In the planetary physics of Earth, the pressure-gradient force prevents gravity from collapsing the planetary atmosphere into a thin, dense shell, whereas gravity prevents the pressure-gradient force from diffusing the atmosphere into outer space. In general, it is what causes objects in space to be spherical.

In physics, Gauss's law, also known as Gauss's flux theorem, is one of Maxwell's equations. It is an application of the divergence theorem, and it relates the distribution of electric charge to the resulting electric field.

Poisson's equation is an elliptic partial differential equation of broad utility in theoretical physics. For example, the solution to Poisson's equation is the potential field caused by a given electric charge or mass density distribution; with the potential field known, one can then calculate the corresponding electrostatic or gravitational (force) field. It is a generalization of Laplace's equation, which is also frequently seen in physics. The equation is named after French mathematician and physicist Siméon Denis Poisson who published it in 1823.

In electromagnetism, the Mie solution to Maxwell's equations describes the scattering of an electromagnetic plane wave by a homogeneous sphere. The solution takes the form of an infinite series of spherical multipole partial waves. It is named after German physicist Gustav Mie.
Electron density or electronic density is the measure of the probability of an electron being present at an infinitesimal element of space surrounding any given point. It is a scalar quantity depending upon three spatial variables and is typically denoted as either or . The density is determined, through definition, by the normalised -electron wavefunction which itself depends upon variables. Conversely, the density determines the wave function modulo up to a phase factor, providing the formal foundation of density functional theory.

Stellar dynamics is the branch of astrophysics which describes in a statistical way the collective motions of stars subject to their mutual gravity. The essential difference from celestial mechanics is that the number of body
In astronomy, air mass or airmass is a measure of the amount of air along the line of sight when observing a star or other celestial source from below Earth's atmosphere. It is formulated as the integral of air density along the light ray.
A galactic halo is an extended, roughly spherical component of a galaxy which extends beyond the main, visible component. Several distinct components of a galaxy comprise its halo:

In modern models of physical cosmology, a dark matter halo is a basic unit of cosmological structure. It is a hypothetical region that has decoupled from cosmic expansion and contains gravitationally bound matter. A single dark matter halo may contain multiple virialized clumps of dark matter bound together by gravity, known as subhalos. Modern cosmological models, such as ΛCDM, propose that dark matter halos and subhalos may contain galaxies. The dark matter halo of a galaxy envelops the galactic disc and extends well beyond the edge of the visible galaxy. Thought to consist of dark matter, halos have not been observed directly. Their existence is inferred through observations of their effects on the motions of stars and gas in galaxies and gravitational lensing. Dark matter halos play a key role in current models of galaxy formation and evolution. Theories that attempt to explain the nature of dark matter halos with varying degrees of success include cold dark matter (CDM), warm dark matter, and massive compact halo objects (MACHOs).

The Jeans instability is a concept in astrophysics that describes an instability that leads to the gravitational collapse of a cloud of gas or dust. It causes the collapse of interstellar gas clouds and subsequent star formation. It occurs when the internal gas pressure is not strong enough to prevent the gravitational collapse of a region filled with matter. It is named after James Jeans.
The Navarro–Frenk–White (NFW) profile is a spatial mass distribution of dark matter fitted to dark matter halos identified in N-body simulations by Julio Navarro, Carlos Frenk and Simon White. The NFW profile is one of the most commonly used model profiles for dark matter halos.
Cosmic time, or cosmological time, is the time coordinate commonly used in the Big Bang models of physical cosmology. This concept of time avoids some issues related to relativity by being defined within a solution to the equations of general relativity widely used in cosmology.
In physics, Gauss's law for gravity, also known as Gauss's flux theorem for gravity, is a law of physics that is equivalent to Newton's law of universal gravitation. It is named after Carl Friedrich Gauss. It states that the flux of the gravitational field over any closed surface is proportional to the mass enclosed. Gauss's law for gravity is often more convenient to work from than Newton's law.

The Sérsic profile is a mathematical function that describes how the intensity of a galaxy varies with distance from its center. It is a generalization of de Vaucouleurs' law. José Luis Sérsic first published his law in 1963.
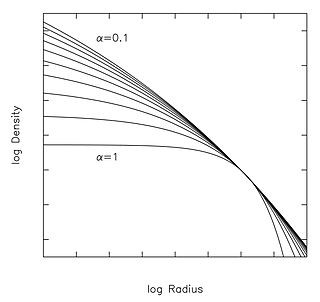
The Einasto profile is a mathematical function that describes how the density of a spherical stellar system varies with distance from its center. Jaan Einasto introduced his model at a 1963 conference in Alma-Ata, Kazakhstan.

Osipkov–Merritt models are mathematical representations of spherical stellar systems. The Osipkov–Merritt formula generates a one-parameter family of phase-space distribution functions that reproduce a specified density profile in a specified gravitational potential. The density and potential need not be self-consistently related. A free parameter adjusts the degree of velocity anisotropy, from isotropic to completely radial motions. The method is a generalization of Eddington's formula for constructing isotropic spherical models.
The Press–Schechter formalism is a mathematical model for predicting the number of objects of a certain mass within a given volume of the Universe. It was described in an academic paper by William H. Press and Paul Schechter in 1974.

The Jeans equations are a set of partial differential equations that describe the motion of a collection of stars in a gravitational field. The Jeans equations relate the second-order velocity moments to the density and potential of a stellar system for systems without collision. They are analogous to the Euler equations for fluid flow and may be derived from the collisionless Boltzmann equation. The Jeans equations can come in a variety of different forms, depending on the structure of what is being modelled. Most utilization of these equations has been found in simulations with large number of gravitationally bound objects.

In astrophysics, the Emden–Chandrasekhar equation is a dimensionless form of the Poisson equation for the density distribution of a spherically symmetric isothermal gas sphere subjected to its own gravitational force, named after Robert Emden and Subrahmanyan Chandrasekhar. The equation was first introduced by Robert Emden in 1907. The equation reads
The spherical collapse model describes the evolution of nearly homogeneous matter in the early Universe into collapsed virialized structures - dark matter halos. This model assumes that halos are spherical and dominated by gravity which leads to an analytical solution for several of the halos' properties such as density and radius over time.


















