In mathematics, the Bernoulli numbersBn are a sequence of rational numbers which occur frequently in analysis. The Bernoulli numbers appear in the Taylor series expansions of the tangent and hyperbolic tangent functions, in Faulhaber's formula for the sum of m-th powers of the first n positive integers, in the Euler–Maclaurin formula, and in expressions for certain values of the Riemann zeta function.

A fluid flowing around an object exerts a force on it. Lift is the component of this force that is perpendicular to the oncoming flow direction. It contrasts with the drag force, which is the component of the force parallel to the flow direction. Lift conventionally acts in an upward direction in order to counter the force of gravity, but it can act in any direction at right angles to the flow.

Daniel Bernoulli FRS was a Swiss mathematician and physicist and was one of the many prominent mathematicians in the Bernoulli family from Basel. He is particularly remembered for his applications of mathematics to mechanics, especially fluid mechanics, and for his pioneering work in probability and statistics. His name is commemorated in the Bernoulli's principle, a particular example of the conservation of energy, which describes the mathematics of the mechanism underlying the operation of two important technologies of the 20th century: the carburetor and the airplane wing.
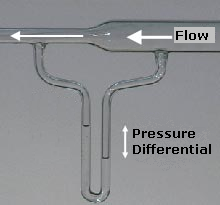
In fluid dynamics, Bernoulli's principle states that an increase in the speed of a fluid occurs simultaneously with a decrease in static pressure or a decrease in the fluid's potential energy. The principle is named after Daniel Bernoulli who published it in his book Hydrodynamica in 1738. Although Bernoulli deduced that pressure decreases when the flow speed increases, it was Leonhard Euler in 1752 who derived Bernoulli's equation in its usual form. The principle is only applicable for isentropic flows: when the effects of irreversible processes and non-adiabatic processes are small and can be neglected.

Jacob Bernoulli was one of the many prominent mathematicians in the Bernoulli family. He was an early proponent of Leibnizian calculus and sided with Gottfried Wilhelm Leibniz during the Leibniz–Newton calculus controversy. He is known for his numerous contributions to calculus, and along with his brother Johann, was one of the founders of the calculus of variations. He also discovered the fundamental mathematical constant e. However, his most important contribution was in the field of probability, where he derived the first version of the law of large numbers in his work Ars Conjectandi.
In probability and statistics, a Bernoulli process is a finite or infinite sequence of binary random variables, so it is a discrete-time stochastic process that takes only two values, canonically 0 and 1. The component Bernoulli variablesXi are identically distributed and independent. Prosaically, a Bernoulli process is a repeated coin flipping, possibly with an unfair coin. Every variable Xi in the sequence is associated with a Bernoulli trial or experiment. They all have the same Bernoulli distribution. Much of what can be said about the Bernoulli process can also be generalized to more than two outcomes ; this generalization is known as the Bernoulli scheme.

In the theory of probability and statistics, a Bernoulli trial is a random experiment with exactly two possible outcomes, "success" and "failure", in which the probability of success is the same every time the experiment is conducted. It is named after Jacob Bernoulli, a 17th-century Swiss mathematician, who analyzed them in his Ars Conjectandi (1713).

In probability theory and statistics, the Bernoulli distribution, named after Swiss mathematician Jacob Bernoulli, is the discrete probability distribution of a random variable which takes the value 1 with probability and the value 0 with probability . Less formally, it can be thought of as a model for the set of possible outcomes of any single experiment that asks a yes–no question. Such questions lead to outcomes that are boolean-valued: a single bit whose value is success/yes/true/one with probability p and failure/no/false/zero with probability q. It can be used to represent a coin toss where 1 and 0 would represent "heads" and "tails", respectively, and p would be the probability of the coin landing on heads or tails, respectively. In particular, unfair coins would have
The Bernoulli family of Basel was a patrician family, notable for having produced eight mathematically gifted academics who, among them, contributed substantially to the development of mathematics and physics during the early modern period.

Johann Bernoulli was a Swiss mathematician and was one of the many prominent mathematicians in the Bernoulli family. He is known for his contributions to infinitesimal calculus and educating Leonhard Euler in the pupil's youth.

Bernoulli is a lunar impact crater that is located in the northeast part of the Moon. It lies to the south of the crater Messala, and east of Geminus.

Nicolaus II Bernoulli, a.k.a. Niklaus Bernoulli, Nikolaus Bernoulli was a Swiss mathematician as were his father Johann Bernoulli and one of his brothers, Daniel Bernoulli. He was one of the many prominent mathematicians in the Bernoulli family.

Guillaume François Antoine, Marquis de l'Hôpital, also known as Guillaume-François-Antoine Marquis de l'Hôpital, Marquis de Sainte-Mesme, Comte d'Entremont, and Seigneur d'Ouques-la-Chaise, was a French mathematician. His name is firmly associated with l'Hôpital's rule for calculating limits involving indeterminate forms 0/0 and ∞/∞. Although the rule did not originate with l'Hôpital, it appeared in print for the first time in his 1696 treatise on the infinitesimal calculus, entitled Analyse des Infiniment Petits pour l'Intelligence des Lignes Courbes. This book was a first systematic exposition of differential calculus. Several editions and translations to other languages were published and it became a model for subsequent treatments of calculus.

In algebraic geometry, a lemniscate is any of several figure-eight or ∞-shaped curves. The word comes from the Latin "lēmniscātus" meaning "decorated with ribbons", from the Greek λημνίσκος meaning "ribbons", or which alternatively may refer to the wool from which the ribbons were made.
The Institute of Mathematical Statistics is an international professional and scholarly society devoted to the development, dissemination, and application of statistics and probability. The Institute currently has about 4,000 members in all parts of the world. Beginning in 2005, the institute started offering joint membership with the Bernoulli Society for Mathematical Statistics and Probability as well as with the International Statistical Institute. The Institute was founded in 1935 with Harry C. Carver and Henry L. Rietz as its two most important supporters. The institute publishes a variety of journals, and holds several international conference every year.
Bernoulli can refer to:
The Bernoulli Society is a professional association which aims to further the progress of probability and mathematical statistics, founded as part of the International Statistical Institute in 1975. It is named after the Bernoulli family of mathematicians and scientists whose researchers covered "most areas of scientific knowledge".

Ars Conjectandi is a book on combinatorics and mathematical probability written by Jacob Bernoulli and published in 1713, eight years after his death, by his nephew, Niklaus Bernoulli. The seminal work consolidated, apart from many combinatorial topics, many central ideas in probability theory, such as the very first version of the law of large numbers: indeed, it is widely regarded as the founding work of that subject. It also addressed problems that today are classified in the twelvefold way and added to the subjects; consequently, it has been dubbed an important historical landmark in not only probability but all combinatorics by a plethora of mathematical historians. The importance of this early work had a large impact on both contemporary and later mathematicians; for example, Abraham de Moivre.

French submarine Bernoulli (Q83) was a Laubeuf type submarine of the Brumaire class, built for the French Navy prior to World War I.

In probability theory, statistics, and machine learning, the continuous Bernoulli distribution is a family of continuous probability distributions parameterized by a single shape parameter , defined on the unit interval , by: