In the mathematical field of geometric topology, the Poincaré conjecture is a theorem about the characterization of the 3-sphere, which is the hypersphere that bounds the unit ball in four-dimensional space.

In algebraic and differential geometry, a Calabi–Yau manifold, also known as a Calabi–Yau space, is a particular type of manifold which has properties, such as Ricci flatness, yielding applications in theoretical physics. Particularly in superstring theory, the extra dimensions of spacetime are sometimes conjectured to take the form of a 6-dimensional Calabi–Yau manifold, which led to the idea of mirror symmetry. Their name was coined by Candelas et al. (1985), after Eugenio Calabi who first conjectured that such surfaces might exist, and Shing-Tung Yau who proved the Calabi conjecture.
Riemannian geometry is the branch of differential geometry that studies Riemannian manifolds, defined as smooth manifolds with a Riemannian metric. This gives, in particular, local notions of angle, length of curves, surface area and volume. From those, some other global quantities can be derived by integrating local contributions.
In mathematics, Thurston's geometrization conjecture states that each of certain three-dimensional topological spaces has a unique geometric structure that can be associated with it. It is an analogue of the uniformization theorem for two-dimensional surfaces, which states that every simply connected Riemann surface can be given one of three geometries.
In the mathematical field of Riemannian geometry, the scalar curvature is a measure of the curvature of a Riemannian manifold. To each point on a Riemannian manifold, it assigns a single real number determined by the geometry of the metric near that point. It is defined by a complicated explicit formula in terms of partial derivatives of the metric components, although it is also characterized by the volume of infinitesimally small geodesic balls. In the context of the differential geometry of surfaces, the scalar curvature is twice the Gaussian curvature, and completely characterizes the curvature of a surface. In higher dimensions, however, the scalar curvature only represents one particular part of the Riemann curvature tensor.
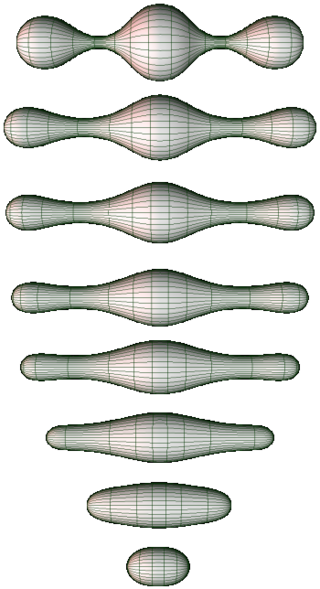
In the mathematical fields of differential geometry and geometric analysis, the Ricci flow, sometimes also referred to as Hamilton's Ricci flow, is a certain partial differential equation for a Riemannian metric. It is often said to be analogous to the diffusion of heat and the heat equation, due to formal similarities in the mathematical structure of the equation. However, it is nonlinear and exhibits many phenomena not present in the study of the heat equation.
In the mathematical field of differential geometry, there are various splitting theorems on when a pseudo-Riemannian manifold can be given as a metric product. The best-known is the Cheeger–Gromoll splitting theorem for Riemannian manifolds, although there has also been research into splitting of Lorentzian manifolds.
In mathematics, the soul theorem is a theorem of Riemannian geometry that largely reduces the study of complete manifolds of non-negative sectional curvature to that of the compact case. Jeff Cheeger and Detlef Gromoll proved the theorem in 1972 by generalizing a 1969 result of Gromoll and Wolfgang Meyer. The related soul conjecture, formulated by Cheeger and Gromoll at that time, was proved twenty years later by Grigori Perelman.

In mathematics, a 3-manifold is a topological space that locally looks like a three-dimensional Euclidean space. A 3-manifold can be thought of as a possible shape of the universe. Just as a sphere looks like a plane to a small and close enough observer, all 3-manifolds look like our universe does to a small enough observer. This is made more precise in the definition below.
In mathematics, a 4-manifold is a 4-dimensional topological manifold. A smooth 4-manifold is a 4-manifold with a smooth structure. In dimension four, in marked contrast with lower dimensions, topological and smooth manifolds are quite different. There exist some topological 4-manifolds which admit no smooth structure, and even if there exists a smooth structure, it need not be unique.

Richard Melvin Schoen is an American mathematician known for his work in differential geometry and geometric analysis. He is best known for the resolution of the Yamabe problem in 1984.
In the mathematical field of differential geometry, a smooth map between Riemannian manifolds is called harmonic if its coordinate representatives satisfy a certain nonlinear partial differential equation. This partial differential equation for a mapping also arises as the Euler-Lagrange equation of a functional called the Dirichlet energy. As such, the theory of harmonic maps contains both the theory of unit-speed geodesics in Riemannian geometry and the theory of harmonic functions.

Tian Gang is a Chinese mathematician. He is a professor of mathematics at Peking University and Higgins Professor Emeritus at Princeton University. He is known for contributions to the mathematical fields of Kähler geometry, Gromov-Witten theory, and geometric analysis.
Huai-Dong Cao is a Chinese–American mathematician. He is the A. Everett Pitcher Professor of Mathematics at Lehigh University. He is known for his research contributions to the Ricci flow, a topic in the field of geometric analysis.
In the mathematical area of topology, the generalized Poincaré conjecture is a statement that a manifold which is a homotopy sphere is a sphere. More precisely, one fixes a category of manifolds: topological (Top), piecewise linear (PL), or differentiable (Diff). Then the statement is

Thierry Aubin was a French mathematician who worked at the Centre de Mathématiques de Jussieu, and was a leading expert on Riemannian geometry and non-linear partial differential equations. His fundamental contributions to the theory of the Yamabe equation led, in conjunction with results of Trudinger and Schoen, to a proof of the Yamabe Conjecture: every compact Riemannian manifold can be conformally rescaled to produce a manifold of constant scalar curvature. Along with Yau, he also showed that Kähler manifolds with negative first Chern classes always admit Kähler–Einstein metrics, a result closely related to the Calabi conjecture. The latter result, established by Yau, provides the largest class of known examples of compact Einstein manifolds. Aubin was the first mathematician to propose the Cartan–Hadamard conjecture.
The Yamabe problem refers to a conjecture in the mathematical field of differential geometry, which was resolved in the 1980s. It is a statement about the scalar curvature of Riemannian manifolds:
Let (M,g) be a closed smooth Riemannian manifold. Then there exists a positive and smooth function f on M such that the Riemannian metric fg has constant scalar curvature.
In mathematics, the Almgren–Pitts min-max theory is an analogue of Morse theory for hypersurfaces.
Wanxiong Shi was a Chinese mathematician. He was known for his fundamental work in the theory of Ricci flow.

Aaron Naber is an American mathematician.











