
In mathematics, a Diophantine equation is an equation, typically a polynomial equation in two or more unknowns with integer coefficients, for which only integer solutions are of interest. A linear Diophantine equation equates to a constant the sum of two or more monomials, each of degree one. An exponential Diophantine equation is one in which unknowns can appear in exponents.
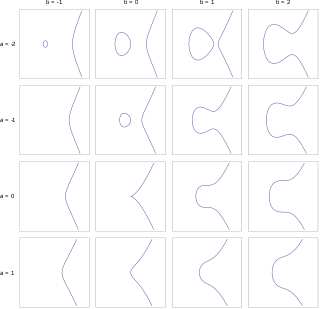
In mathematics, an elliptic curve is a smooth, projective, algebraic curve of genus one, on which there is a specified point O. An elliptic curve is defined over a field K and describes points in K2, the Cartesian product of K with itself. If the field's characteristic is different from 2 and 3, then the curve can be described as a plane algebraic curve which consists of solutions (x, y) for:
Catalan's conjecture (or Mihăilescu's theorem) is a theorem in number theory that was conjectured by the mathematician Eugène Charles Catalan in 1844 and proven in 2002 by Preda Mihăilescu at Paderborn University. The integers 23 and 32 are two perfect powers (that is, powers of exponent higher than one) of natural numbers whose values (8 and 9, respectively) are consecutive. The theorem states that this is the only case of two consecutive perfect powers. That is to say, that

Faltings's theorem is a result in arithmetic geometry, according to which a curve of genus greater than 1 over the field of rational numbers has only finitely many rational points. This was conjectured in 1922 by Louis Mordell, and known as the Mordell conjecture until its 1983 proof by Gerd Faltings. The conjecture was later generalized by replacing by any number field.
In mathematics, a proof by infinite descent, also known as Fermat's method of descent, is a particular kind of proof by contradiction used to show that a statement cannot possibly hold for any number, by showing that if the statement were to hold for a number, then the same would be true for a smaller number, leading to an infinite descent and ultimately a contradiction. It is a method which relies on the well-ordering principle, and is often used to show that a given equation, such as a Diophantine equation, has no solutions.
In mathematics, the Birch and Swinnerton-Dyer conjecture describes the set of rational solutions to equations defining an elliptic curve. It is an open problem in the field of number theory and is widely recognized as one of the most challenging mathematical problems. It is named after mathematicians Bryan John Birch and Peter Swinnerton-Dyer, who developed the conjecture during the first half of the 1960s with the help of machine computation. Only special cases of the conjecture have been proven.
In mathematics, the Nagell–Lutz theorem is a result in the diophantine geometry of elliptic curves, which describes rational torsion points on elliptic curves over the integers. It is named for Trygve Nagell and Élisabeth Lutz.
Ribet's theorem is part of number theory. It concerns properties of Galois representations associated with modular forms. It was proposed by Jean-Pierre Serre and proven by Ken Ribet. The proof was a significant step towards the proof of Fermat's Last Theorem (FLT). As shown by Serre and Ribet, the Taniyama–Shimura conjecture and the epsilon conjecture together imply that FLT is true.

In mathematics, the Fermat curve is the algebraic curve in the complex projective plane defined in homogeneous coordinates (X:Y:Z) by the Fermat equation:

In number theory, a congruent number is a positive integer that is the area of a right triangle with three rational number sides. A more general definition includes all positive rational numbers with this property.
In number theory and algebraic geometry, a rational point of an algebraic variety is a point whose coordinates belong to a given field. If the field is not mentioned, the field of rational numbers is generally understood. If the field is the field of real numbers, a rational point is more commonly called a real point.

In number theory, a congruum is the difference between successive square numbers in an arithmetic progression of three squares. That is, if , , and are three square numbers that are equally spaced apart from each other, then the spacing between them, , is called a congruum.
174 is the natural number following 173 and preceding 175.
In number theory, the classical modular curve is an irreducible plane algebraic curve given by an equation
In mathematics, Hall's conjecture is an open question on the differences between perfect squares and perfect cubes. It asserts that a perfect square y2 and a perfect cube x3 that are not equal must lie a substantial distance apart. This question arose from consideration of the Mordell equation in the theory of integer points on elliptic curves.

In number theory, Fermat's Last Theorem states that no three positive integers a, b, and c satisfy the equation an + bn = cn for any integer value of n greater than 2. The cases n = 1 and n = 2 have been known since antiquity to have infinitely many solutions.
Fermat's Last Theorem is a theorem in number theory, originally stated by Pierre de Fermat in 1637 and proven by Andrew Wiles in 1995. The statement of the theorem involves an integer exponent n larger than 2. In the centuries following the initial statement of the result and before its general proof, various proofs were devised for particular values of the exponent n. Several of these proofs are described below, including Fermat's proof in the case n = 4, which is an early example of the method of infinite descent.

Fermat's right triangle theorem is a non-existence proof in number theory, published in 1670 among the works of Pierre de Fermat, soon after his death. It is the only complete proof given by Fermat. It has many equivalent formulations, one of which was stated in 1225 by Fibonacci. In its geometric forms, it states:

In mathematics, a stella octangula number is a figurate number based on the stella octangula, of the form n(2n2 − 1).

In arithmetic and algebra the sixth power of a number n is the result of multiplying six instances of n together. So:










