Related Research Articles
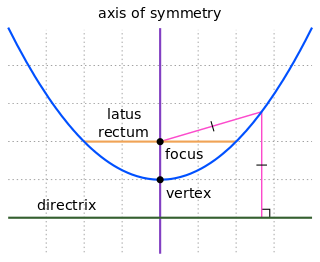
In mathematics, a parabola is a plane curve which is mirror-symmetrical and is approximately U-shaped. It fits several other superficially different mathematical descriptions, which can all be proved to define exactly the same curves.

A bullet is a kinetic projectile and the component of firearm ammunition that is expelled from the gun barrel during shooting. The term is from Middle French and originated as the diminutive of the word boulle (boullet), which means "small ball". Bullets are made of a variety of materials such as copper, lead, steel, polymer, rubber and even wax. They are available either singly as in muzzleloading and cap and ball firearms or as a component of paper cartridges, but much more commonly in the form of metallic cartridges. Bullets are made in varied shapes and constructions depending on the intended applications, including specialized functions such as hunting, target shooting, training and combat.

In elementary geometry, the property of being perpendicular (perpendicularity) is the relationship between two lines which meet at a right angle. The property extends to other related geometric objects.

An armor-piercing shell, AP for short, is a type of ammunition designed to penetrate armor. From the 1860s to 1950s, a major application of armor-piercing projectiles was to defeat the thick armor carried on many warships. From the 1920s onwards, armor-piercing weapons were required for anti-tank missions. AP rounds smaller than 20 mm are typically known as "armor-piercing ammunition", and are intended for lightly-armored targets such as body armor, bulletproof glass and light armored vehicles. The AP shell is now seldom used in naval warfare, as modern warships have little or no armor protection. In the anti-tank role, as tank armor improved during World War II newer designs began to use a smaller but dense penetrating body within a larger shell. These lightweight shells were fired at very high muzzle velocity and retained that speed and the associated penetrating power over longer distances.
A projectile is any object thrown into space by the exertion of a force. Although any object in motion through space may be called a projectile, the term more commonly refers to a ranged weapon. Mathematical equations of motion are used to analyze projectile trajectory. An object projected at an angle to the horizontal has both the vertical and horizontal components of velocity. The vertical component of the velocity on the y-axis given as Vy=USin(teta) while the horizontal component of the velocity Vx=UCos(teta). There are various terms used in projectiles at specific angle teta 1. Time to reach maximum height. It is symbolized as (t), which is the time taken for the projectile to reach the maximum height from the plane of projection. Mathematically, it is given as t=USin(teta)/g Where g=acceleration due to gravity(app 10m/s²) U= initial velocity (m/s) teta= angle made by the projectile with the horizontal axis.

A trajectory or flight path is the path that an object with mass in motion follows through space as a function of time. In classical mechanics, a trajectory is defined by Hamiltonian mechanics via canonical coordinates; hence, a complete trajectory is defined by position and momentum, simultaneously. Trajectory in quantum mechanics is not defined due to Heisenberg uncertainty principle that position and momentum can not be measured simultaneously.

Evangelista Torricelli was an Italian physicist and mathematician, and a student of Galileo. He is best known for his invention of the barometer, but is also known for his advances in optics and work on the method of indivisibles.
The Method of Mechanical Theorems, also referred to as The Method, is considered one of the major surviving works of the ancient Greek polymath Archimedes. The Method takes the form of a letter from Archimedes to Eratosthenes, the chief librarian at the Library of Alexandria, and contains the first attested explicit use of indivisibles. The work was originally thought to be lost, but in 1906 was rediscovered in the celebrated Archimedes Palimpsest. The palimpsest includes Archimedes' account of the "mechanical method", so-called because it relies on the law of the lever, which was first demonstrated by Archimedes, and of the center of mass, which he had found for many special shapes.
Muzzle velocity is the speed of a projectile at the moment it leaves the end of a gun's barrel. Firearm muzzle velocities range from approximately 120 m/s (390 ft/s) to 370 m/s (1,200 ft/s) in black powder muskets, to more than 1,200 m/s (3,900 ft/s) in modern rifles with high-velocity cartridges such as the .220 Swift and .204 Ruger, all the way to 1,700 m/s (5,600 ft/s) for tank guns firing kinetic energy penetrator ammunition. To simulate orbital debris impacts on spacecraft, NASA launches projectiles through light-gas guns at speeds up to 8,500 m/s (28,000 ft/s).

External ballistics or exterior ballistics is the part of ballistics that deals with the behavior of a projectile in flight. The projectile may be powered or un-powered, guided or unguided, spin or fin stabilized, flying through an atmosphere or in the vacuum of space, but most certainly flying under the influence of a gravitational field.

Sloped armour is armour that is neither in a vertical nor a horizontal position. Such "angled" armour is often mounted on tanks and other armoured fighting vehicles (AFVs), as well as naval vessels such as battleships and cruisers. Sloping an armour plate makes it harder to penetrate for antitank-weapons, such as armour-piercing shells and rockets, if they take a more or less horizontal path to their target, as is often the case. The better protection is caused by three main effects.

In the differential geometry of curves, the evolute of a curve is the locus of all its centers of curvature. That is to say that when the center of curvature of each point on a curve is drawn, the resultant shape will be the evolute of that curve. The evolute of a circle is therefore a single point at its center. Equivalently, an evolute is the envelope of the normals to a curve.
In the differential geometry of curves, a roulette is a kind of curve, generalizing cycloids, epicycloids, hypocycloids, trochoids, and involutes.

Projectile motion is a form of motion experienced by an object or particle your that is projected near the Earth's surface and moves along a curved path under the action of gravity only. This curved path was shown by Galileo to be a parabola. The study of such motions is called ballistics, and such a trajectory is a ballistic trajectory. The only force of significance that acts on the object is gravity, which acts downward, thus imparting to the object a downward acceleration. Because of the object's inertia, no external horizontal force is needed to maintain the horizontal velocity component of the object. Taking other forces into account, such as friction from aerodynamic drag or internal propulsion such as in a rocket, requires additional analysis. A ballistic missile is a missile only guided during the relatively brief initial powered phase of flight, and whose subsequent course is governed by the laws of classical mechanics.
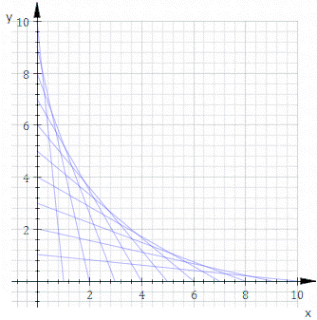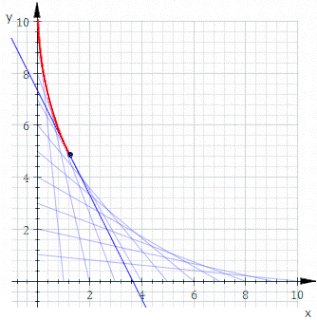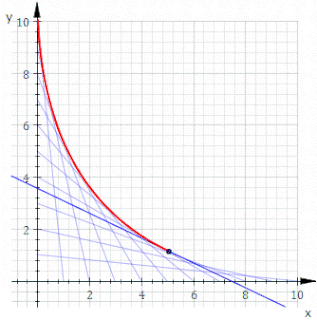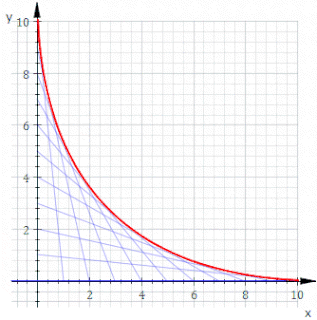
In geometry, an envelope of a family of curves in the plane is a curve that is tangent to each member of the family at some point, and these points of tangency together form the whole envelope. Classically, a point on the envelope can be thought of as the intersection of two "infinitesimally adjacent" curves, meaning the limit of intersections of nearby curves. This idea can be generalized to an envelope of surfaces in space, and so on to higher dimensions.
Point-blank range is any distance over which the trajectory of a given projectile fired from a given weapon remains sufficiently flat that one can strike a target by firing at it directly. Point-blank range will vary by a weapon's external ballistics characteristics and target chosen. A weapon with a flatter trajectory will permit a longer maximum point-blank range for a given target size, while a larger target will allow a longer point-blank range for a given weapon.
A proof test is a form of stress test to demonstrate the fitness of a load-bearing structure. An individual proof test may apply only to the unit tested, or to its design in general for mass-produced items. Such a structure is often subjected to loads above that expected in actual use, demonstrating safety and design margin. Proof testing is nominally a nondestructive test, particularly if both design margins and test levels are well-chosen. However, unit failures are by definition considered to have been destroyed for their originally-intended use and load levels.

In mathematics, a conic section is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties.

Track geometry is three-dimensional geometry of track layouts and associated measurements used in design, construction and maintenance of railroad tracks. The subject is used in the context of standards, speed limits and other regulations in the areas of track gauge, alignment, elevation, curvature and track surface. Although, the geometry of the tracks is three-dimensional by nature, the standards are usually expressed in two separate layouts for horizontal and vertical.
In function graphing, a horizontal translation is a transformation which results in a graph that is equivalent to shifting the base graph left or right in the direction of the x-axis. A graph is translated k units horizontally by moving each point on the graph k units horizontally.
References
Philip Robinson, On the Geometrical Approach to Projectile Motion, The Mathematical Gazette, Vol. 82, No. 493, 1998, pp. 118–122
| This physics-related article is a stub. You can help Wikipedia by expanding it. |