| Discipline | Mathematics |
|---|---|
| Language | English |
| Publication details | |
Publication history | 1976 to present |
| Publisher | |
| Frequency | Biannual |
| Standard abbreviations | |
| Real Anal. Exch. | |
| Real Anal. Exchange | |
| Indexing | |
| ISSN | 0147-1937 |
| LCCN | 77-647120 |
| Links | |
The Real Analysis Exchange (RAEX) is a biannual mathematics journal, publishing survey articles, research papers, and conference reports in real analysis and related topics. Its editor-in-chief is Paul D. Humke.
  | This article about a mathematics journal is a stub. You can help Wikipedia by expanding it. See tips for writing articles about academic journals. Further suggestions might be found on the article's talk page. |
Complex analysis, traditionally known as the theory of functions of a complex variable, is the branch of mathematical analysis that investigates functions of complex numbers. It is useful in many branches of mathematics, including algebraic geometry, number theory, analytic combinatorics, applied mathematics; as well as in physics, including the branches of hydrodynamics, thermodynamics, and particularly quantum mechanics. By extension, use of complex analysis also has applications in engineering fields such as nuclear, aerospace, mechanical and electrical engineering.
A complex number is a number that can be expressed in the form a + bi, where a and b are real numbers, and i is a solution of the equation x2 = −1. Because no real number satisfies this equation, i is called an imaginary number. For the complex number a + bi, a is called the real part, and b is called the imaginary part. Despite the historical nomenclature "imaginary", complex numbers are regarded in the mathematical sciences as just as "real" as the real numbers, and are fundamental in many aspects of the scientific description of the natural world.
Functional analysis is a branch of mathematical analysis, the core of which is formed by the study of vector spaces endowed with some kind of limit-related structure and the linear functions defined on these spaces and respecting these structures in a suitable sense. The historical roots of functional analysis lie in the study of spaces of functions and the formulation of properties of transformations of functions such as the Fourier transform as transformations defining continuous, unitary etc. operators between function spaces. This point of view turned out to be particularly useful for the study of differential and integral equations.
A geographic information system (GIS) is a system designed to capture, store, manipulate, analyze, manage, and present spatial or geographic data. GIS applications are tools that allow users to create interactive queries, analyze spatial information, edit data in maps, and present the results of all these operations. GIS sometimes refers to geographic information science (GIScience), the science underlying geographic concepts, applications, and systems.
In mathematics, a holomorphic function is a complex-valued function of one or more complex variables that is, at every point of its domain, complex differentiable in a neighbourhood of the point. The existence of a complex derivative in a neighbourhood is a very strong condition, for it implies that any holomorphic function is actually infinitely differentiable and equal, locally, to its own Taylor series (analytic). Holomorphic functions are the central objects of study in complex analysis.
The history of calculus is fraught with philosophical debates about the meaning and logical validity of fluxions or infinitesimal numbers. The standard way to resolve these debates is to define the operations of calculus using epsilon–delta procedures rather than infinitesimals. Non-standard analysis instead reformulates the calculus using a logically rigorous notion of infinitesimal numbers.
In mathematics, real analysis is the branch of mathematical analysis that studies the behavior of real numbers, sequences and series of real numbers, and real-valued functions. Some particular properties of real-valued sequences and functions that real analysis studies include convergence, limits, continuity, smoothness, differentiability and integrability.
Mathematical analysis is the branch of mathematics dealing with limits and related theories, such as differentiation, integration, measure, infinite series, and analytic functions.
In mathematics, a (real) interval is a set of real numbers with the property that any number that lies between two numbers in the set is also included in the set. For example, the set of all numbers x satisfying 0 ≤ x ≤ 1 is an interval which contains 0 and 1, as well as all numbers between them. Other examples of intervals are the set of all real numbers , the set of all negative real numbers, and the empty set.
In mathematics, infinitesimals are things so small that there is no way to measure them. The insight with exploiting infinitesimals was that entities could still retain certain specific properties, such as angle or slope, even though these entities were quantitatively small. The word infinitesimal comes from a 17th-century Modern Latin coinage infinitesimus, which originally referred to the "infinity-th" item in a sequence. Infinitesimals are a basic ingredient in the procedures of infinitesimal calculus as developed by Leibniz, including the law of continuity and the transcendental law of homogeneity. In common speech, an infinitesimal object is an object that is smaller than any feasible measurement, but not zero in size—or, so small that it cannot be distinguished from zero by any available means. Hence, when used as an adjective, "infinitesimal" means "extremely small". To give it a meaning, it usually must be compared to another infinitesimal object in the same context. Infinitely many infinitesimals are summed to produce an integral.
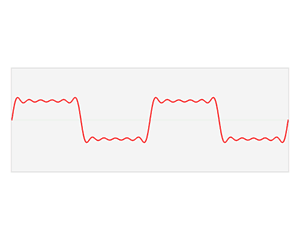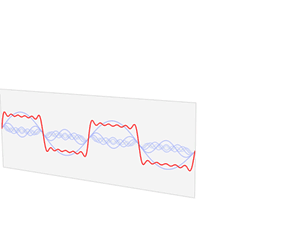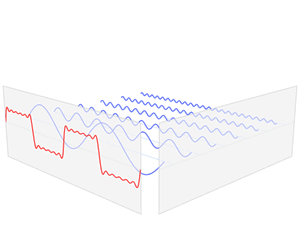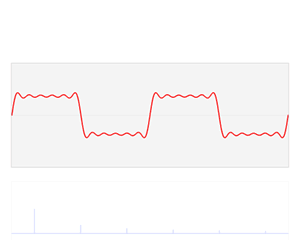
Time domain refers to the analysis of mathematical functions, physical signals or time series of economic or environmental data, with respect to time. In the time domain, the signal or function's value is known for all real numbers, for the case of continuous time, or at various separate instants in the case of discrete time. An oscilloscope is a tool commonly used to visualize real-world signals in the time domain. A time-domain graph shows how a signal changes with time, whereas a frequency-domain graph shows how much of the signal lies within each given frequency band over a range of frequencies.
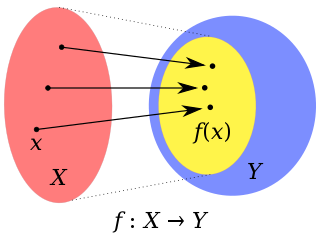
In mathematics, and more specifically in naïve set theory, the range of a function refers to either the codomain or the image of the function, depending upon usage. Modern usage almost always uses range to mean image.
Elias Menachem Stein was an American mathematician, and a leading figure in the field of harmonic analysis. He was professor of Mathematics at Princeton University from 1963 until his death in 2018.
A voltage source is a two-terminal device which can maintain a fixed voltage. An ideal voltage source can maintain the fixed voltage independent of the load resistance or the output current. However, a real-world voltage source cannot supply unlimited current. A voltage source is the dual of a current source. Real-world sources of electrical energy, such as batteries, generators, can be modeled for analysis purposes as a combination of an ideal voltage source and additional combinations of impedance elements.
In mathematics, 0.999... denotes the repeating decimal consisting of infinitely many 9s after the decimal point. This repeating decimal represents the smallest number no less than every decimal number in the sequence. This number is equal to 1. In other words, "0.999..." and "1" represent the same number. There are many ways of showing this equality, from intuitive arguments to mathematically rigorous proofs. The technique used depends on the target audience, background assumptions, historical context, and preferred development of the real numbers, the system within which 0.999... is commonly defined.
The National University of Science and Technology "MISiS" is a Russian technical university—the first to gain the status of National University of Science and Technology. As of 2018, NUST MISIS comprises nine colleges and five branches—four in Russia and one abroad.
Walter Rudin was an Austrian-American mathematician and professor of Mathematics at the University of Wisconsin–Madison.
Rautaruukki Oyj using the marketing name Ruukki is a Finnish company, headquartered in Helsinki, which manufactures and supplies metal-based components and systems to the construction and engineering industries.
In mathematics, a real number is a value of a continuous quantity that can represent a distance along a line. The adjective real in this context was introduced in the 17th century by René Descartes, who distinguished between real and imaginary roots of polynomials. The real numbers include all the rational numbers, such as the integer −5 and the fraction 4/3, and all the irrational numbers, such as √2. Included within the irrationals are the transcendental numbers, such as π (3.14159265...). In addition to measuring distance, real numbers can be used to measure quantities such as time, mass, energy, velocity, and many more.
Rating-Agentur Expert RA GmbH is a credit rating agency established in Frankfurt am Main, Germany. The agency is a business unit of International group RAEX operating in the European Union since 2013. It is the first rating agency with Russian roots, officially recognized by European Union Supervisory Authority.
