
Young's modulus is a mechanical property of solid materials that measures the tensile or compressive stiffness when the force is applied lengthwise. It is the modulus of elasticity for tension or axial compression. Young's modulus is defined as the ratio of the stress applied to the object and the resulting axial strain in the linear elastic region of the material.

Nacre, also known as mother of pearl, is an organic–inorganic composite material produced by some molluscs as an inner shell layer. It is also the material of which pearls are composed. It is strong, resilient, and iridescent.
In physics and mathematics, a random field is a random function over an arbitrary domain. That is, it is a function that takes on a random value at each point (or some other domain). It is also sometimes thought of as a synonym for a stochastic process with some restriction on its index set. That is, by modern definitions, a random field is a generalization of a stochastic process where the underlying parameter need no longer be real or integer valued "time" but can instead take values that are multidimensional vectors or points on some manifold.

Microstructure is the very small scale structure of a material, defined as the structure of a prepared surface of material as revealed by an optical microscope above 25× magnification. The microstructure of a material can strongly influence physical properties such as strength, toughness, ductility, hardness, corrosion resistance, high/low temperature behaviour or wear resistance. These properties in turn govern the application of these materials in industrial practice.
Micromechanics is the analysis of composite or heterogeneous materials on the level of the individual constituents that constitute these materials.

In materials science Functionally Graded Materials (FGMs) may be characterized by the variation in composition and structure gradually over volume, resulting in corresponding changes in the properties of the material. The materials can be designed for specific function and applications. Various approaches based on the bulk, preform processing, layer processing and melt processing are used to fabricate the functionally graded materials.
High-performance fiber-reinforced cementitious composites (HPFRCCs) are a group of fiber-reinforced cement-based composites that possess the unique ability to flex and self-strengthen before fracturing. This particular class of concrete was developed with the goal of solving the structural problems inherent with today’s typical concrete, such as its tendency to fail in a brittle manner under excessive loading and its lack of long-term durability. Because of their design and composition, HPFRCCs possess the remarkable ability to plastically yield and harden under excessive loading, so that they flex or deform before fracturing, a behavior similar to that exhibited by most metals under tensile or bending stresses. Because of this capability, HPFRCCs are more resistant to cracking and last considerably longer than normal concrete. Another extremely desirable property of HPFRCCs is their low density. A less dense, and hence lighter material means that HPFRCCs could eventually require much less energy to produce and handle, deeming them a more economical building material. Because of HPFRCCs’ lightweight composition and ability to strain harden, it has been proposed that they could eventually become a more durable and efficient alternative to typical concrete.
Methods have been devised to modify the yield strength, ductility, and toughness of both crystalline and amorphous materials. These strengthening mechanisms give engineers the ability to tailor the mechanical properties of materials to suit a variety of different applications. For example, the favorable properties of steel result from interstitial incorporation of carbon into the iron lattice. Brass, a binary alloy of copper and zinc, has superior mechanical properties compared to its constituent metals due to solution strengthening. Work hardening has also been used for centuries by blacksmiths to introduce dislocations into materials, increasing their yield strengths.
Material failure theory is an interdisciplinary field of materials science and solid mechanics which attempts to predict the conditions under which solid materials fail under the action of external loads. The failure of a material is usually classified into brittle failure (fracture) or ductile failure (yield). Depending on the conditions most materials can fail in a brittle or ductile manner or both. However, for most practical situations, a material may be classified as either brittle or ductile.
Fiber volume ratio is an important mathematical element in composite engineering. Fiber volume ratio, or fiber volume fraction, is the percentage of fiber volume in the entire volume of a fiber-reinforced composite material. When manufacturing polymer composites, fibers are impregnated with resin. The amount of resin to fiber ratio is calculated by the geometric organization of the fibers, which affects the amount of resin that can enter the composite. The impregnation around the fibers is highly dependent on the orientation of the fibers and the architecture of the fibers. The geometric analysis of the composite can be seen in the cross-section of the composite. Voids are often formed in a composite structure throughout the manufacturing process and must be calculated into the total fiber volume fraction of the composite. The fraction of fiber reinforcement is very important in determining the overall mechanical properties of a composite. A higher fiber volume fraction typically results in better mechanical properties of the composite.
In mathematics and physics, homogenization is a method of studying partial differential equations with rapidly oscillating coefficients, such as

The theory of micro-mechanics of failure aims to explain the failure of continuous fiber reinforced composites by micro-scale analysis of stresses within each constituent material, and of the stresses at the interfaces between those constituents, calculated from the macro stresses at the ply level.

According to the classical theories of elastic or plastic structures made from a material with non-random strength (ft), the nominal strength (σN) of a structure is independent of the structure size (D) when geometrically similar structures are considered. Any deviation from this property is called the size effect. For example, conventional strength of materials predicts that a large beam and a tiny beam will fail at the same stress if they are made of the same material. In the real world, because of size effects, a larger beam will fail at a lower stress than a smaller beam.
cadec-online.com was a multilingual web application that performs analysis of composite materials and is used primarily for teaching, especially within the disciplines of aerospace engineering, materials science, naval engineering, mechanical engineering, and civil engineering. Users navigate the application through a tree view which structures the component chapters. cadec-online is an engineering cloud application. It uses the LaTeX library to render equations and symbols, then Sprites to optimize the delivery of images to the page. As of 2021, the application is no longer available.
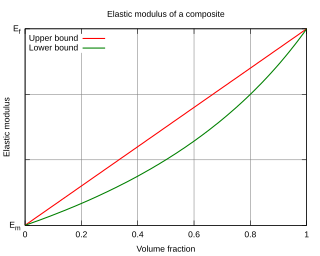
In materials science, a general rule of mixtures is a weighted mean used to predict various properties of a composite material. It provides a theoretical upper- and lower-bound on properties such as the elastic modulus, ultimate tensile strength, thermal conductivity, and electrical conductivity. In general there are two models, one for axial loading, and one for transverse loading.

James Henry Williams Jr. is a mechanical engineer, consultant, civic commentator, and teacher of engineering. He is currently Professor of Applied Mechanics in the Mechanical Engineering Department at the Massachusetts Institute of Technology (MIT). He is regarded as one of the world's leading experts in the mechanics, design, fabrication, and nondestructive evaluation (NDE) of nonmetallic fiber reinforced composite materials and structures. He is also Professor of Writing and Humanistic Studies at MIT.
Martin Ostoja-Starzewski is a Polish-Canadian-American scientist and engineer, a professor of mechanical science and engineering at the University of Illinois Urbana-Champaign. His research includes work on deterministic and stochastic mechanics: random and fractal media, representative elementary volume in linear and nonlinear material systems, universal elastic anisotropy index, random fields, and bridging continuum mechanics to fluctuation theorem.

The use of microstructures in 3D printing, where the thickness of each strut scale of tens of microns ranges from 0.2mm to 0.5mm, has the capabilities necessary to change the physical properties of objects (metamaterials) such as: elasticity, resistance, and hardness. In other words, these capabilities allow physical objects to become lighter or more flexible. The pattern has to adhere to geometric constraints, and thickness constraints, or can be enforced using optimization methods. Innovations in this field are being discovered in addition to 3D printers being built and researched with the intent to specialize in building structures needing altered physical properties.
Crystal plasticity is a mesoscale computational technique that takes into account crystallographic anisotropy in modelling the mechanical behaviour of polycrystalline materials. The technique has typically been used to study deformation through the process of slip, however, there are some flavors of crystal plasticity that can incorporate other deformation mechanisms like twinning and phase transformations. Crystal plasticity is used to obtain the relationship between stress and strain that also captures the underlying physics at the crystal level. Hence, it can be used to predict not just the stress-strain response of a material, but also the texture evolution, micromechanical field distributions, and regions of strain localisation. The two widely used formulations of crystal plasticity are the one based on the finite element method known as Crystal Plasticity Finite Element Method (CPFEM), which is developed based on the finite strain formulation for the mechanics, and a spectral formulation which is more computationally efficient due to the fast Fourier transform, but is based on the small strain formulation for the mechanics.
Indentation plastometry is the idea of using an indentation-based procedure to obtain (bulk) mechanical properties in the form of stress-strain relationships in the plastic regime. Since indentation is a much easier and more convenient procedure than conventional tensile testing, with far greater potential for mapping of spatial variations, this is an attractive concept.
















