Related Research Articles

Algebraic geometry is a branch of mathematics which uses abstract algebraic techniques, mainly from commutative algebra, to solve geometrical problems. Classically, it studies zeros of multivariate polynomials; the modern approach generalizes this in a few different aspects.
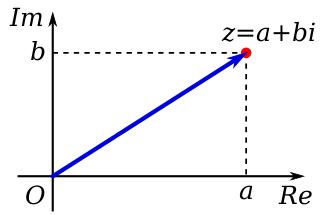
In mathematics, a complex number is an element of a number system that extends the real numbers with a specific element denoted i, called the imaginary unit and satisfying the equation ; every complex number can be expressed in the form , where a and b are real numbers. Because no real number satisfies the above equation, i was called an imaginary number by René Descartes. For the complex number ,a is called the real part, and b is called the imaginary part. The set of complex numbers is denoted by either of the symbols or C. Despite the historical nomenclature, "imaginary" complex numbers have a mathematical existence as firm as that of the real numbers, and they are fundamental tools in the scientific description of the natural world.

A number is a mathematical object used to count, measure, and label. The most basic examples are the natural numbers 1, 2, 3, 4, and so forth. Numbers can be represented in language with number words. More universally, individual numbers can be represented by symbols, called numerals; for example, "5" is a numeral that represents the number five. As only a relatively small number of symbols can be memorized, basic numerals are commonly organized in a numeral system, which is an organized way to represent any number. The most common numeral system is the Hindu–Arabic numeral system, which allows for the representation of any non-negative integer using a combination of ten fundamental numeric symbols, called digits. In addition to their use in counting and measuring, numerals are often used for labels, for ordering, and for codes. In common usage, a numeral is not clearly distinguished from the number that it represents.
Analysis is the branch of mathematics dealing with continuous functions, limits, and related theories, such as differentiation, integration, measure, infinite sequences, series, and analytic functions.

In mathematics, Galois theory, originally introduced by Évariste Galois, provides a connection between field theory and group theory. This connection, the fundamental theorem of Galois theory, allows reducing certain problems in field theory to group theory, which makes them simpler and easier to understand.
Hilbert's tenth problem is the tenth on the list of mathematical problems that the German mathematician David Hilbert posed in 1900. It is the challenge to provide a general algorithm that, for any given Diophantine equation, can decide whether the equation has a solution with all unknowns taking integer values.

Amalie Emmy Noether was a German mathematician who made many important contributions to abstract algebra. She proved Noether's first and second theorems, which are fundamental in mathematical physics. She was described by Pavel Alexandrov, Albert Einstein, Jean Dieudonné, Hermann Weyl and Norbert Wiener as the most important woman in the history of mathematics. As one of the leading mathematicians of her time, she developed theories of rings, fields, and algebras. In physics, Noether's theorem explains the connection between symmetry and conservation laws.

Oscar Zariski was an American mathematician. The Russian-born scientist was one of the most influential algebraic geometers of the 20th century.
Paul de Casteljau was a French physicist and mathematician. In 1959, while working at Citroën, he developed an algorithm for evaluating calculations on a certain family of curves, which would later be formalized and popularized by engineer Pierre Bézier, leading to the curves widely known as Bézier curves.

Mathematics during the Golden Age of Islam, especially during the 9th and 10th centuries, was built on Greek mathematics and Indian mathematics. Important progress was made, such as full development of the decimal place-value system to include decimal fractions, the first systematised study of algebra, and advances in geometry and trigonometry.
Joan Sylvia Lyttle Birman is an American mathematician, specializing in low-dimensional topology. She has made contributions to the study of knots, 3-manifolds, mapping class groups of surfaces, geometric group theory, contact structures and dynamical systems. Birman is research professor emerita at Barnard College, Columbia University, where she has been since 1973.
Arithmetic dynamics is a field that amalgamates two areas of mathematics, dynamical systems and number theory. Part of the inspiration comes from complex dynamics, the study of the iteration of self-maps of the complex plane or other complex algebraic varieties. Arithmetic dynamics is the study of the number-theoretic properties of integer, rational, p-adic, or algebraic points under repeated application of a polynomial or rational function. A fundamental goal is to describe arithmetic properties in terms of underlying geometric structures.
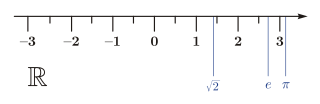
In mathematics, a real number is a number that can be used to measure a continuous one-dimensional quantity such as a distance, duration or temperature. Here, continuous means that pairs of values can have arbitrarily small differences. Every real number can be almost uniquely represented by an infinite decimal expansion.

In mathematics, the irrational numbers are all the real numbers that are not rational numbers. That is, irrational numbers cannot be expressed as the ratio of two integers. When the ratio of lengths of two line segments is an irrational number, the line segments are also described as being incommensurable, meaning that they share no "measure" in common, that is, there is no length, no matter how short, that could be used to express the lengths of both of the two given segments as integer multiples of itself.
In mathematics and computer science, computer algebra, also called symbolic computation or algebraic computation, is a scientific area that refers to the study and development of algorithms and software for manipulating mathematical expressions and other mathematical objects. Although computer algebra could be considered a subfield of scientific computing, they are generally considered as distinct fields because scientific computing is usually based on numerical computation with approximate floating point numbers, while symbolic computation emphasizes exact computation with expressions containing variables that have no given value and are manipulated as symbols.

In mathematical optimization, the criss-cross algorithm is any of a family of algorithms for linear programming. Variants of the criss-cross algorithm also solve more general problems with linear inequality constraints and nonlinear objective functions; there are criss-cross algorithms for linear-fractional programming problems, quadratic-programming problems, and linear complementarity problems.
Hermite's problem is an open problem in mathematics posed by Charles Hermite in 1848. He asked for a way of expressing real numbers as sequences of natural numbers, such that the sequence is eventually periodic precisely when the original number is a cubic irrational.
Ronald Neil Goldman is a Professor of Computer Science at Rice University in Houston, Texas. Professor Goldman received his B.S. in Mathematics from the Massachusetts Institute of Technology in 1968 and his M.A. and Ph.D. in Mathematics from Johns Hopkins University in 1973.
Felix Adalbert Behrend was a German mathematician of Jewish descent who escaped Nazi Germany and settled in Australia. His research interests included combinatorics, number theory, and topology. Behrend's theorem and Behrend sequences are named after him.
References
- 1 2 Birth year from Library of Congress catalog entry, retrieved 2024-04-05
- 1 2 "Thomas Garrity". Mathematics & Statistics. Retrieved February 20, 2023.
- ↑ Garrity, Thomas (December 2021). "Curriculum Vitae of Thomas A. Garrity" (PDF).
- ↑ "Thomas Garrity".
- ↑ Bajaj, Chanderjit; Canny, John; Garrity, Thomas; Warren, Joe (1989). "Factoring Rational Polynomials over the Complex Numbers" (PDF). SIAM Journal on Computing. 22 (2): 318–331. doi:10.1137/0222024. ISSN 0097-5397.
- ↑ Garrity, Thomas; Warren, Joe (February 1991). "Geometric continuity". Computer Aided Geometric Design. 8 (1): 51–65. doi:10.1016/0167-8396(91)90049-h. ISSN 0167-8396.
- 1 2 Garrity, Thomas (2001). "On periodic sequences for algebraic numbers". Journal of Number Theory. 88 (1): 86–103. arXiv: math/9906016 . doi:10.1006/jnth.2000.2608. MR 1825992.
- ↑ "MAA Awards Presented in Phoenix" (PDF). Notices of the American Mathematical Society. 51 (5): 544–545. May 2004.