Algebra tile

Last updated

Algebra tiles are mathematical manipulatives that allow students to better understand ways of algebraic thinking and the concepts of algebra. These tiles have proven to provide concrete models for elementary school, middle school, high school, and college-level introductory algebra students. They have also been used to prepare prison inmates for their General Educational Development (GED) tests. [1] Algebra tiles allow both an algebraic and geometric approach to algebraic concepts. They give students another way to solve algebraic problems other than just abstract manipulation. [1] The National Council of Teachers of Mathematics (NCTM) recommends a decreased emphasis on the memorization of the rules of algebra and the symbol manipulation of algebra in their Curriculum and Evaluation Standards for Mathematics. According to the NCTM 1989 standards "[r]elating models to one another builds a better understanding of each". [2]

Contents

Uses

Adding integers

Adding integers is the best place to start when one wants to get used to the idea of representing numbers with a quantity of tiles. Any integer can be represented by using the same number of tiles in the correct color. For example, for a 6 one could select six yellow tiles. For -3 one would select three red tiles. The tiles are usually double sided with yellow on one side and red on the other. This allows the student to grasp the powerful concept of "taking the opposite" of a negative, simply meaning the opposite. So one yellow tile is positive and the opposite (flip it over) is a negative one. This idea comes in handy when dealing with a - (-2). In order to work with a complex situation like this, start with two -1s (red side) and the extra negative means take the opposite or flip them over. - (-2) = 2.

When adding tiles, one should think of combining the quantities together. If one is adding 2 + 3, they are to combine two yellow tiles with three yellow tiles to make 5 yellow tiles. The same idea works for combining negative numbers. If one is to add -3 + -1, they are to combine negative three red tiles with one negative red tile to get negative four red tiles. -3 + -1 = -4.

When one adds positive numbers to negative numbers using algebra tiles, they need to bring in the idea of "elimination" or "zero pairs" every time they add a positive one to a negative one. This is true for any number of tiles, as long as the same quantity and opposite sign eliminate each other (or create a zero pair). For example, if one adds -5 + 7, they will combine five red tiles with seven yellow tiles. One can match the red and yellow tiles up one at a time to eliminate five of the yellow tiles to end up with two yellow tiles and zero red tiles. -5 + 7 = 2.

If one starts with more yellow tiles than red, the answer will be positive. If one starts with more red tiles than yellow, the answer will be negative.

One more example: -5 + 2. Five red tiles are being combines with two yellow tiles. The two yellow tiles will eliminate each other, (or form a zero pair) with two of the red tiles leaving three red tiles behind. -5 + 2 = -3.

Subtracting integers

Algebra tiles can also be used for subtracting integers. A person can take a problem such as and begin with a group of six unit tiles and then take three away to leave the student with three remaining, so then . Algebra tiles can also be used to solve problems like , which is equivalent to the problem . Being able to relate these two problems and why they result in the same answer is important because it shows that . Another way in which algebra tiles can be used for integer subtraction can be seen through looking at problems where one is to subtract a positive integer from a smaller positive integer, like . Here one would begin with five positive unit tiles and then add zero pairs to the five positive unit tiles until there were eight positive unit tiles. Adding the zero pairs will not change the value of the original five positive unit tiles. The student would then remove the eight positive unit tiles and count the number of negative unit tiles left. This number of negative unit tiles would then be the answer, which would be -3. [3]

Multiplication of integers

Multiplication of integers with algebra tiles is performed through forming a rectangle with the tiles. The length and width of the rectangle would be the two factors and then the total number of tiles in the rectangle would be the answer to the multiplication problem. For instance, in order to determine 3 × 4, one would take three positive unit tiles to represent three rows in the rectangle and then there would be four positive unit tiles to represent the columns in the rectangle. This would lead to having a rectangle with four columns of three positive unit tiles, which represents 3×4. Now, the student may count the number of unit tiles in the rectangle, which will equal 12.

Modeling and simplifying algebraic expressions

Modeling algebraic expressions with algebra tiles is very similar to modeling addition and subtraction of integers using algebra tiles. In an expression such as one would group five positive x tiles together and then three negative unit tiles together to represent this algebraic expression. Along with modeling these expressions, algebra tiles can also be used to simplify algebraic expressions. For instance, if one has they can combine the positive and negative x tiles and unit tiles to form zero pairs to leave the student with the expression . Since the tiles are laid out right in front of the student, it is easy to combine the like terms, or the terms that represent the same type of tile. [3]

The distributive property is modeled through the algebra tiles by demonstrating that a(b+c)=(a×b)+(a×c). One would want to model what is being represented on both sides of the equation separately and determine that they are both equal to each other. If one wants to show that then they would make three sets of one unit tile and one x tile and then combine them together to see if it results in , which it does. [4]

Solving linear equations using addition

The linear equation can be modeled with one positive tile and eight negative unit tiles on the left side of a piece of paper and six positive unit tiles on the right side. To maintain equality of the sides, each action must be performed on both sides. [1] For example, eight positive unit tiles can be added to both sides. [1] Zero pairs of unit tiles are removed from the left side, leaving one positive tile. The right side has 14 positive unit tiles, so .

Solving linear equations using subtraction

The equation can be modeled with one positive tile and seven positive unit tiles on the left side and 10 positive unit tiles on the right side. Rather than adding the same number of tiles to both sides, the same number of tiles can be subtracted from both sides. For example, seven positive unit tiles can be removed from both sides. This leaves one positive tile on the left side and three positive unit tiles on the right side, so . [1]

Solving linear systems

Linear systems of equations may be solved algebraically by isolating one of the variables and then performing a substitution. Isolating a variable can be modeled with algebra tiles in a manner similar to solving linear equations (above), and substitution can be modeled with algebra tiles by replacing tiles with other tiles.

Multiplying polynomials

When using algebra tiles to multiply a monomial by a monomial, the student must first set up a rectangle where the length of the rectangle is the one monomial and then the width of the rectangle is the other monomial, similar to when one multiplies integers using algebra tiles. Once the sides of the rectangle are represented by the algebra tiles, one would then try to figure out which algebra tiles would fill in the rectangle. For instance, if one had x×x, the only algebra tile that would complete the rectangle would be x2, which is the answer.

Multiplication of binomials is similar to multiplication of monomials when using the algebra tiles . Multiplication of binomials can also be thought of as creating a rectangle where the factors are the length and width. [2] As with the monomials, one would set up the sides of the rectangle to be the factors and then fill in the rectangle with the algebra tiles. [2] This method of using algebra tiles to multiply polynomials is known as the area model [5] and it can also be applied to multiplying monomials and binomials with each other. An example of multiplying binomials is (2x+1)×(x+2) and the first step the student would take is set up two positive x tiles and one positive unit tile to represent the length of a rectangle and then one would take one positive x tile and two positive unit tiles to represent the width. These two lines of tiles would create a space that looks like a rectangle which can be filled in with certain tiles. In the case of this example the rectangle would be composed of two positive x2 tiles, five positive x tiles, and two positive unit tiles. So the solution is 2x2+5x+2.

Factoring

Algebra tile model of x 2 + 3 x + 2 {\displaystyle x^{2}+3x+2} Algebra tile factoring.jpg
Algebra tile model of

In order to factor using algebra tiles, one has to start out with a set of tiles that the student combines into a rectangle, this may require the use of adding zero pairs in order to make the rectangular shape. An example would be where one is given one positive x2 tile, three positive x tiles, and two positive unit tiles. The student forms the rectangle by having the x2 tile in the upper right corner, then one has two x tiles on the right side of the x2 tile, one x tile underneath the x2 tile, and two unit tiles are in the bottom right corner. By placing the algebra tiles to the sides of this rectangle we can determine that we need one positive x tile and one positive unit tile for the length and then one positive x tile and two positive unit tiles for the width. This means that the two factors are and . [1] In a sense this is the reverse of the procedure for multiplying polynomials.

Completing the square

The process of completing the square can be accomplished using algebra tiles by placing the x2 tiles and x tiles into a square. One will not be able to completely create the square because there will be a smaller square missing from the larger square the student made from the tiles they were given, which will be filled in by the unit tiles. To complete the square, the student would determine how many unit tiles would be needed to fill in the missing square. To complete the square of x2+6x, one would start off with one positive x2 tile and six positive x tiles. Then, they would place the x2 tile in the upper left corner and then one would place three positive x tiles to the right of the x2 tile and three positive unit x tiles under the x2 tile. In order to fill in the square we need nine positive unit tiles. we have now created x2+6x+9, which can be factored into . [6]

Related Research Articles

Arithmetic Elementary branch of mathematics

Arithmetic is a branch of mathematics that consists of the study of numbers, especially concerning the properties of the traditional operations on them—addition, subtraction, multiplication, division, exponentiation and extraction of roots. Arithmetic is an elementary part of number theory, and number theory is considered to be one of the top-level divisions of modern mathematics, along with algebra, geometry, and analysis. The terms arithmetic and higher arithmetic were used until the beginning of the 20th century as synonyms for number theory, and are sometimes still used to refer to a wider part of number theory.

In mathematics, an equation is a statement that asserts the equality of two expressions, which are connected by the equals sign "=". The word equation and its cognates in other languages may have subtly different meanings; for example, in French an équation is defined as containing one or more variables, while in English, any equality is an equation.

Euclidean algorithm Algorithm for computing greatest common divisors

In mathematics, the Euclidean algorithm, or Euclid's algorithm, is an efficient method for computing the greatest common divisor (GCD) of two integers (numbers), the largest number that divides them both without a remainder. It is named after the ancient Greek mathematician Euclid, who first described it in his Elements . It is an example of an algorithm, a step-by-step procedure for performing a calculation according to well-defined rules, and is one of the oldest algorithms in common use. It can be used to reduce fractions to their simplest form, and is a part of many other number-theoretic and cryptographic calculations.

Integer Number in {..., –2, –1, 0, 1, 2, ...}

An integer is colloquially defined as a number that can be written without a fractional component. For example, 21, 4, 0, and −2048 are integers, while 9.75, 5+1/2, and √2 are not.

Multiplication Arithmetical operation

Multiplication is one of the four elementary mathematical operations of arithmetic, with the other ones being addition, subtraction, and division. The result of a multiplication operation is called a product.

Number Mathematical description of the common concept

A number is a mathematical object used to count, measure, and label. The original examples are the natural numbers 1, 2, 3, 4, and so forth. Numbers can be represented in language with number words. More universally, individual numbers can be represented by symbols, called numerals; for example, "5" is a numeral that represents the number five. As only a relatively small number of symbols can be memorized, basic numerals are commonly organized in a numeral system, which is an organized way to represent any number. The most common numeral system is the Hindu–Arabic numeral system, which allows for the representation of any number using a combination of ten fundamental numeric symbols, called digits. In addition to their use in counting and measuring, numerals are often used for labels, for ordering, and for codes. In common usage, a numeral is not clearly distinguished from the number that it represents.

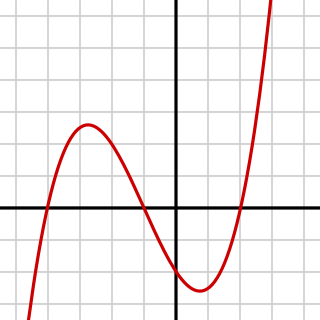
Polynomial In mathematics, sum of products of variables, power of variables, and coefficients

In mathematics, a polynomial is an expression consisting of indeterminates and coefficients, that involves only the operations of addition, subtraction, multiplication, and non-negative integer exponentiation of variables. An example of a polynomial of a single indeterminate x is x2 − 4x + 7. An example in three variables is x3 + 2xyz2 − yz + 1.

Square root Number whose square is a given number

In mathematics, a square root of a number x is a number y such that y2 = x; in other words, a number y whose square (the result of multiplying the number by itself, or y ⋅ y) is x. For example, 4 and −4 are square roots of 16, because 42 = (−4)2 = 16. Every nonnegative real number x has a unique nonnegative square root, called the principal square root, which is denoted by where the symbol is called the radical sign or radix. For example, the principal square root of 9 is 3, which is denoted by because 32 = 3 ⋅ 3 = 9 and 3 is nonnegative. The term (or number) whose square root is being considered is known as the radicand. The radicand is the number or expression underneath the radical sign, in this case 9.

In mathematics, the discriminant of a polynomial is a quantity that depends on the coefficients and determines various properties of the roots. It is generally defined as a polynomial function of the coefficients of the original polynomial. The discriminant is widely used in polynomial factoring, number theory, and algebraic geometry. It is often denoted by the symbol .

In mathematics, a Laurent polynomial in one variable over a field is a linear combination of positive and negative powers of the variable with coefficients in . Laurent polynomials in X form a ring denoted [X, X−1]. They differ from ordinary polynomials in that they may have terms of negative degree. The construction of Laurent polynomials may be iterated, leading to the ring of Laurent polynomials in several variables. Laurent polynomials are of particular importance in the study of complex variables.

Exponentiation Mathematical operation

Exponentiation is a mathematical operation, written as bn, involving two numbers, the baseb and the exponent or powern, and pronounced as "b raised to the power of n". When n is a positive integer, exponentiation corresponds to repeated multiplication of the base: that is, bn is the product of multiplying n bases:

Division by zero Class of mathematical expression

In mathematics, division by zero is division where the divisor (denominator) is zero. Such a division can be formally expressed as where a is the dividend (numerator). In ordinary arithmetic, the expression has no meaning, as there is no number which, when multiplied by 0, gives a, and so division by zero is undefined. Since any number multiplied by zero is zero, the expression is also undefined; when it is the form of a limit, it is an indeterminate form. Historically, one of the earliest recorded references to the mathematical impossibility of assigning a value to is contained in Anglo-Irish philosopher George Berkeley’s criticism of infinitesimal calculus in 1734 in The Analyst.

Multiplicative inverse Number which when multiplied by x equals 1

In mathematics, a multiplicative inverse or reciprocal for a number x, denoted by 1/x or x−1, is a number which when multiplied by x yields the multiplicative identity, 1. The multiplicative inverse of a fraction a/b is b/a. For the multiplicative inverse of a real number, divide 1 by the number. For example, the reciprocal of 5 is one fifth, and the reciprocal of 0.25 is 1 divided by 0.25, or 4. The reciprocal function, the function f(x) that maps x to 1/x, is one of the simplest examples of a function which is its own inverse.

Brahmagupta was an Indian mathematician and astronomer. He is the author of two early works on mathematics and astronomy: the Brāhmasphuṭasiddhānta, a theoretical treatise, and the Khaṇḍakhādyaka, a more practical text.

In mathematics, and more specifically in computer algebra, computational algebraic geometry, and computational commutative algebra, a Gröbner basis is a particular kind of generating set of an ideal in a polynomial ring K[x1, …, xn] over a field K. A Gröbner basis allows many important properties of the ideal and the associated algebraic variety to be deduced easily, such as the dimension and the number of zeros when it is finite. Gröbner basis computation is one of the main practical tools for solving systems of polynomial equations and computing the images of algebraic varieties under projections or rational maps.

In mathematics, a monomial is, roughly speaking, a polynomial which has only one term. Two definitions of a monomial may be encountered:

  1. A monomial, also called power product, is a product of powers of variables with nonnegative integer exponents, or, in other words, a product of variables, possibly with repetitions. For example, is a monomial. The constant is a monomial, being equal to the empty product and to for any variable . If only a single variable is considered, this means that a monomial is either or a power of , with a positive integer. If several variables are considered, say, then each can be given an exponent, so that any monomial is of the form with non-negative integers.
  2. A monomial is a monomial in the first sense multiplied by a nonzero constant, called the coefficient of the monomial. A monomial in the first sense is a special case of a monomial in the second sense, where the coefficient is . For example, in this interpretation and are monomials.
Polynomial ring Algebraic structure

In mathematics, especially in the field of algebra, a polynomial ring or polynomial algebra is a ring formed from the set of polynomials in one or more indeterminates with coefficients in another ring, often a field.

Square (algebra) Result of multiplying a number, or other expression, by itself

In mathematics, a square is the result of multiplying a number by itself. The verb "to square" is used to denote this operation. Squaring is the same as raising to the power 2, and is denoted by a superscript 2; for instance, the square of 3 may be written as 32, which is the number 9. In some cases when superscripts are not available, as for instance in programming languages or plain text files, the notations x^2 or x**2 may be used in place of x2.

Fraction Mathematical representation of a portion of a whole

A fraction represents a part of a whole or, more generally, any number of equal parts. When spoken in everyday English, a fraction describes how many parts of a certain size there are, for example, one-half, eight-fifths, three-quarters. A common, vulgar, or simple fraction consists of a numerator displayed above a line, and a non-zero denominator, displayed below that line. Numerators and denominators are also used in fractions that are not common, including compound fractions, complex fractions, and mixed numerals.

Sign (mathematics)

In mathematics, the sign of a real number is its property of being either positive, negative, or zero. Depending on local conventions, zero may be considered as being neither positive nor negative, or it may be considered both positive and negative. Whenever not specifically mentioned, this article adheres to the first convention.

References

  1. 1 2 3 4 5 6 Kitt 2000.
  2. 1 2 3 Stein 2000.
  3. 1 2 "Prentice Hall School" (PDF). Phschool.com. Archived from the original (PDF) on 2012-02-12. Retrieved 2013-07-22.
  4. ↑ Archived May 16, 2008, at the Wayback Machine
  5. ↑ Larson R: "Algebra 1", page 516. McDougal Littell, 1998.
  6. ↑ Donna Roberts. "Using Algebra Tiles to Complete the Square". Regentsprep.org. Archived from the original on 2013-08-18. Retrieved 2013-07-22.

Sources

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.