A hash function is any function that can be used to map data of arbitrary size to fixed-size values, though there are some hash functions that support variable length output. The values returned by a hash function are called hash values, hash codes, digests, or simply hashes. The values are usually used to index a fixed-size table called a hash table. Use of a hash function to index a hash table is called hashing or scatter storage addressing.

In computing, a hash table, also known as hash map, is a data structure that implements an associative array or dictionary. It is an abstract data type that maps keys to values. A hash table uses a hash function to compute an index, also called a hash code, into an array of buckets or slots, from which the desired value can be found. During lookup, the key is hashed and the resulting hash indicates where the corresponding value is stored.

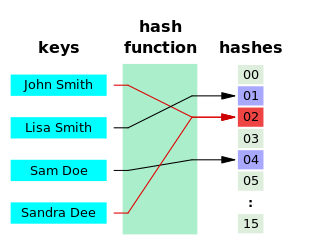
In computer science, a perfect hash functionh for a set S is a hash function that maps distinct elements in S to a set of m integers, with no collisions. In mathematical terms, it is an injective function.
In computer science, a one-way function is a function that is easy to compute on every input, but hard to invert given the image of a random input. Here, "easy" and "hard" are to be understood in the sense of computational complexity theory, specifically the theory of polynomial time problems. Not being one-to-one is not considered sufficient for a function to be called one-way.
A randomized algorithm is an algorithm that employs a degree of randomness as part of its logic or procedure. The algorithm typically uses uniformly random bits as an auxiliary input to guide its behavior, in the hope of achieving good performance in the "average case" over all possible choices of random determined by the random bits; thus either the running time, or the output are random variables.
In computational complexity theory, the 3SUM problem asks if a given set of real numbers contains three elements that sum to zero. A generalized version, k-SUM, asks the same question on k numbers. 3SUM can be easily solved in time, and matching lower bounds are known in some specialized models of computation.

Linear probing is a scheme in computer programming for resolving collisions in hash tables, data structures for maintaining a collection of key–value pairs and looking up the value associated with a given key. It was invented in 1954 by Gene Amdahl, Elaine M. McGraw, and Arthur Samuel and first analyzed in 1963 by Donald Knuth.

Cuckoo hashing is a scheme in computer programming for resolving hash collisions of values of hash functions in a table, with worst-case constant lookup time. The name derives from the behavior of some species of cuckoo, where the cuckoo chick pushes the other eggs or young out of the nest when it hatches in a variation of the behavior referred to as brood parasitism; analogously, inserting a new key into a cuckoo hashing table may push an older key to a different location in the table.
In mathematics and computing, universal hashing refers to selecting a hash function at random from a family of hash functions with a certain mathematical property. This guarantees a low number of collisions in expectation, even if the data is chosen by an adversary. Many universal families are known, and their evaluation is often very efficient. Universal hashing has numerous uses in computer science, for example in implementations of hash tables, randomized algorithms, and cryptography.
In computer science, locality-sensitive hashing (LSH) is an algorithmic technique that hashes similar input items into the same "buckets" with high probability. Since similar items end up in the same buckets, this technique can be used for data clustering and nearest neighbor search. It differs from conventional hashing techniques in that hash collisions are maximized, not minimized. Alternatively, the technique can be seen as a way to reduce the dimensionality of high-dimensional data; high-dimensional input items can be reduced to low-dimensional versions while preserving relative distances between items.
In computer science, streaming algorithms are algorithms for processing data streams in which the input is presented as a sequence of items and can be examined in only a few passes, typically just one. These algorithms are designed to operate with limited memory, generally logarithmic in the size of the stream and/or in the maximum value in the stream, and may also have limited processing time per item.
In computer science and graph theory, the term color-coding refers to an algorithmic technique which is useful in the discovery of network motifs. For example, it can be used to detect a simple path of length k in a given graph. The traditional color-coding algorithm is probabilistic, but it can be derandomized without much overhead in the running time.
In cryptography, SWIFFT is a collection of provably secure hash functions. It is based on the concept of the fast Fourier transform (FFT). SWIFFT is not the first hash function based on FFT, but it sets itself apart by providing a mathematical proof of its security. It also uses the LLL basis reduction algorithm. It can be shown that finding collisions in SWIFFT is at least as difficult as finding short vectors in cyclic/ideal lattices in the worst case. By giving a security reduction to the worst-case scenario of a difficult mathematical problem, SWIFFT gives a much stronger security guarantee than most other cryptographic hash functions.
In discrete mathematics, ideal lattices are a special class of lattices and a generalization of cyclic lattices. Ideal lattices naturally occur in many parts of number theory, but also in other areas. In particular, they have a significant place in cryptography. Micciancio defined a generalization of cyclic lattices as ideal lattices. They can be used in cryptosystems to decrease by a square root the number of parameters necessary to describe a lattice, making them more efficient. Ideal lattices are a new concept, but similar lattice classes have been used for a long time. For example, cyclic lattices, a special case of ideal lattices, are used in NTRUEncrypt and NTRUSign.
In computer science and data mining, MinHash is a technique for quickly estimating how similar two sets are. The scheme was invented by Andrei Broder (1997), and initially used in the AltaVista search engine to detect duplicate web pages and eliminate them from search results. It has also been applied in large-scale clustering problems, such as clustering documents by the similarity of their sets of words.
In computer science, integer sorting is the algorithmic problem of sorting a collection of data values by integer keys. Algorithms designed for integer sorting may also often be applied to sorting problems in which the keys are floating point numbers, rational numbers, or text strings. The ability to perform integer arithmetic on the keys allows integer sorting algorithms to be faster than comparison sorting algorithms in many cases, depending on the details of which operations are allowed in the model of computing and how large the integers to be sorted are.
In mathematics, a submodular set function is a set function whose value, informally, has the property that the difference in the incremental value of the function that a single element makes when added to an input set decreases as the size of the input set increases. Submodular functions have a natural diminishing returns property which makes them suitable for many applications, including approximation algorithms, game theory and electrical networks. Recently, submodular functions have also found immense utility in several real world problems in machine learning and artificial intelligence, including automatic summarization, multi-document summarization, feature selection, active learning, sensor placement, image collection summarization and many other domains.
In computer science, tabulation hashing is a method for constructing universal families of hash functions by combining table lookup with exclusive or operations. It was first studied in the form of Zobrist hashing for computer games; later work by Carter and Wegman extended this method to arbitrary fixed-length keys. Generalizations of tabulation hashing have also been developed that can handle variable-length keys such as text strings.
In cryptography, an accumulator is a one way membership hash function. It allows users to certify that potential candidates are a member of a certain set without revealing the individual members of the set. This concept was formally introduced by Josh Benaloh and Michael de Mare in 1993.
In mathematics and computer science, polynomial evaluation refers to computation of the value of a polynomial when its indeterminates are substituted for some values. In other words, evaluating the polynomial at consists of computing See also Polynomial ring § Polynomial evaluation
































