Quasicrystals and Geometry

Last updated

Quasicrystals and Geometry is a book on quasicrystals and aperiodic tiling by Marjorie Senechal, published in 1995 by Cambridge University Press ( ISBN   0-521-37259-3). [1] [2] [3] [4] [5]

Contents

One of the main themes of the book is to understand how the mathematical properties of aperiodic tilings such as the Penrose tiling, and in particular the existence of arbitrarily large patches of five-way rotational symmetry throughout these tilings, correspond to the properties of quasicrystals including the five-way symmetry of their Bragg peaks. Neither kind of symmetry is possible for a traditional periodic tiling or periodic crystal structure, and the interplay between these topics led from the 1960s into the 1990s to new developments and new fundamental definitions in both mathematics and crystallography. [3]

Topics

The book is divided into two parts. The first part covers the history of crystallography, the use of X-ray diffraction to study crystal structures through the Bragg peaks formed on their diffraction patterns, and the discovery in the early 1980s of quasicrystals, materials that form Bragg peaks in patterns with five-way symmetry, impossible for a repeating crystal structure. It models the arrangement of atoms in a substance by a Delone set, a set of points in the plane or in Euclidean space that are neither too closely spaced nor too far apart, and it discusses the mathematical and computational issues in X-ray diffraction and the construction of the diffraction spectrum from a Delone set. Finally, it discusses a method for constructing Delone sets that have Bragg peaks by projecting bounded subsets of higher-dimensional lattices into lower-dimensional spaces. [2] This material also has strong connections to spectral theory and ergodic theory, deep topics in pure mathematics, but these were omitted in order to make the book accessible to non-specialists in those topics. [3]

Another method for the construction of Delone sets that have Bragg peaks is to choose as points the vertices of certain aperiodic tilings such as the Penrose tiling. [2] (There also exist other aperiodic tilings, such as the pinwheel tiling, for which the existence of discrete peaks in the diffraction pattern is less clear.) [1] The second part of the book discusses methods for generating these tilings, including projections of higher-dimensional lattices as well as recursive constructions with hierarchical structure, and it discusses the long-range patterns that can be shown to exist in tilings constructed in these ways. [2]

Included in the book are software for generating diffraction patterns and Penrose tilings, and a "pictorial atlas" of the diffraction patterns of known aperiodic tilings. [4]

Audience

Although the discovery of quasicrystals immediately set off a rush for applications in materials capable of withstanding high temperature, providing non-stick surfaces, or having other useful material properties, this book is more abstract and mathematical, and concerns mathematical models of quasicrystals rather than physical materials. Nevertheless, chemist István Hargittai writes that it can be read with interest by "students and researchers in mathematics, physics, materials science, and crystallography". [5]

Related Research Articles

Crystallography scientific study of crystal structure

Crystallography is the experimental science of determining the arrangement of atoms in crystalline solids. The word "crystallography" is derived from the Greek words crystallon "cold drop, frozen drop", with its meaning extending to all solids with some degree of transparency, and graphein "to write". In July 2012, the United Nations recognised the importance of the science of crystallography by proclaiming that 2014 would be the International Year of Crystallography.

Quasicrystal Chemical structure

A quasiperiodic crystal, or quasicrystal, is a structure that is ordered but not periodic. A quasicrystalline pattern can continuously fill all available space, but it lacks translational symmetry. While crystals, according to the classical crystallographic restriction theorem, can possess only two-, three-, four-, and six-fold rotational symmetries, the Bragg diffraction pattern of quasicrystals shows sharp peaks with other symmetry orders—for instance, five-fold.

X-ray crystallography Technique used in studying crystal structure

X-ray crystallography (XRC) is the experimental science determining the atomic and molecular structure of a crystal, in which the crystalline structure causes a beam of incident X-rays to diffract into many specific directions. By measuring the angles and intensities of these diffracted beams, a crystallographer can produce a three-dimensional picture of the density of electrons within the crystal. From this electron density, the mean positions of the atoms in the crystal can be determined, as well as their chemical bonds, their crystallographic disorder, and various other information.

Tessellation Tiling of a plane using one or more geometric shapes, called tiles, with no overlaps and no gaps

A tiling or tessellation of a flat surface is the covering of a plane using one or more geometric shapes, called tiles, with no overlaps and no gaps. In mathematics, tessellations can be generalized to higher dimensions and a variety of geometries.

Aperiodic tiling Non-periodic tiling with the additional property that it does not contain arbitrarily large periodic patches

An aperiodic tiling is a non-periodic tiling with the additional property that it does not contain arbitrarily large periodic patches. A set of tile-types is aperiodic if copies of these tiles can form only non-periodic tilings. The Penrose tilings are the best-known examples of aperiodic tilings.

Robert Ammann American mathematician

Robert Ammann was an amateur mathematician who made several significant and groundbreaking contributions to the theory of quasicrystals and aperiodic tilings.

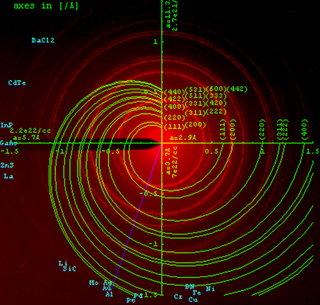
Powder diffraction

Powder diffraction is a scientific technique using X-ray, neutron, or electron diffraction on powder or microcrystalline samples for structural characterization of materials. An instrument dedicated to performing such powder measurements is called a powder diffractometer.

In geometry, a tile substitution is a method for constructing highly ordered tilings. Most importantly, some tile substitutions generate aperiodic tilings, which are tilings whose prototiles do not admit any tiling with translational symmetry. The most famous of these are the Penrose tilings. Substitution tilings are special cases of finite subdivision rules, which do not require the tiles to be geometrically rigid.

Ammann–Beenker tiling

In geometry, an Ammann–Beenker tiling is a nonperiodic tiling which can be generated either by an aperiodic set of prototiles as done by Robert Ammann in the 1970s, or by the cut-and-project method as done independently by F. P. M. Beenker. Because all tilings obtained with the tiles are non-periodic, Ammann–Beenker tilings are considered aperiodic tilings. They are one of the five sets of tilings discovered by Ammann and described in Tilings and Patterns.

Phason is a quasiparticle existing in quasicrystals due to their specific, quasiperiodic lattice structure. Similar to phonon, phason is associated with atomic motion. However, whereas phonons are related to translation of atoms, phasons are associated with atomic rearrangements. As a result of these rearrangements, waves, describing the position of atoms in crystal, change phase, thus the term "phason".

Penrose tiling Non-periodic tiling of the plane

A Penrose tiling is an example of an aperiodic tiling. Here, a tiling is a covering of the plane by non-overlapping polygons or other shapes, and aperiodic means that shifting any tiling with these shapes by any finite distance, without rotation, cannot produce the same tiling. However, despite their lack of translational symmetry, Penrose tilings may have both reflection symmetry and fivefold rotational symmetry. Penrose tilings are named after mathematician and physicist Roger Penrose, who investigated them in the 1970s.

A Euclidean graph is periodic if there exists a basis of that Euclidean space whose corresponding translations induce symmetries of that graph. Equivalently, a periodic Euclidean graph is a periodic realization of an abelian covering graph over a finite graph. A Euclidean graph is uniformly discrete if there is a minimal distance between any two vertices. Periodic graphs are closely related to tessellations of space and the geometry of their symmetry groups, hence to geometric group theory, as well as to discrete geometry and the theory of polytopes, and similar areas.

Pythagorean tiling

A Pythagorean tiling or two squares tessellation is a tiling of a Euclidean plane by squares of two different sizes, in which each square touches four squares of the other size on its four sides. Many proofs of the Pythagorean theorem are based on it, explaining its name. It is commonly used as a pattern for floor tiles. When used for this, it is also known as a hopscotch pattern or pinwheel pattern, but it should not be confused with the mathematical pinwheel tiling, an unrelated pattern.

Fibonacci quasicrystal

A Fibonacci crystal or quasicrystal is a model used to study systems with aperiodic structure. Both names are acceptable as a 'Fibonacci crystal' denotes a quasicrystal and a 'Fibonacci' quasicrystal is a specific type of quasicrystal. Fibonacci 'chains' or 'lattices' are closely related terms, depending on the dimension of the model. While it is used mostly as a theoretical construct, physical models are implemented in order to verify the concept empirically. Most of its applications pertain to various areas of solid state physics.

Alan Lindsay Mackay

Alan Lindsay Mackay FRS is a British crystallographer, born in Wolverhampton.

Marjorie Lee Senechal is an American mathematician and historian of science, the Louise Wolff Kahn Professor Emerita in Mathematics and History of Science and Technology at Smith College and editor-in-chief of The Mathematical Intelligencer. In mathematics, she is known for her work on tessellations and quasicrystals; she has also studied ancient Parthian electric batteries and published several books about silk.

Aperiodic set of prototiles

A set of prototiles is aperiodic if copies of the prototiles can be assembled to create tilings, such that all possible tessellation patterns are non-periodic. The aperiodicity referred to is a property of the particular set of prototiles; the various resulting tilings themselves are just non-periodic.

In plane geometry, the einstein problem asks about the existence of a single prototile that by itself forms an aperiodic set of prototiles, that is, a shape that can tessellate space, but only in a nonperiodic way. Such a shape is called an "einstein", a play on the German words ein Stein, meaning one tile. Depending on the particular definitions of nonperiodicity and the specifications of what sets may qualify as tiles and what types of matching rules are permitted, the problem is either open or solved. The einstein problem can be seen as a natural extension of the second part of Hilbert's eighteenth problem, which asks for a single polyhedron that tiles Euclidean 3-space, but such that no tessellation by this polyhedron is isohedral. Such anisohedral tiles were found by Karl Reinhardt in 1928, but these anisohedral tiles all tile space periodically.

References

  1. 1 2 Cahn, John W. (November 1995), "Crystallography expanded", Science , 270 (5237): 839–842, doi:10.1126/science.270.5237.839, JSTOR   2888935, S2CID   220110430
  2. 1 2 3 4 Kenyon, Richard (1996), Mathematical Reviews, MR   1340198 CS1 maint: untitled periodical (link)
  3. 1 2 3 Radin, Charles (April 1996), "Book Review: Quasicrystals and geometry" (PDF), Notices of the American Mathematical Society , 43 (4): 416–421
  4. 1 2 Hayes, Brian (July–August 1996), American Scientist , 84 (4): 404–405, JSTOR   29775727 CS1 maint: untitled periodical (link)
  5. 1 2 Hargittai, István (1997), "Critics on crystals", Advanced Materials , 9 (12): 994–996, doi:10.1002/adma.19970091217
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.