
In mathematical physics and mathematics, the Pauli matrices are a set of three 2 × 2 complex matrices which are Hermitian, involutory and unitary. Usually indicated by the Greek letter sigma, they are occasionally denoted by tau when used in connection with isospin symmetries.
In particle physics, the Dirac equation is a relativistic wave equation derived by British physicist Paul Dirac in 1928. In its free form, or including electromagnetic interactions, it describes all spin-1⁄2 massive particles, called "Dirac particles", such as electrons and quarks for which parity is a symmetry. It is consistent with both the principles of quantum mechanics and the theory of special relativity, and was the first theory to account fully for special relativity in the context of quantum mechanics. It was validated by accounting for the fine structure of the hydrogen spectrum in a completely rigorous way.
In special relativity, four-momentum (also called momentum–energy or momenergy) is the generalization of the classical three-dimensional momentum to four-dimensional spacetime. Momentum is a vector in three dimensions; similarly four-momentum is a four-vector in spacetime. The contravariant four-momentum of a particle with relativistic energy E and three-momentum p = (px, py, pz) = γmv, where v is the particle's three-velocity and γ the Lorentz factor, is

In special relativity, a four-vector is an object with four components, which transform in a specific way under Lorentz transformations. Specifically, a four-vector is an element of a four-dimensional vector space considered as a representation space of the standard representation of the Lorentz group, the representation. It differs from a Euclidean vector in how its magnitude is determined. The transformations that preserve this magnitude are the Lorentz transformations, which include spatial rotations and boosts.
In mathematics, conformal geometry is the study of the set of angle-preserving (conformal) transformations on a space.
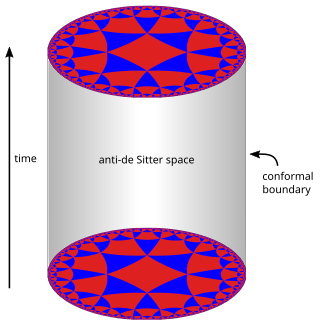
In mathematics and physics, n-dimensional anti-de Sitter space (AdSn) is a maximally symmetric Lorentzian manifold with constant negative scalar curvature. Anti-de Sitter space and de Sitter space are named after Willem de Sitter (1872–1934), professor of astronomy at Leiden University and director of the Leiden Observatory. Willem de Sitter and Albert Einstein worked together closely in Leiden in the 1920s on the spacetime structure of the universe. Paul Dirac was the first person to rigorously explore anti-de Sitter space, doing so in 1963.
In geometry and complex analysis, a Möbius transformation of the complex plane is a rational function of the form
A conformal field theory (CFT) is a quantum field theory that is invariant under conformal transformations. In two dimensions, there is an infinite-dimensional algebra of local conformal transformations, and conformal field theories can sometimes be exactly solved or classified.

In mathematics, the conformal group of an inner product space is the group of transformations from the space to itself that preserve angles. More formally, it is the group of transformations that preserve the conformal geometry of the space.
In mathematics, a linear fractional transformation is, roughly speaking, an invertible transformation of the form
In mathematical physics, the conformal symmetry of spacetime is expressed by an extension of the Poincaré group, known as the conformal group. The extension includes special conformal transformations and dilations. In three spatial plus one time dimensions, conformal symmetry has 15 degrees of freedom: ten for the Poincaré group, four for special conformal transformations, and one for a dilation.
In mathematics, the projective line over a ring is an extension of the concept of projective line over a field. Given a ring A with 1, the projective line P(A) over A consists of points identified by projective coordinates. Let U be the group of units of A; pairs (a, b) and (c, d) from A × A are related when there is a u in U such that ua = c and ub = d. This relation is an equivalence relation. A typical equivalence class is written U[a, b].

In nuclear physics, the chiral model, introduced by Feza Gürsey in 1960, is a phenomenological model describing effective interactions of mesons in the chiral limit (where the masses of the quarks go to zero), but without necessarily mentioning quarks at all. It is a nonlinear sigma model with the principal homogeneous space of a Lie group as its target manifold. When the model was originally introduced, this Lie group was the SU(N), where N is the number of quark flavors. The Riemannian metric of the target manifold is given by a positive constant multiplied by the Killing form acting upon the Maurer–Cartan form of SU(N).
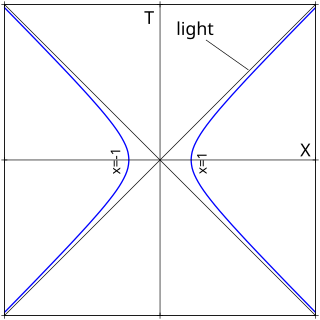
Hyperbolic motion is the motion of an object with constant proper acceleration in special relativity. It is called hyperbolic motion because the equation describing the path of the object through spacetime is a hyperbola, as can be seen when graphed on a Minkowski diagram whose coordinates represent a suitable inertial (non-accelerated) frame. This motion has several interesting features, among them that it is possible to outrun a photon if given a sufficient head start, as may be concluded from the diagram.
In projective geometry, a homography is an isomorphism of projective spaces, induced by an isomorphism of the vector spaces from which the projective spaces derive. It is a bijection that maps lines to lines, and thus a collineation. In general, some collineations are not homographies, but the fundamental theorem of projective geometry asserts that is not so in the case of real projective spaces of dimension at least two. Synonyms include projectivity, projective transformation, and projective collineation.

The covariant formulation of classical electromagnetism refers to ways of writing the laws of classical electromagnetism in a form that is manifestly invariant under Lorentz transformations, in the formalism of special relativity using rectilinear inertial coordinate systems. These expressions both make it simple to prove that the laws of classical electromagnetism take the same form in any inertial coordinate system, and also provide a way to translate the fields and forces from one frame to another. However, this is not as general as Maxwell's equations in curved spacetime or non-rectilinear coordinate systems.

In theoretical physics, the BPST instanton is the instanton with winding number 1 found by Alexander Belavin, Alexander Polyakov, Albert Schwarz and Yu. S. Tyupkin. It is a classical solution to the equations of motion of SU(2) Yang–Mills theory in Euclidean space-time, meaning it describes a transition between two different topological vacua of the theory. It was originally hoped to open the path to solving the problem of confinement, especially since Polyakov had proven in 1987 that instantons are the cause of confinement in three-dimensional compact-QED. This hope was not realized, however.
In mathematical physics, inversion transformations are a natural extension of Poincaré transformations to include all conformal, one-to-one transformations on coordinate space-time. They are less studied in physics because, unlike the rotations and translations of Poincaré symmetry, an object cannot be physically transformed by the inversion symmetry. Some physical theories are invariant under this symmetry, in these cases it is what is known as a 'hidden symmetry'. Other hidden symmetries of physics include gauge symmetry and general covariance.
In mathematics, quaternionic analysis is the study of functions with quaternions as the domain and/or range. Such functions can be called functions of a quaternion variable just as functions of a real variable or a complex variable are called.

In physics, particularly in quantum field theory, the Weyl equation is a relativistic wave equation for describing massless spin-1/2 particles called Weyl fermions. The equation is named after Hermann Weyl. The Weyl fermions are one of the three possible types of elementary fermions, the other two being the Dirac and the Majorana fermions.
















