Visual calculus, invented by Mamikon Mnatsakanian (known as Mamikon), is an approach to solving a variety of integral calculus problems. [1] Many problems that would otherwise seem quite difficult yield to the method with hardly a line of calculation. Mamikon collaborated with Tom Apostol on the 2013 book New Horizons in Geometry describing the subject.
Mamikon devised his method in 1959 while an undergraduate, first applying it to a well-known geometry problem: find the area of a ring (annulus), given the length of a chord tangent to the inner circumference. Perhaps surprisingly, no additional information is needed; the solution does not depend on the ring's inner and outer dimensions.
The traditional approach involves algebra and application of the Pythagorean theorem. Mamikon's method, however, envisions an alternate construction of the ring: first the inner circle alone is drawn, then a constant-length tangent is made to travel along its circumference, "sweeping out" the ring as it goes.
Now if all the (constant-length) tangents used in constructing the ring are translated so that their points of tangency coincide, the result is a circular disk of known radius (and easily computed area). Indeed, since the inner circle's radius is irrelevant, one could just as well have started with a circle of radius zero (a point)—and sweeping out a ring around a circle of zero radius is indistinguishable from simply rotating a line segment about one of its endpoints and sweeping out a disk.
Mamikon's insight was to recognize the equivalence of the two constructions; and because they are equivalent, they yield equal areas. Moreover, the two starting curves need not be circular—a finding not easily proven by more traditional geometric methods. This yields Mamikon's theorem:
The area of a cycloid can be calculated by considering the area between it and an enclosing rectangle. These tangents can all be clustered to form a circle. If the circle generating the cycloid has radius r then this circle also has radius r and area πr2. The area of the rectangle is 2r × 2πr = 4πr2. Therefore, the area of the cycloid is 3πr2: it is 3 times the area of the generating circle.
The tangent cluster can be seen to be a circle because the cycloid is generated by a circle and the tangent to the cycloid will be at right angle to the line from the generating point to the rolling point. Thus the tangent and the line to the contact point form a right-angled triangle in the generating circle. This means that clustered together the tangents will describe the shape of the generating circle. [3]
Area is the measure of a region's size on a surface. The area of a plane region or plane area refers to the area of a shape or planar lamina, while surface area refers to the area of an open surface or the boundary of a three-dimensional object. Area can be understood as the amount of material with a given thickness that would be necessary to fashion a model of the shape, or the amount of paint necessary to cover the surface with a single coat. It is the two-dimensional analogue of the length of a curve or the volume of a solid . Two different regions may have the same area ; by synecdoche, "area" sometimes is used to refer to the region, as in a "polygonal area".
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. The distance between any point of the circle and the centre is called the radius.
A sphere is a geometrical object that is a three-dimensional analogue to a two-dimensional circle. Formally, a sphere is the set of points that are all at the same distance r from a given point in three-dimensional space. That given point is the center of the sphere, and r is the sphere's radius. The earliest known mentions of spheres appear in the work of the ancient Greek mathematicians.
In geometry, a cycloid is the curve traced by a point on a circle as it rolls along a straight line without slipping. A cycloid is a specific form of trochoid and is an example of a roulette, a curve generated by a curve rolling on another curve.
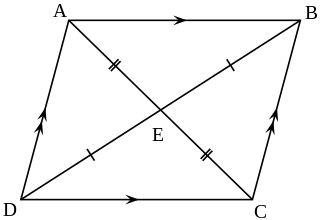
In Euclidean geometry, a parallelogram is a simple (non-self-intersecting) quadrilateral with two pairs of parallel sides. The opposite or facing sides of a parallelogram are of equal length and the opposite angles of a parallelogram are of equal measure. The congruence of opposite sides and opposite angles is a direct consequence of the Euclidean parallel postulate and neither condition can be proven without appealing to the Euclidean parallel postulate or one of its equivalent formulations.
In mathematics, hyperbolic geometry is a non-Euclidean geometry. The parallel postulate of Euclidean geometry is replaced with:
In mathematics, an annulus is the region between two concentric circles. Informally, it is shaped like a ring or a hardware washer. The word "annulus" is borrowed from the Latin word anulus or annulus meaning 'little ring'. The adjectival form is annular.
In mathematics, a semicircle is a one-dimensional locus of points that forms half of a circle. It is a circular arc that measures 180°. It has only one line of symmetry.
In geometry, an arbelos is a plane region bounded by three semicircles with three apexes such that each corner of each semicircle is shared with one of the others (connected), all on the same side of a straight line that contains their diameters.
In geometry, the area enclosed by a circle of radius r is πr2. Here the Greek letter π represents the constant ratio of the circumference of any circle to its diameter, approximately equal to 3.14159.
Tom Mike Apostol was an American analytic number theorist and professor at the California Institute of Technology, best known as the author of widely used mathematical textbooks.
In plane geometry, Holditch's theorem states that if a chord of fixed length is allowed to rotate inside a convex closed curve, then the locus of a point on the chord a distance p from one end and a distance q from the other is a closed curve whose enclosed area is less than that of the original curve by . The theorem was published in 1858 by Rev. Hamnet Holditch. While not mentioned by Holditch, the proof of the theorem requires an assumption that the chord be short enough that the traced locus is a simple closed curve.
The circle packing theorem describes the possible tangency relations between circles in the plane whose interiors are disjoint. A circle packing is a connected collection of circles whose interiors are disjoint. The intersection graph of a circle packing is the graph having a vertex for each circle, and an edge for every pair of circles that are tangent. If the circle packing is on the plane, or, equivalently, on the sphere, then its intersection graph is called a coin graph; more generally, intersection graphs of interior-disjoint geometric objects are called tangency graphs or contact graphs. Coin graphs are always connected, simple, and planar. The circle packing theorem states that these are the only requirements for a graph to be a coin graph:
A timeline of calculus and mathematical analysis.
In geometry, Cavalieri's principle, a modern implementation of the method of indivisibles, named after Bonaventura Cavalieri, is as follows:
Mamikon A. Mnatsakanian (1942–2021) was an Armenian physicist. In 1959, he discovered a new proof of the Pythagorean theorem.
In Euclidean geometry, a tangential polygon, also known as a circumscribed polygon, is a convex polygon that contains an inscribed circle. This is a circle that is tangent to each of the polygon's sides. The dual polygon of a tangential polygon is a cyclic polygon, which has a circumscribed circle passing through each of its vertices.
In geometry, a cyclogon is the curve traced by a vertex of a regular polygon that rolls without slipping along a straight line.
In mathematics and particularly in elementary geometry, a circumgon is a geometric figure which circumscribes some circle, in the sense that it is the union of the outer edges of non-overlapping triangles each of which has a vertex at the center of the circle and opposite side on a line that is tangent to the circle. The limiting case in which part or all of the circumgon is a circular arc is permitted. A circumgonal region is the union of those triangular regions.