In mathematics, a continuous function is a function such that a small variation of the argument induces a small variation of the value of the function. This implies there are no abrupt changes in value, known as discontinuities. More precisely, a function is continuous if arbitrarily small changes in its value can be assured by restricting to sufficiently small changes of its argument. A discontinuous function is a function that is not continuous. Until the 19th century, mathematicians largely relied on intuitive notions of continuity and considered only continuous functions. The epsilon–delta definition of a limit was introduced to formalize the definition of continuity.

In topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint non-empty open subsets. Connectedness is one of the principal topological properties that are used to distinguish topological spaces.
In mathematics, a topological space is, roughly speaking, a geometrical space in which closeness is defined but cannot necessarily be measured by a numeric distance. More specifically, a topological space is a set whose elements are called points, along with an additional structure called a topology, which can be defined as a set of neighbourhoods for each point that satisfy some axioms formalizing the concept of closeness. There are several equivalent definitions of a topology, the most commonly used of which is the definition through open sets, which is easier than the others to manipulate.
This is a glossary of some terms used in the branch of mathematics known as topology. Although there is no absolute distinction between different areas of topology, the focus here is on general topology. The following definitions are also fundamental to algebraic topology, differential topology and geometric topology.
In the mathematical field of topology, a uniform space is a topological space with additional structure that is used to define uniform properties, such as completeness, uniform continuity and uniform convergence. Uniform spaces generalize metric spaces and topological groups, but the concept is designed to formulate the weakest axioms needed for most proofs in analysis.

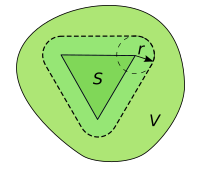
In mathematics, an open set is a generalization of an open interval in the real line.
In topology, the closure of a subset S of points in a topological space consists of all points in S together with all limit points of S. The closure of S may equivalently be defined as the union of S and its boundary, and also as the intersection of all closed sets containing S. Intuitively, the closure can be thought of as all the points that are either in S or "very near" S. A point which is in the closure of S is a point of closure of S. The notion of closure is in many ways dual to the notion of interior.

In mathematics, topological groups are the combination of groups and topological spaces, i.e. they are groups and topological spaces at the same time, such that the continuity condition for the group operations connects these two structures together and consequently they are not independent from each other.
In mathematics, a topological vector space is one of the basic structures investigated in functional analysis. A topological vector space is a vector space that is also a topological space with the property that the vector space operations are also continuous functions. Such a topology is called a vector topology and every topological vector space has a uniform topological structure, allowing a notion of uniform convergence and completeness. Some authors also require that the space is a Hausdorff space. One of the most widely studied categories of TVSs are locally convex topological vector spaces. This article focuses on TVSs that are not necessarily locally convex. Banach spaces, Hilbert spaces and Sobolev spaces are other well-known examples of TVSs.
In mathematics, a base (or basis; pl.: bases) for the topology τ of a topological space (X, τ) is a family of open subsets of X such that every open set of the topology is equal to the union of some sub-family of . For example, the set of all open intervals in the real number line is a basis for the Euclidean topology on because every open interval is an open set, and also every open subset of can be written as a union of some family of open intervals.

In topology and mathematics in general, the boundary of a subset S of a topological space X is the set of points in the closure of S not belonging to the interior of S. An element of the boundary of S is called a boundary point of S. The term boundary operation refers to finding or taking the boundary of a set. Notations used for boundary of a set S include and .
In topology and related branches of mathematics, separated sets are pairs of subsets of a given topological space that are related to each other in a certain way: roughly speaking, neither overlapping nor touching. The notion of when two sets are separated or not is important both to the notion of connected spaces as well as to the separation axioms for topological spaces.
In general topology, a branch of mathematics, a non-empty family A of subsets of a set is said to have the finite intersection property (FIP) if the intersection over any finite subcollection of is non-empty. It has the strong finite intersection property (SFIP) if the intersection over any finite subcollection of is infinite. Sets with the finite intersection property are also called centered systems and filter subbases.
In mathematics, more specifically in topology, an open map is a function between two topological spaces that maps open sets to open sets. That is, a function is open if for any open set in the image is open in Likewise, a closed map is a function that maps closed sets to closed sets. A map may be open, closed, both, or neither; in particular, an open map need not be closed and vice versa.
In mathematical analysis, a family of functions is equicontinuous if all the functions are continuous and they have equal variation over a given neighbourhood, in a precise sense described herein. In particular, the concept applies to countable families, and thus sequences of functions.
In topology and related areas of mathematics, the neighbourhood system, complete system of neighbourhoods, or neighbourhood filter for a point in a topological space is the collection of all neighbourhoods of

In topology and other branches of mathematics, a topological space X is locally connected if every point admits a neighbourhood basis consisting of open connected sets.
In mathematics, an adherent point of a subset of a topological space is a point in such that every neighbourhood of contains at least one point of A point is an adherent point for if and only if is in the closure of thus
In topology and related areas of mathematics, a subset A of a topological space X is said to be dense in X if every point of X either belongs to A or else is arbitrarily "close" to a member of A — for instance, the rational numbers are a dense subset of the real numbers because every real number either is a rational number or has a rational number arbitrarily close to it. Formally, is dense in if the smallest closed subset of containing is itself.
In functional analysis and related areas of mathematics, a complete topological vector space is a topological vector space (TVS) with the property that whenever points get progressively closer to each other, then there exists some point towards which they all get closer. The notion of "points that get progressively closer" is made rigorous by Cauchy nets or Cauchy filters, which are generalizations of Cauchy sequences, while "point towards which they all get closer" means that this Cauchy net or filter converges to The notion of completeness for TVSs uses the theory of uniform spaces as a framework to generalize the notion of completeness for metric spaces. But unlike metric-completeness, TVS-completeness does not depend on any metric and is defined for all TVSs, including those that are not metrizable or Hausdorff.