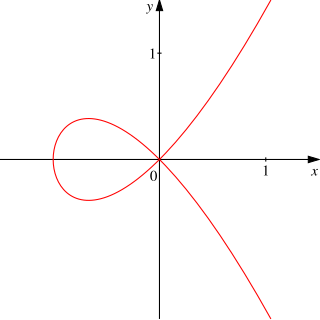Motivation
For example, suppose C is a plane curve defined by a polynomial equation
- F(X,Y) = 0
and take P to be the origin (0,0). Erasing terms of higher order than 1 would produce a 'linearised' equation reading
- L(X,Y) = 0
in which all terms XaYb have been discarded if a + b > 1.
We have two cases: L may be 0, or it may be the equation of a line. In the first case the (Zariski) tangent space to C at (0,0) is the whole plane, considered as a two-dimensional affine space. In the second case, the tangent space is that line, considered as affine space. (The question of the origin comes up, when we take P as a general point on C; it is better to say 'affine space' and then note that P is a natural origin, rather than insist directly that it is a vector space.)
It is easy to see that over the real field we can obtain L in terms of the first partial derivatives of F. When those both are 0 at P, we have a singular point (double point, cusp or something more complicated). The general definition is that singular points of C are the cases when the tangent space has dimension 2.
Definition
The cotangent space of a local ring R, with maximal ideal  is defined to be
is defined to be

where  2 is given by the product of ideals. It is a vector space over the residue field k:= R/
2 is given by the product of ideals. It is a vector space over the residue field k:= R/ . Its dual (as a k-vector space) is called tangent space of R.
. Its dual (as a k-vector space) is called tangent space of R.
This definition is a generalization of the above example to higher dimensions: suppose given an affine algebraic variety V and a point v of V. Morally, modding out  2 corresponds to dropping the non-linear terms from the equations defining V inside some affine space, therefore giving a system of linear equations that define the tangent space.
2 corresponds to dropping the non-linear terms from the equations defining V inside some affine space, therefore giving a system of linear equations that define the tangent space.
The tangent space  and cotangent space
and cotangent space  to a scheme X at a point P is the (co)tangent space of
to a scheme X at a point P is the (co)tangent space of  . Due to the functoriality of Spec, the natural quotient map
. Due to the functoriality of Spec, the natural quotient map  induces a homomorphism
induces a homomorphism  for X=Spec(R), P a point in Y=Spec(R/I). This is used to embed
for X=Spec(R), P a point in Y=Spec(R/I). This is used to embed  in
in  . [2] Since morphisms of fields are injective, the surjection of the residue fields induced by g is an isomorphism. Then a morphism k of the cotangent spaces is induced by g, given by
. [2] Since morphisms of fields are injective, the surjection of the residue fields induced by g is an isomorphism. Then a morphism k of the cotangent spaces is induced by g, given by




Since this is a surjection, the transpose  is an injection.
is an injection.
(One often defines the tangent and cotangent spaces for a manifold in the analogous manner.)
Properties
If R is a Noetherian local ring, the dimension of the tangent space is at least the dimension of R:
- dim m/m2 ≧ dim R
R is called regular if equality holds. In a more geometric parlance, when R is the local ring of a variety V at a point v, one also says that v is a regular point. Otherwise it is called a singular point.
The tangent space has an interpretation in terms of K[t]/(t2), the dual numbers for K; in the parlance of schemes, morphisms from SpecK[t]/(t2) to a scheme X over K correspond to a choice of a rational point x ∈ X(k) and an element of the tangent space at x. Therefore, one also talks about tangent vectors. See also: tangent space to a functor.
In general, the dimension of the Zariski tangent space can be extremely large. For example, let  be the ring of continuously differentiable real-valued functions on
be the ring of continuously differentiable real-valued functions on  . Define
. Define  to be the ring of germs of such functions at the origin. Then R is a local ring, and its maximal ideal m consists of all germs which vanish at the origin. The functions
to be the ring of germs of such functions at the origin. Then R is a local ring, and its maximal ideal m consists of all germs which vanish at the origin. The functions  for
for  define linearly independent vectors in the Zariski cotangent space
define linearly independent vectors in the Zariski cotangent space  , so the dimension of
, so the dimension of  is at least the
is at least the  , the cardinality of the continuum. The dimension of the Zariski tangent space
, the cardinality of the continuum. The dimension of the Zariski tangent space  is therefore at least
is therefore at least  . On the other hand, the ring of germs of smooth functions at a point in an n-manifold has an n-dimensional Zariski cotangent space. [lower-alpha 1]
. On the other hand, the ring of germs of smooth functions at a point in an n-manifold has an n-dimensional Zariski cotangent space. [lower-alpha 1]
In mathematics, the tangent space of a manifold is a generalization of tangent lines to curves in two-dimensional space and tangent planes to surfaces in three-dimensional space in higher dimensions. In the context of physics the tangent space to a manifold at a point can be viewed as the space of possible velocities for a particle moving on the manifold.
In commutative algebra, the prime spectrum of a commutative ring R is the set of all prime ideals of R, and is usually denoted by ; in algebraic geometry it is simultaneously a topological space equipped with the sheaf of rings .
In commutative algebra, the Krull dimension of a commutative ring R, named after Wolfgang Krull, is the supremum of the lengths of all chains of prime ideals. The Krull dimension need not be finite even for a Noetherian ring. More generally the Krull dimension can be defined for modules over possibly non-commutative rings as the deviation of the poset of submodules.

Algebraic varieties are the central objects of study in algebraic geometry, a sub-field of mathematics. Classically, an algebraic variety is defined as the set of solutions of a system of polynomial equations over the real or complex numbers. Modern definitions generalize this concept in several different ways, while attempting to preserve the geometric intuition behind the original definition.
In mathematics and specifically in algebraic geometry, the dimension of an algebraic variety may be defined in various equivalent ways.
In mathematics and physics, a tensor field assigns a tensor to each point of a mathematical space. Tensor fields are used in differential geometry, algebraic geometry, general relativity, in the analysis of stress and strain in materials, and in numerous applications in the physical sciences. As a tensor is a generalization of a scalar and a vector, a tensor field is a generalization of a scalar field or vector field that assigns, respectively, a scalar or vector to each point of space. If a tensor A is defined on a vector fields set X(M) over a module M, we call A a tensor field on M.

In mathematics, an affine space is a geometric structure that generalizes some of the properties of Euclidean spaces in such a way that these are independent of the concepts of distance and measure of angles, keeping only the properties related to parallelism and ratio of lengths for parallel line segments. Affine space is the setting for affine geometry.
In algebraic geometry, an affine algebraic set is the set of the common zeros over an algebraically closed field k of some family of polynomials in the polynomial ring An affine variety or affine algebraic variety, is an affine algebraic set such that the ideal generated by the defining polynomials is prime.
In mathematics, a scheme is a mathematical structure that enlarges the notion of algebraic variety in several ways, such as taking account of multiplicities and allowing "varieties" defined over any commutative ring.
In commutative algebra, a regular local ring is a Noetherian local ring having the property that the minimal number of generators of its maximal ideal is equal to its Krull dimension. In symbols, let A be a Noetherian local ring with maximal ideal m, and suppose a1, ..., an is a minimal set of generators of m. Then by Krull's principal ideal theorem n ≥ dim A, and A is defined to be regular if n = dim A.
In mathematics, deformation theory is the study of infinitesimal conditions associated with varying a solution P of a problem to slightly different solutions Pε, where ε is a small number, or a vector of small quantities. The infinitesimal conditions are the result of applying the approach of differential calculus to solving a problem with constraints. The name is an analogy to non-rigid structures that deform slightly to accommodate external forces.
In mathematics, a Killing vector field, named after Wilhelm Killing, is a vector field on a Riemannian manifold that preserves the metric. Killing fields are the infinitesimal generators of isometries; that is, flows generated by Killing fields are continuous isometries of the manifold. More simply, the flow generates a symmetry, in the sense that moving each point of an object the same distance in the direction of the Killing vector will not distort distances on the object.
In geometry, the tangent cone is a generalization of the notion of the tangent space to a manifold to the case of certain spaces with singularities.

In mathematics, a differentiable manifold is a type of manifold that is locally similar enough to a vector space to allow one to apply calculus. Any manifold can be described by a collection of charts (atlas). One may then apply ideas from calculus while working within the individual charts, since each chart lies within a vector space to which the usual rules of calculus apply. If the charts are suitably compatible, then computations done in one chart are valid in any other differentiable chart.
In mathematics, a D-module is a module over a ring D of differential operators. The major interest of such D-modules is as an approach to the theory of linear partial differential equations. Since around 1970, D-module theory has been built up, mainly as a response to the ideas of Mikio Sato on algebraic analysis, and expanding on the work of Sato and Joseph Bernstein on the Bernstein–Sato polynomial.
Affine geometry, broadly speaking, is the study of the geometrical properties of lines, planes, and their higher dimensional analogs, in which a notion of "parallel" is retained, but no metrical notions of distance or angle are. Affine spaces differ from linear spaces in that they do not have a distinguished choice of origin. So, in the words of Marcel Berger, "An affine space is nothing more than a vector space whose origin we try to forget about, by adding translations to the linear maps." Accordingly, a complex affine space, that is an affine space over the complex numbers, is like a complex vector space, but without a distinguished point to serve as the origin.
In algebraic geometry, a smooth scheme over a field is a scheme which is well approximated by affine space near any point. Smoothness is one way of making precise the notion of a scheme with no singular points. A special case is the notion of a smooth variety over a field. Smooth schemes play the role in algebraic geometry of manifolds in topology.
This is a glossary of algebraic geometry.
In algebraic geometry, a derived scheme is a homotopy-theoretic generalization of a scheme in which classical commutative rings are replaced with derived versions such as cdgas, commutative simplicial rings, or commutative ring spectra.
In algebraic geometry, the main theorem of elimination theory states that every projective scheme is proper. A version of this theorem predates the existence of scheme theory. It can be stated, proved, and applied in the following more classical setting. Let k be a field, denote by the n-dimensional projective space over k. The main theorem of elimination theory is the statement that for any n and any algebraic variety V defined over k, the projection map sends Zariski-closed subsets to Zariski-closed subsets.