 | This article needs additional citations for verification .(July 2022) |
Different cultures used different traditional numeral systems for naming large numbers. The extent of large numbers used varied in each culture. Two interesting points in using large numbers are the confusion on the term billion and milliard in many countries, and the use of zillion to denote a very large number where precision is not required.
The Shukla Yajurveda has a list of names for powers of ten up to 1012. The list given in the Yajurveda text is:
Later Hindu and Buddhist texts have extended this list, but these lists are no longer mutually consistent and names of numbers larger than 108 differ between texts.
For example, the Panchavimsha Brahmana lists 109 as nikharva, 1010vâdava, 1011akṣiti, while Śâṅkhyâyana Śrauta Sûtra has 109nikharva, 1010samudra, 1011salila, 1012antya, 1013ananta. Such lists of names for powers of ten are called daśaguṇottarra saṁjñâ. There area also analogous lists of Sanskrit names for fractional numbers, that is, powers of one tenth.
The Mahayana Lalitavistara Sutra is notable for giving a very extensive such list, with terms going up to 10421. The context is an account of a contest including writing, arithmetic, wrestling and archery, in which the Buddha was pitted against the great mathematician Arjuna and showed off his numerical skills by citing the names of the powers of ten up to 1 'tallakshana', which equals 1053, but then going on to explain that this is just one of a series of counting systems that can be expanded geometrically.
The Avataṃsaka Sūtra, a text associated with the Lokottaravāda school of Buddhism, has an even more extensive list of names for numbers, and it goes beyond listing mere powers of ten introducing concatenation of exponentiation, the largest number mentioned being nirabhilapya nirabhilapya parivarta (Bukeshuo bukeshuo zhuan 不可說不可說轉), corresponding to . [2] [3] though chapter 30 (the Asamkyeyas) in Thomas Cleary's translation of it we find the definition of the number "untold" as exactly 1010*2122, expanded in the 2nd verses to 104*5*2121 and continuing a similar expansion indeterminately. Examples for other names given in the Avatamsaka Sutra include: asaṃkhyeya (असंख्येय) 10140.
The Jain mathematical text Surya Prajnapti (c. 4th–3rd century BCE) classifies all numbers into three sets: enumerable, innumerable, and infinite. Each of these was further subdivided into three orders: [4] enumerable (lowest, intermediate, and highest), innumerable (nearly innumerable, truly innumerable, and innumerably innumerable), and infinite (nearly infinite, truly infinite, infinitely infinite).
In modern India, the terms lakh for 105 and crore for 107 are in common use. Both are vernacular (Hindustani) forms derived from a list of names for powers of ten in Yājñavalkya Smṛti, where 105 and 107 named lakṣa and koṭi, respectively.
In the Western world, specific number names for larger numbers did not come into common use until quite recently. The Ancient Greeks used a system based on the myriad, that is, ten thousand, and their largest named number was a myriad myriad, or one hundred million.
In The Sand Reckoner , Archimedes (c. 287–212 BC) devised a system of naming large numbers reaching up to
essentially by naming powers of a myriad myriad. This largest number appears because it equals a myriad myriad to the myriad myriadth power, all taken to the myriad myriadth power. This gives a good indication of the notational difficulties encountered by Archimedes, and one can propose that he stopped at this number because he did not devise any new ordinal numbers (larger than 'myriad myriadth') to match his new cardinal numbers. Archimedes only used his system up to 1064.
Archimedes' goal was presumably to name large powers of 10 in order to give rough estimates, but shortly thereafter, Apollonius of Perga invented a more practical system of naming large numbers which were not powers of 10, based on naming powers of a myriad, for example, would be a myriad squared.
Much later, but still in antiquity, the Hellenistic mathematician Diophantus (3rd century) used a similar notation to represent large numbers.
The Romans, who were less interested in theoretical issues, expressed 1,000,000 as decies centena milia, that is, 'ten hundred thousand'; it was only in the 13th century that the (originally French) word 'million' was introduced .
Far larger finite numbers than any of these occur in modern mathematics. For instance, Graham's number is too large to reasonably express using exponentiation or even tetration. For more about modern usage for large numbers, see Large numbers. To handle these numbers, new notations are created and used. There is a large community of mathematicians dedicated to naming large numbers. Rayo's number has been claimed to be the largest named number. [5]
The ultimate in large numbers was, until recently, the concept of infinity, a number defined by being greater than any finite number, and used in the mathematical theory of limits.
However, since the 19th century, mathematicians have studied transfinite numbers, numbers which are not only greater than any finite number, but also, from the viewpoint of set theory, larger than the traditional concept of infinity. Of these transfinite numbers, perhaps the most extraordinary, and arguably, if they exist, "largest", are the large cardinals.
Arithmetic is an elementary part of mathematics that consists of the study of the properties of the traditional operations on numbers—addition, subtraction, multiplication, division, exponentiation, and extraction of roots. In the 19th century, Italian mathematician Giuseppe Peano formalized arithmetic with his Peano axioms, which are highly important to the field of mathematical logic today.
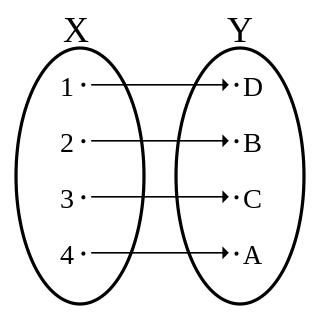
In mathematics, a cardinal number, or cardinal for short, is what is commonly called the number of elements of a set. In the case of a finite set, its cardinal number, or cardinality is therefore a natural number. For dealing with the case of infinite sets, the infinite cardinal numbers have been introduced, which are often denoted with the Hebrew letter (aleph) marked with subscript indicating their rank among the infinite cardinals.
The decimal numeral system is the standard system for denoting integer and non-integer numbers. It is the extension to non-integer numbers of the Hindu–Arabic numeral system. The way of denoting numbers in the decimal system is often referred to as decimal notation.
A number is a mathematical object used to count, measure, and label. The original examples are the natural numbers 1, 2, 3, 4, and so forth. Numbers can be represented in language with number words. More universally, individual numbers can be represented by symbols, called numerals; for example, "5" is a numeral that represents the number five. As only a relatively small number of symbols can be memorized, basic numerals are commonly organized in a numeral system, which is an organized way to represent any number. The most common numeral system is the Hindu–Arabic numeral system, which allows for the representation of any number using a combination of ten fundamental numeric symbols, called digits. In addition to their use in counting and measuring, numerals are often used for labels, for ordering, and for codes. In common usage, a numeral is not clearly distinguished from the number that it represents.
Hilbert's paradox of the Grand Hotel is a thought experiment which illustrates a counterintuitive property of infinite sets. It is demonstrated that a fully occupied hotel with infinitely many rooms may still accommodate additional guests, even infinitely many of them, and this process may be repeated infinitely often. The idea was introduced by David Hilbert in a 1924 lecture "Über das Unendliche", reprinted in, and was popularized through George Gamow's 1947 book One Two Three... Infinity.
In the philosophy of mathematics, the abstraction of actual infinity involves the acceptance of infinite entities as given, actual and completed objects. These might include the set of natural numbers, extended real numbers, transfinite numbers, or even an infinite sequence of rational numbers. Actual infinity is to be contrasted with potential infinity, in which a non-terminating process produces a sequence with no last element, and where each individual result is finite and is achieved in a finite number of steps. As a result, potential infinity is often formalized using the concept of a limit.
Finitism is a philosophy of mathematics that accepts the existence only of finite mathematical objects. It is best understood in comparison to the mainstream philosophy of mathematics where infinite mathematical objects are accepted as legitimate.
In mathematics, an infinitesimal number is a quantity that is closer to 0 than any standard real number, but that is not 0. The word infinitesimal comes from a 17th-century Modern Latin coinage infinitesimus, which originally referred to the "infinity-th" item in a sequence.
In mathematics, transfinite numbers or infinite numbers are numbers that are "infinite" in the sense that they are larger than all finite numbers. These include the transfinite cardinals, which are cardinal numbers used to quantify the size of infinite sets, and the transfinite ordinals, which are ordinal numbers used to provide an ordering of infinite sets. The term transfinite was coined in 1895 by Georg Cantor, who wished to avoid some of the implications of the word infinite in connection with these objects, which were, nevertheless, not finite. Few contemporary writers share these qualms; it is now accepted usage to refer to transfinite cardinals and ordinals as infinite numbers. Nevertheless, the term transfinite also remains in use.
The Sand Reckoner is a work by Archimedes, an Ancient Greek mathematician of the 3rd century BC, in which he set out to determine an upper bound for the number of grains of sand that fit into the universe. In order to do this, Archimedes had to estimate the size of the universe according to the contemporary model, and invent a way to talk about extremely large numbers.
The Buddhāvataṃsaka-nāma-mahāvaipulya-sūtra is one of the most influential Mahāyāna sutras of East Asian Buddhism. It is often referred to in short as the Avataṃsaka Sūtra. In Classical Sanskrit, avataṃsaka means garland, wreath, or any circular ornament, such as an earring. Thus, the title may be rendered in English as A Garland of Buddhas, Buddha Ornaments, or Buddha’s Garland. In Buddhist Hybrid Sanskrit, the term avataṃsaka means “a great number,” “a multitude,” or “a collection.” This is matched by the Tibetan title of the sutra, which is A Multitude of Buddhas.
In mathematics, particularly in set theory, the aleph numbers are a sequence of numbers used to represent the cardinality of infinite sets that can be well-ordered. They were introduced by the mathematician Georg Cantor and are named after the symbol he used to denote them, the Hebrew letter aleph.
-yllion is a proposal from Donald Knuth for the terminology and symbols of an alternate decimal superbase system. In it, he adapts the familiar English terms for large numbers to provide a systematic set of names for much larger numbers. In addition to providing an extended range, -yllion also dodges the long and short scale ambiguity of -illion.
Two naming scales for large numbers have been used in English and other European languages since the early modern era: the long and short scales. Most English variants use the short scale today, but the long scale remains dominant in many non-English-speaking areas, including continental Europe and Spanish-speaking countries in Latin America. These naming procedures are based on taking the number n occurring in 103n+3 or 106n and concatenating Latin roots for its units, tens, and hundreds place, together with the suffix -illion.
A power of 10 is any of the integer powers of the number ten; in other words, ten multiplied by itself a certain number of times. By definition, the number one is a power of ten. The first few non-negative powers of ten are:
In the mathematical field of set theory, ordinal arithmetic describes the three usual operations on ordinal numbers: addition, multiplication, and exponentiation. Each can be defined in essentially two different ways: either by constructing an explicit well-ordered set that represents the result of the operation or by using transfinite recursion. Cantor normal form provides a standardized way of writing ordinals. In addition to these usual ordinal operations, there are also the "natural" arithmetic of ordinals and the nimber operations.
In philosophy and theology, infinity is explored in articles under headings such as the Absolute, God, and Zeno's paradoxes.
A timeline of numerals and arithmetic.
This is a timeline of pure and applied mathematics history. It is divided here into three stages, corresponding to stages in the development of mathematical notation: a "rhetorical" stage in which calculations are described purely by words, a "syncopated" stage in which quantities and common algebraic operations are beginning to be represented by symbolic abbreviations, and finally a "symbolic" stage, in which comprehensive notational systems for formulas are the norm.
Infinity is something which is boundless, endless, or larger than any natural number. It is often denoted by the infinity symbol .
