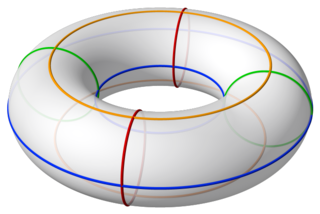
In geometry, a torus is a surface of revolution generated by revolving a circle in three-dimensional space one full revolution about an axis that is coplanar with the circle. The main types of toruses include ring toruses, horn toruses, and spindle toruses. A ring torus is sometimes colloquially referred to as a donut or doughnut.
In mathematics, homology is a general way of associating a sequence of algebraic objects, such as abelian groups or modules, with other mathematical objects such as topological spaces. Homology groups were originally defined in algebraic topology. Similar constructions are available in a wide variety of other contexts, such as abstract algebra, groups, Lie algebras, Galois theory, and algebraic geometry.
In mathematics, a diffeology on a set generalizes the concept of smooth charts in a differentiable manifold, declaring what the "smooth parametrizations" in the set are.

In mathematics, de Rham cohomology is a tool belonging both to algebraic topology and to differential topology, capable of expressing basic topological information about smooth manifolds in a form particularly adapted to computation and the concrete representation of cohomology classes. It is a cohomology theory based on the existence of differential forms with prescribed properties.
In mathematics, homotopy groups are used in algebraic topology to classify topological spaces. The first and simplest homotopy group is the fundamental group, denoted which records information about loops in a space. Intuitively, homotopy groups record information about the basic shape, or holes, of a topological space.
In mathematics, a closed manifold is a manifold without boundary that is compact. In comparison, an open manifold is a manifold without boundary that has only non-compact components.
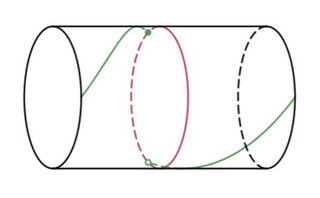
In geometric topology, a branch of mathematics, a Dehn twist is a certain type of self-homeomorphism of a surface.
In mathematics, in the subfield of geometric topology, the mapping class group is an important algebraic invariant of a topological space. Briefly, the mapping class group is a certain discrete group corresponding to symmetries of the space.

In mathematics, a 3-manifold is a topological space that locally looks like a three-dimensional Euclidean space. A 3-manifold can be thought of as a possible shape of the universe. Just as a sphere looks like a plane to a small and close enough observer, all 3-manifolds look like our universe does to a small enough observer. This is made more precise in the definition below.

In mathematics, the Whitehead manifold is an open 3-manifold that is contractible, but not homeomorphic to J. H. C. Whitehead discovered this puzzling object while he was trying to prove the Poincaré conjecture, correcting an error in an earlier paper Whitehead where he incorrectly claimed that no such manifold exists.

In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, an -dimensional manifold, or -manifold for short, is a topological space with the property that each point has a neighborhood that is homeomorphic to an open subset of -dimensional Euclidean space.

In mathematics, a solenoid is a compact connected topological space that may be obtained as the inverse limit of an inverse system of topological groups and continuous homomorphisms

The three-dimensional torus, or 3-torus, is defined as any topological space that is homeomorphic to the Cartesian product of three circles, In contrast, the usual torus is the Cartesian product of only two circles.
A Picard horn, also called the Picard topology or Picard model, is one of the oldest known hyperbolic 3-manifolds, first described by Émile Picard in 1884. The manifold is the quotient of the upper half-plane model of hyperbolic 3-space by the projective special linear group, . It was proposed as a model for the shape of the universe in 2004. The term "horn" is due to pseudosphere models of hyperbolic space.
In mathematics, a Riemannian manifold is said to be flat if its Riemann curvature tensor is everywhere zero. Intuitively, a flat manifold is one that "locally looks like" Euclidean space in terms of distances and angles, e.g. the interior angles of a triangle add up to 180°.

Spacetime topology is the topological structure of spacetime, a topic studied primarily in general relativity. This physical theory models gravitation as the curvature of a four dimensional Lorentzian manifold and the concepts of topology thus become important in analysing local as well as global aspects of spacetime. The study of spacetime topology is especially important in physical cosmology.

In mathematics, especially in the area of mathematical analysis known as dynamical systems theory, a linear flow on the torus is a flow on the n-dimensional torus
In mathematics, a quasitoric manifold is a topological analogue of the nonsingular projective toric variety of algebraic geometry. A smooth -dimensional manifold is a quasitoric manifold if it admits a smooth, locally standard action of an -dimensional torus, with orbit space an -dimensional simple convex polytope.
The Hantzsche–Wendt manifold, also known as the HW manifold or didicosm, is a compact, orientable, flat 3-manifold, first studied by Walter Hantzsche and Hilmar Wendt in 1934. It is the only closed flat 3-manifold with first Betti number zero. Its holonomy group is . It has been suggested as a possible shape of the universe because its complicated geometry can obscure the features in the cosmic microwave background that would arise if the universe is a closed flat manifold, such as the 3-torus.












